5 Ways To Convert Quadratic Equations To Vertex Form

Quick Links :

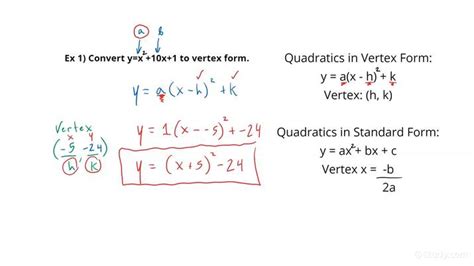
Converting quadratic equations to vertex form is an essential skill in algebra, as it allows us to easily identify the vertex of a parabola and understand its behavior. In this article, we'll explore five ways to convert quadratic equations to vertex form, including using the completing the square method, the vertex form formula, and more.

Why Convert Quadratic Equations to Vertex Form?

Before we dive into the methods, let's quickly discuss why converting quadratic equations to vertex form is important. Vertex form, also known as the "vertex representation," provides a concise way to represent quadratic equations in the form y = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. This form is particularly useful for identifying the vertex, axis of symmetry, and direction of opening of the parabola.

Method 1: Completing the Square

The completing the square method is a popular technique for converting quadratic equations to vertex form. This method involves manipulating the equation to create a perfect square trinomial, which can then be written in vertex form.

To complete the square, follow these steps:

• Write the quadratic equation in standard form: ax^2 + bx + c = 0
• Move the constant term to the right side: ax^2 + bx = -c
• Divide both sides by a: x^2 + (b/a)x = -c/a
• Add (b/2a)^2 to both sides: x^2 + (b/a)x + (b/2a)^2 = -c/a + (b/2a)^2
• Factor the left side: (x + b/2a)^2 = -c/a + (b/2a)^2

The resulting equation is in vertex form, with the vertex at (-b/2a, -c/a + (b/2a)^2).

Method 2: Using the Vertex Form Formula

The vertex form formula provides a quick and easy way to convert quadratic equations to vertex form. The formula is:

y = a(x - h)^2 + k

where (h, k) is the vertex of the parabola. To use this formula, simply identify the values of a, h, and k from the given quadratic equation.

For example, given the quadratic equation x^2 + 4x + 4 = 0, we can identify the values of a, h, and k as follows:

• a = 1
• h = -4/2(1) = -2
• k = -4 + 4/2(1)^2 = -4 + 2 = -2

Substituting these values into the vertex form formula, we get:

y = (x + 2)^2 - 2

Method 3: Using the Quadratic Formula

The quadratic formula can also be used to convert quadratic equations to vertex form. The quadratic formula is:

x = (-b ± √(b^2 - 4ac)) / 2a

To use the quadratic formula to convert to vertex form, follow these steps:

• Write the quadratic equation in standard form: ax^2 + bx + c = 0
• Use the quadratic formula to find the roots of the equation
• Write the equation in factored form: a(x - r_1)(x - r_2) = 0
• Expand the factored form to get the equation in vertex form

For example, given the quadratic equation x^2 + 5x + 6 = 0, we can use the quadratic formula to find the roots:

x = (-5 ± √(5^2 - 4(1)(6))) / 2(1) x = (-5 ± √(25 - 24)) / 2 x = (-5 ± √1) / 2 x = (-5 ± 1) / 2 x = -2 or x = -3

Writing the equation in factored form, we get:

(x + 2)(x + 3) = 0

Expanding the factored form, we get:

x^2 + 5x + 6 = (x + 2)^2 + 2

Method 4: Using Graphing Calculators

Graphing calculators can also be used to convert quadratic equations to vertex form. Most graphing calculators have a built-in function for finding the vertex of a parabola.

To use a graphing calculator to convert to vertex form, follow these steps:

• Enter the quadratic equation into the calculator
• Use the calculator's built-in function to find the vertex of the parabola
• Write the equation in vertex form using the values of a, h, and k provided by the calculator

For example, given the quadratic equation x^2 + 4x + 4 = 0, we can use a graphing calculator to find the vertex:

• Enter the equation into the calculator: y = x^2 + 4x + 4
• Use the calculator's built-in function to find the vertex: Vertex: (-2, -2)
• Write the equation in vertex form: y = (x + 2)^2 - 2

Method 5: Using Algebraic Manipulation

Algebraic manipulation can also be used to convert quadratic equations to vertex form. This method involves manipulating the equation to create a perfect square trinomial, which can then be written in vertex form.

To use algebraic manipulation to convert to vertex form, follow these steps:

• Write the quadratic equation in standard form: ax^2 + bx + c = 0
• Factor out the leading coefficient: a(x^2 + (b/a)x + c/a) = 0
• Complete the square: a(x + b/2a)^2 = -c/a + (b/2a)^2
• Write the equation in vertex form: y = a(x - h)^2 + k

For example, given the quadratic equation x^2 + 6x + 9 = 0, we can use algebraic manipulation to convert to vertex form:

• Factor out the leading coefficient: 1(x^2 + 6x + 9) = 0
• Complete the square: (x + 3)^2 = -9 + 9
• Write the equation in vertex form: y = (x + 3)^2

Conclusion

Converting quadratic equations to vertex form is an essential skill in algebra, and there are several methods to do so. In this article, we explored five ways to convert quadratic equations to vertex form, including using the completing the square method, the vertex form formula, the quadratic formula, graphing calculators, and algebraic manipulation. By mastering these methods, you'll be able to easily identify the vertex of a parabola and understand its behavior.