Polynomial To Standard Form Made Easy

Quick Links :

Converting polynomials to standard form is a crucial concept in algebra, and it's essential to understand the process to simplify and manipulate polynomial expressions. In this article, we'll break down the steps to convert polynomials to standard form, making it easy for you to grasp and apply this concept in your math journey.

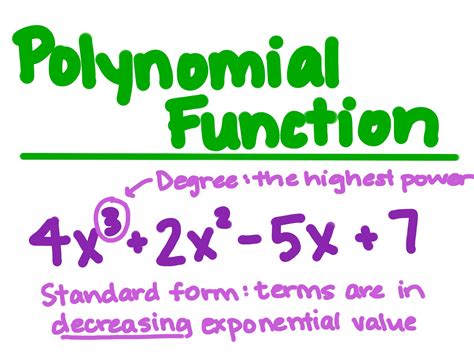
A polynomial is an expression consisting of variables and coefficients combined using addition, subtraction, and multiplication. Standard form, also known as the canonical form, is a way of writing polynomials in a specific order, making it easier to compare and manipulate them. Converting polynomials to standard form is a fundamental skill that will help you solve equations, graph functions, and model real-world problems.

What is Standard Form?

Standard form is a way of writing polynomials in a specific order, where the terms are arranged in descending order of their exponents. For example, the polynomial 3x^2 + 2x - 4 is in standard form, while 2x - 4 + 3x^2 is not. The standard form of a polynomial makes it easier to identify the degree of the polynomial, which is the highest exponent of the variable.

BENEFITS OF STANDARD FORM

Writing polynomials in standard form has several benefits, including:

• Easier comparison: Standard form makes it easy to compare two polynomials and determine if they are equal.
• Simplified addition and subtraction: When polynomials are in standard form, it's easier to add and subtract them.
• Easier factoring: Standard form makes it easier to factor polynomials, which is essential for solving equations.
• Better visualization: Standard form helps to visualize the graph of a polynomial function.

How to Convert Polynomials to Standard Form

Converting polynomials to standard form is a straightforward process that involves arranging the terms in descending order of their exponents. Here are the steps:

1. Write the polynomial expression: Start by writing the polynomial expression in its original form.
2. Identify the terms: Identify the individual terms in the polynomial expression.
3. Arrange the terms: Arrange the terms in descending order of their exponents. If there are multiple terms with the same exponent, arrange them in alphabetical order.
4. Combine like terms: Combine any like terms, which are terms with the same variable and exponent.

EXAMPLES OF POLYNOMIAL CONVERSION

Let's consider a few examples to illustrate the conversion process:

Example 1: Convert the polynomial 2x - 4 + 3x^2 to standard form.

Solution: Identify the terms, arrange them in descending order of their exponents, and combine like terms:

3x^2 + 2x - 4

Example 2: Convert the polynomial x^3 - 2x^2 + 3x - 1 to standard form.

Solution: Identify the terms, arrange them in descending order of their exponents, and combine like terms:

x^3 - 2x^2 + 3x - 1 (already in standard form)

Tips and Tricks for Converting Polynomials

Here are some tips and tricks to help you convert polynomials to standard form:

• Always write the polynomial expression in its original form before attempting to convert it.
• Identify the terms carefully, making sure to include all the variables and coefficients.
• Arrange the terms in descending order of their exponents, paying attention to multiple terms with the same exponent.
• Combine like terms carefully, making sure to combine only terms with the same variable and exponent.

COMMON MISTAKES TO AVOID

When converting polynomials to standard form, avoid the following common mistakes:

• Failing to identify all the terms in the polynomial expression.
• Arranging the terms in ascending order of their exponents instead of descending order.
• Combining unlike terms, which can lead to incorrect results.

We hope this article has helped you understand the process of converting polynomials to standard form. Remember to always follow the steps carefully, and don't hesitate to ask for help if you need it. Practice converting polynomials to standard form, and you'll become a pro in no time!