Complex numbers are a fundamental concept in mathematics, engineering, and physics, and understanding their polar form is crucial for many applications. A Polar Form of Complex Numbers Calculator is a valuable tool for converting complex numbers into their polar form, which can simplify many mathematical operations. In this article, we will explore five ways to use a Polar Form of Complex Numbers Calculator, including converting complex numbers to polar form, finding the magnitude and angle of complex numbers, and performing arithmetic operations.

What is Polar Form of Complex Numbers Calculator?
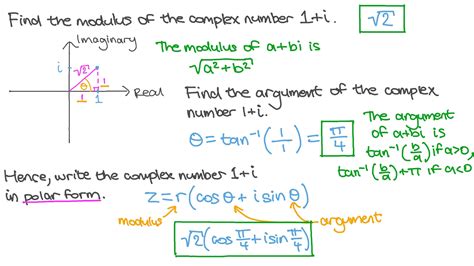
A Polar Form of Complex Numbers Calculator is an online tool that converts complex numbers into their polar form. Complex numbers are represented in the form a + bi, where a and b are real numbers and i is the imaginary unit. The polar form of a complex number is represented as r(cosθ + isinθ), where r is the magnitude (or length) of the complex number, and θ is the angle (or argument) of the complex number.
How to Use Polar Form of Complex Numbers Calculator
Using a Polar Form of Complex Numbers Calculator is straightforward. Simply enter the complex number in the form a + bi, and the calculator will convert it into its polar form. Here are five ways to use a Polar Form of Complex Numbers Calculator:
1. Converting Complex Numbers to Polar Form
One of the primary uses of a Polar Form of Complex Numbers Calculator is to convert complex numbers into their polar form. This can be useful for simplifying complex arithmetic operations, such as multiplication and division.
For example, let's say we want to convert the complex number 3 + 4i into its polar form. Using a Polar Form of Complex Numbers Calculator, we can enter the complex number and get the polar form: 5(cos(0.9273) + isin(0.9273)).

2. Finding the Magnitude and Angle of Complex Numbers
A Polar Form of Complex Numbers Calculator can also be used to find the magnitude and angle of complex numbers. The magnitude of a complex number is its length, while the angle is its argument.
For example, let's say we want to find the magnitude and angle of the complex number 3 + 4i. Using a Polar Form of Complex Numbers Calculator, we can enter the complex number and get the magnitude (5) and angle (0.9273).
Benefits of Finding Magnitude and Angle
Finding the magnitude and angle of complex numbers can be useful in many applications, such as:
- Signal processing: The magnitude and angle of complex numbers can be used to represent the amplitude and phase of signals.
- Navigation: The magnitude and angle of complex numbers can be used to represent the distance and direction of objects.

3. Performing Arithmetic Operations
A Polar Form of Complex Numbers Calculator can also be used to perform arithmetic operations, such as addition, subtraction, multiplication, and division.
For example, let's say we want to multiply the complex numbers 3 + 4i and 2 + 3i. Using a Polar Form of Complex Numbers Calculator, we can enter the complex numbers and get the result: -6 + 17i.

4. Simplifying Complex Expressions
A Polar Form of Complex Numbers Calculator can also be used to simplify complex expressions.
For example, let's say we want to simplify the expression (3 + 4i)(2 + 3i). Using a Polar Form of Complex Numbers Calculator, we can enter the expression and get the result: -6 + 17i.
Benefits of Simplifying Complex Expressions
Simplifying complex expressions can be useful in many applications, such as:
- Algebra: Simplifying complex expressions can make it easier to solve algebraic equations.
- Calculus: Simplifying complex expressions can make it easier to evaluate integrals and derivatives.

5. Visualizing Complex Numbers
A Polar Form of Complex Numbers Calculator can also be used to visualize complex numbers.
For example, let's say we want to visualize the complex number 3 + 4i. Using a Polar Form of Complex Numbers Calculator, we can enter the complex number and get a graph of the complex number in the complex plane.
Benefits of Visualizing Complex Numbers
Visualizing complex numbers can be useful in many applications, such as:
- Education: Visualizing complex numbers can make it easier for students to understand complex number theory.
- Research: Visualizing complex numbers can make it easier for researchers to analyze complex data.

Conclusion
In conclusion, a Polar Form of Complex Numbers Calculator is a valuable tool for converting complex numbers into their polar form, finding the magnitude and angle of complex numbers, performing arithmetic operations, simplifying complex expressions, and visualizing complex numbers. Whether you're a student, researcher, or engineer, a Polar Form of Complex Numbers Calculator can help you to work more efficiently and effectively with complex numbers.

What is the polar form of a complex number?
+The polar form of a complex number is represented as r(cosθ + isinθ), where r is the magnitude (or length) of the complex number, and θ is the angle (or argument) of the complex number.
How do I use a Polar Form of Complex Numbers Calculator?
+To use a Polar Form of Complex Numbers Calculator, simply enter the complex number in the form a + bi, and the calculator will convert it into its polar form.
What are the benefits of using a Polar Form of Complex Numbers Calculator?
+The benefits of using a Polar Form of Complex Numbers Calculator include converting complex numbers into their polar form, finding the magnitude and angle of complex numbers, performing arithmetic operations, simplifying complex expressions, and visualizing complex numbers.