Solving Point Slope Form Example Problems Made Easy

Quick Links :

Unlocking the Power of Point Slope Form: A Comprehensive Guide

Point slope form is a fundamental concept in algebra, and it's essential to master it to solve various math problems. If you're struggling to understand point slope form, you're not alone. Many students find it challenging to grasp, but with the right approach, it can become a breeze. In this article, we'll delve into the world of point slope form, explore its significance, and provide step-by-step examples to help you become proficient in solving point slope form problems.

What is Point Slope Form?

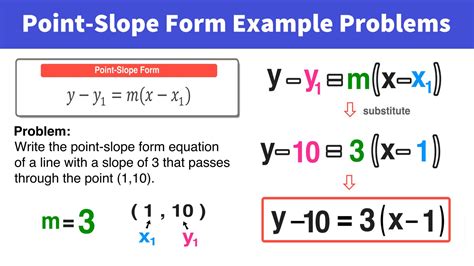
Point slope form is a way of expressing a linear equation in mathematics. It's a formula that uses the slope of a line and a point on the line to represent the equation. The point slope form formula is:

y - y1 = m(x - x1)

where:

• (x1, y1) is a point on the line
• m is the slope of the line
• x and y are the variables

Why is Point Slope Form Important?

Point slope form is crucial in mathematics because it allows us to:

• Write linear equations in a convenient form
• Find the equation of a line given a point and the slope
• Solve problems involving linear equations

How to Solve Point Slope Form Example Problems

Now that we've covered the basics, let's dive into some point slope form example problems. We'll start with simple problems and gradually move on to more complex ones.

Example 1: Find the equation of a line given a point and the slope

Problem: Find the equation of a line that passes through the point (2, 3) and has a slope of 4.

Solution:

Using the point slope form formula, we get:

y - 3 = 4(x - 2)

Simplifying the equation, we get:

y - 3 = 4x - 8

y = 4x - 5

Example 2: Find the equation of a line given two points

Problem: Find the equation of a line that passes through the points (1, 2) and (3, 4).

Solution:

First, we need to find the slope of the line. We can do this by using the slope formula:

m = (y2 - y1) / (x2 - x1)

m = (4 - 2) / (3 - 1)

m = 2 / 2

m = 1

Now that we have the slope, we can use the point slope form formula to find the equation of the line. We'll use the point (1, 2) as our reference point.

y - 2 = 1(x - 1)

Simplifying the equation, we get:

y - 2 = x - 1

y = x + 1

Example 3: Solve a linear equation in point slope form

Problem: Solve the equation 2y - 4 = 3(x - 1) for y.

Solution:

We can start by isolating y on one side of the equation.

2y - 4 = 3(x - 1)

2y = 3(x - 1) + 4

2y = 3x - 3 + 4

2y = 3x + 1

y = (3x + 1) / 2

Common Mistakes to Avoid

When solving point slope form problems, there are a few common mistakes to avoid:

• Forgetting to include the negative sign when subtracting x1 from x
• Not simplifying the equation properly
• Not checking the solution to ensure it's correct

Practical Applications of Point Slope Form

Point slope form has numerous practical applications in various fields, including:

• Physics: to describe the motion of objects
• Engineering: to model real-world systems
• Computer Science: to create algorithms for solving linear equations

Conclusion: Mastering Point Slope Form

Point slope form is a powerful tool for solving linear equations. By mastering point slope form, you'll be able to tackle a wide range of math problems with confidence. Remember to practice regularly and avoid common mistakes. With time and effort, you'll become proficient in solving point slope form problems and unlock the secrets of linear equations.