The point-slope form is a fundamental concept in mathematics, specifically in algebra and geometry. It's a powerful tool used to describe the equation of a line in a two-dimensional coordinate system. In this article, we'll delve into the world of point-slope form, exploring its definition, benefits, and practical applications.
Understanding the point-slope form can seem daunting at first, but with a little practice and patience, you'll be able to grasp this concept with ease. The point-slope form is a way to express the equation of a line using the slope and a point on the line. This form is particularly useful when you know the slope of a line and a point on it, but not the y-intercept.

What is Point Slope Form?
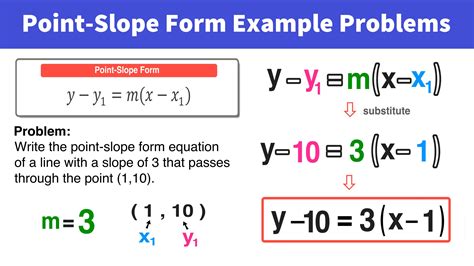
The point-slope form is a way to write the equation of a line in the form y - y1 = m(x - x1), where m is the slope of the line, and (x1, y1) is a point on the line. This form is useful when you know the slope of a line and a point on it, but not the y-intercept. The point-slope form is a versatile equation that can be used to find the equation of a line, given the slope and a point on it.
How to Use the Point Slope Form
Using the point-slope form is relatively straightforward. To write the equation of a line in point-slope form, follow these steps:
- Identify the slope of the line (m).
- Identify a point on the line (x1, y1).
- Plug the values of m, x1, and y1 into the point-slope form equation: y - y1 = m(x - x1).
For example, let's say we want to write the equation of a line with a slope of 2 and passing through the point (3, 4). Using the point-slope form, the equation would be:
y - 4 = 2(x - 3)
Benefits of Point Slope Form
The point-slope form has several benefits that make it a useful tool in mathematics. Some of the benefits include:
- Easy to use: The point-slope form is relatively easy to use, especially when you know the slope of a line and a point on it.
- Flexible: The point-slope form can be used to find the equation of a line, given the slope and a point on it.
- Helpful in graphing: The point-slope form can be used to graph lines, especially when you know the slope and a point on it.

Real-World Applications of Point Slope Form
The point-slope form has several real-world applications, including:
- Physics: The point-slope form is used in physics to describe the motion of objects, including the slope of a line representing the velocity of an object.
- Engineering: The point-slope form is used in engineering to design and analyze systems, including the slope of a line representing the stress on a beam.
- Computer Science: The point-slope form is used in computer science to create algorithms for graphing lines and curves.
Example Problems
Here are some example problems to help you understand the point-slope form:
- Find the equation of a line with a slope of 3 and passing through the point (2, 5).
- Find the equation of a line with a slope of -2 and passing through the point (-1, 3).
Solutions:
- y - 5 = 3(x - 2)
- y - 3 = -2(x + 1)
Common Mistakes to Avoid
When using the point-slope form, there are several common mistakes to avoid. Some of these mistakes include:
- Incorrectly identifying the slope: Make sure to correctly identify the slope of the line.
- Incorrectly identifying the point: Make sure to correctly identify the point on the line.

Conclusion and Final Thoughts
The point-slope form is a powerful tool in mathematics, used to describe the equation of a line in a two-dimensional coordinate system. With its ease of use and flexibility, the point-slope form is a versatile equation that can be used in a variety of real-world applications. By understanding the point-slope form, you'll be able to tackle a range of math problems with confidence.
What do you think about the point-slope form? Do you have any questions or examples you'd like to share? Let us know in the comments below!
What is the point-slope form?
+The point-slope form is a way to write the equation of a line in the form y - y1 = m(x - x1), where m is the slope of the line, and (x1, y1) is a point on the line.
How do I use the point-slope form?
+To use the point-slope form, identify the slope of the line (m) and a point on the line (x1, y1), then plug these values into the point-slope form equation: y - y1 = m(x - x1).
What are the benefits of the point-slope form?
+The point-slope form is easy to use, flexible, and helpful in graphing lines. It's also used in a variety of real-world applications, including physics, engineering, and computer science.