Simplify Fractions: Multiply And Express In Simplest Form

Quick Links :

Simplifying fractions is an essential skill in mathematics, particularly when dealing with fractions in various mathematical operations. One of the key concepts in simplifying fractions is multiplying and expressing them in their simplest form. In this article, we will delve into the world of fractions, exploring the concept of simplifying fractions by multiplying and expressing them in their simplest form.

What are Fractions?

Fractions are a way of representing a part of a whole. They consist of two parts: the numerator and the denominator. The numerator tells us how many equal parts we have, while the denominator tells us how many parts the whole is divided into. For example, in the fraction 3/4, the numerator is 3, and the denominator is 4. This means we have 3 equal parts out of a total of 4 parts.

Why Simplify Fractions?

Simplifying fractions is essential in mathematics because it makes it easier to work with fractions in various operations. When fractions are in their simplest form, it becomes easier to add, subtract, multiply, and divide them. Simplifying fractions also helps to avoid confusion and errors in mathematical calculations.

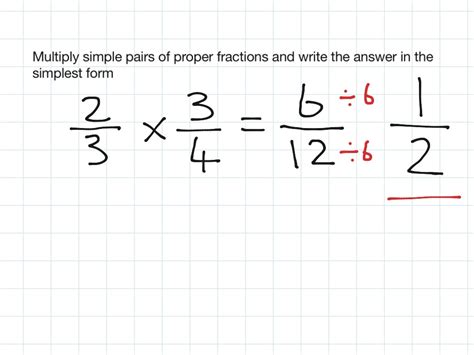
Multiplying Fractions

Multiplying fractions is a straightforward process. To multiply two fractions, we simply multiply the numerators and multiply the denominators. For example, to multiply 1/2 and 3/4, we multiply the numerators (1 x 3) and multiply the denominators (2 x 4). This gives us:

1/2 x 3/4 = (1 x 3) / (2 x 4) = 3/8

Expressing Fractions in Simplest Form

Expressing fractions in their simplest form involves dividing both the numerator and the denominator by the greatest common divisor (GCD). The GCD is the largest number that divides both the numerator and the denominator without leaving a remainder. For example, to simplify the fraction 6/8, we find the GCD of 6 and 8, which is 2. We then divide both the numerator and the denominator by 2:

6 ÷ 2 = 3 8 ÷ 2 = 4

This gives us the simplified fraction 3/4.

Steps to Simplify Fractions

Here are the steps to simplify fractions:

1. Find the GCD: Find the greatest common divisor of the numerator and the denominator.
2. Divide the numerator: Divide the numerator by the GCD.
3. Divide the denominator: Divide the denominator by the GCD.
4. Write the simplified fraction: Write the simplified fraction with the new numerator and denominator.

Examples of Simplifying Fractions

Here are some examples of simplifying fractions:

• Simplify the fraction 12/16:
  • Find the GCD: 4
  • Divide the numerator: 12 ÷ 4 = 3
  • Divide the denominator: 16 ÷ 4 = 4
  • Write the simplified fraction: 3/4
• Simplify the fraction 8/10:
  • Find the GCD: 2
  • Divide the numerator: 8 ÷ 2 = 4
  • Divide the denominator: 10 ÷ 2 = 5
  • Write the simplified fraction: 4/5

Benefits of Simplifying Fractions

Simplifying fractions has several benefits, including:

• Easier calculations: Simplifying fractions makes it easier to perform mathematical operations such as addition, subtraction, multiplication, and division.
• Avoid confusion: Simplifying fractions helps to avoid confusion and errors in mathematical calculations.
• Improved understanding: Simplifying fractions helps to improve understanding of mathematical concepts and relationships.

Conclusion

Simplifying fractions by multiplying and expressing them in their simplest form is an essential skill in mathematics. By following the steps outlined in this article, you can simplify fractions with ease and confidence. Remember to find the greatest common divisor, divide the numerator and denominator, and write the simplified fraction. With practice and patience, you can become proficient in simplifying fractions and perform mathematical operations with ease.