Mathway is an excellent online tool for solving mathematical problems, including those involving point-slope form. Point-slope form is a fundamental concept in algebra and geometry, allowing users to find the equation of a line given a point and the slope. Mastering point-slope form with Mathway can help you solve a wide range of problems in math and science. In this article, we will guide you through 5 easy steps to master Mathway point slope form.
Step 1: Understanding Point-Slope Form

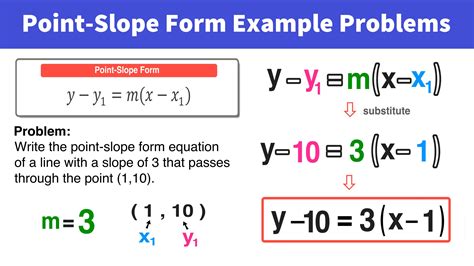
Point-slope form is a way of writing the equation of a line in the form y - y1 = m(x - x1), where (x1, y1) is a point on the line, and m is the slope. The slope represents the rate of change of the line, while the point provides a reference location on the line.
Why is Point-Slope Form Important?
Point-slope form is essential in various mathematical and scientific applications, including:
- Finding the equation of a line given a point and the slope
- Graphing lines and understanding their properties
- Solving systems of linear equations
- Analyzing functions and relationships between variables
Step 2: Using Mathway to Solve Point-Slope Form Problems

Mathway is an excellent online tool for solving point-slope form problems. To use Mathway, follow these steps:
- Go to the Mathway website or mobile app
- Enter the point-slope form equation in the input field
- Specify the values of the point (x1, y1) and the slope (m)
- Click the "Enter" button to solve the equation
Mathway will provide the solution in the form of the equation of the line, including the slope and the y-intercept.
Tips for Using Mathway Effectively
- Make sure to enter the correct values for the point and the slope
- Use the "Help" feature to understand the solution and the steps involved
- Practice solving different types of point-slope form problems to become proficient
Step 3: Mastering the Slope Formula

The slope formula is a critical component of point-slope form. The slope formula is given by:
m = (y2 - y1) / (x2 - x1)
where (x1, y1) and (x2, y2) are two points on the line.
How to Calculate Slope
- Identify two points on the line
- Calculate the difference in y-coordinates (y2 - y1)
- Calculate the difference in x-coordinates (x2 - x1)
- Divide the difference in y-coordinates by the difference in x-coordinates
The resulting value is the slope of the line.
Step 4: Graphing Lines in Point-Slope Form

Graphing lines in point-slope form involves plotting the point and using the slope to draw the line. To graph a line in point-slope form:
- Plot the point (x1, y1) on the coordinate plane
- Use the slope to determine the direction of the line
- Draw the line through the point, using the slope to guide the direction
Tips for Graphing Lines
- Use a ruler to draw the line accurately
- Label the point and the line clearly
- Use different colors to distinguish between multiple lines
Step 5: Applying Point-Slope Form in Real-World Applications

Point-slope form has numerous real-world applications, including:
- Physics and engineering: to model the motion of objects and design systems
- Economics: to analyze supply and demand curves
- Computer science: to develop algorithms and model complex systems
Examples of Real-World Applications
- A car travels from point A to point B at a constant speed. Use point-slope form to find the equation of the line representing the car's motion.
- A company's profit is directly proportional to the number of units sold. Use point-slope form to find the equation of the line representing the relationship between profit and units sold.
By mastering Mathway point slope form, you can solve a wide range of problems in math and science, and develop a deeper understanding of the underlying concepts. Remember to practice regularly and apply the concepts to real-world applications.
What is point-slope form?
+Point-slope form is a way of writing the equation of a line in the form y - y1 = m(x - x1), where (x1, y1) is a point on the line, and m is the slope.
How do I calculate the slope of a line?
+The slope formula is given by m = (y2 - y1) / (x2 - x1), where (x1, y1) and (x2, y2) are two points on the line.
What are some real-world applications of point-slope form?
+Point-slope form has numerous real-world applications, including physics and engineering, economics, and computer science.
We hope this article has helped you master Mathway point slope form. If you have any questions or need further clarification, please don't hesitate to ask. Share your thoughts and experiences in the comments below.