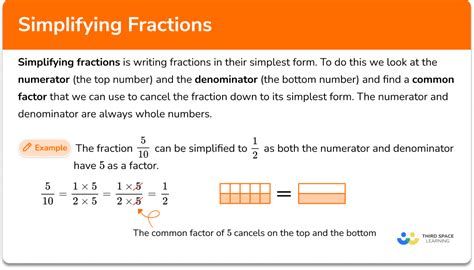
Simplifying Fractions: Converting To Simplest Form Lesson 5

Quick Links :

Simplifying fractions is an essential math skill that can help make calculations easier and more efficient. In this article, we will explore the concept of simplifying fractions, also known as converting fractions to their simplest form. We will discuss the importance of simplifying fractions, the steps involved in the process, and provide examples to illustrate the concept.

Why Simplify Fractions?

Simplifying fractions is important because it makes it easier to compare, add, subtract, multiply, and divide fractions. When fractions are in their simplest form, they are easier to work with and understand. Simplifying fractions also helps to avoid confusion and errors when performing mathematical operations. Additionally, simplifying fractions is a fundamental skill that is used in various areas of mathematics, such as algebra, geometry, and calculus.

What is a Simplest Form?

A simplest form is a fraction that has no common factors between the numerator and the denominator, other than 1. In other words, the numerator and the denominator are relatively prime. For example, the fraction 3/4 is in its simplest form because there are no common factors between 3 and 4, other than 1.

Steps to Simplify Fractions

Simplifying fractions involves dividing the numerator and the denominator by their greatest common divisor (GCD). Here are the steps to simplify fractions:

1. Find the GCD of the numerator and the denominator.
2. Divide the numerator and the denominator by the GCD.
3. Write the result as a simplified fraction.

Examples of Simplifying Fractions

Here are a few examples of simplifying fractions:

• Simplify the fraction 6/8:
  • Find the GCD of 6 and 8, which is 2.
  • Divide the numerator and the denominator by 2: 6 ÷ 2 = 3, 8 ÷ 2 = 4.
  • Write the result as a simplified fraction: 3/4.
• Simplify the fraction 12/16:
  • Find the GCD of 12 and 16, which is 4.
  • Divide the numerator and the denominator by 4: 12 ÷ 4 = 3, 16 ÷ 4 = 4.
  • Write the result as a simplified fraction: 3/4.
• Simplify the fraction 24/30:
  • Find the GCD of 24 and 30, which is 6.
  • Divide the numerator and the denominator by 6: 24 ÷ 6 = 4, 30 ÷ 6 = 5.
  • Write the result as a simplified fraction: 4/5.

Tips for Simplifying Fractions

Here are a few tips for simplifying fractions:

• Always find the GCD of the numerator and the denominator.
• Divide the numerator and the denominator by the GCD.
• Write the result as a simplified fraction.
• Check your work by multiplying the numerator and the denominator to make sure the result is the same as the original fraction.

Common Mistakes to Avoid

Here are a few common mistakes to avoid when simplifying fractions:

• Not finding the GCD of the numerator and the denominator.
• Dividing the numerator and the denominator by a number that is not the GCD.
• Not writing the result as a simplified fraction.

Real-World Applications of Simplifying Fractions

Simplifying fractions has many real-world applications, such as:

• Cooking: Recipes often involve fractions, and simplifying them can make it easier to measure ingredients.
• Building: Fractions are used in construction to measure materials and calculate quantities.
• Science: Fractions are used in scientific measurements and calculations.

Conclusion

In conclusion, simplifying fractions is an essential math skill that can make calculations easier and more efficient. By following the steps outlined in this article and practicing with examples, you can become proficient in simplifying fractions. Remember to always find the GCD of the numerator and the denominator, divide by the GCD, and write the result as a simplified fraction.

We hope this article has been helpful in explaining the concept of simplifying fractions. If you have any questions or comments, please feel free to share them with us.