Intercept Form Of A Quadratic Function Defined

Quick Links :

The intercept form of a quadratic function is a fundamental concept in mathematics, particularly in algebra. It provides a unique way of expressing quadratic functions, which are commonly used to model real-world phenomena. In this article, we will delve into the world of quadratic functions, exploring their intercept form, and discussing its significance, benefits, and practical applications.

What is the Intercept Form of a Quadratic Function?

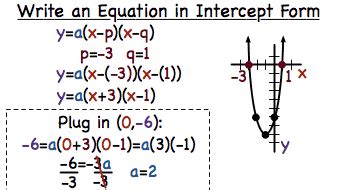
The intercept form of a quadratic function is a way of expressing a quadratic function in the form:

f(x) = a(x - p)(x - q)

where a, p, and q are constants, and x is the variable. This form is also known as the factored form or the vertex form.

Understanding the Components of the Intercept Form

To fully grasp the intercept form, it's essential to understand its components:

• a: This is the leading coefficient, which determines the direction and width of the parabola.
• p and q: These are the x-intercepts, which represent the points where the parabola intersects the x-axis.

By expressing a quadratic function in intercept form, we can easily identify its x-intercepts, vertex, and axis of symmetry.

Benefits of the Intercept Form

The intercept form offers several benefits, making it a valuable tool in mathematics and real-world applications:

• Easy identification of x-intercepts: The intercept form allows us to quickly identify the x-intercepts of a quadratic function, which is crucial in solving equations and inequalities.
• Vertex and axis of symmetry: The intercept form also enables us to find the vertex and axis of symmetry of a parabola, which are essential in graphing and analyzing quadratic functions.
• Simplified calculations: The intercept form can simplify calculations, especially when dealing with complex quadratic functions.

How to Convert a Quadratic Function to Intercept Form

Converting a quadratic function to intercept form involves factoring the quadratic expression. Here's a step-by-step guide:

1. Factor the quadratic expression: Factor the quadratic expression into two binomial factors.
2. Identify the x-intercepts: Identify the x-intercepts by setting each factor equal to zero.
3. Write the intercept form: Write the quadratic function in intercept form using the x-intercepts.

For example, consider the quadratic function f(x) = x^2 + 5x + 6. To convert it to intercept form, we factor the quadratic expression:

f(x) = (x + 2)(x + 3)

The x-intercepts are x = -2 and x = -3. Therefore, the intercept form is:

f(x) = (x + 2)(x + 3)

Practical Applications of the Intercept Form

The intercept form has numerous practical applications in various fields, including:

• Physics and engineering: Quadratic functions are used to model the motion of objects under the influence of gravity or other forces.
• Economics: Quadratic functions are used to model the behavior of economic systems, such as supply and demand curves.
• Computer science: Quadratic functions are used in algorithms for solving equations and inequalities.

Conclusion and Future Directions

In conclusion, the intercept form of a quadratic function is a powerful tool for expressing and analyzing quadratic functions. Its benefits, including easy identification of x-intercepts, vertex, and axis of symmetry, make it a valuable resource in mathematics and real-world applications.

As you continue to explore the world of quadratic functions, remember to:

• Practice converting quadratic functions to intercept form.
• Apply the intercept form to solve equations and inequalities.
• Explore the many practical applications of the intercept form.

Share your thoughts and questions in the comments below, and don't forget to share this article with your friends and colleagues.