6 Ways To Simplify Radicals In Math Problems

Quick Links :

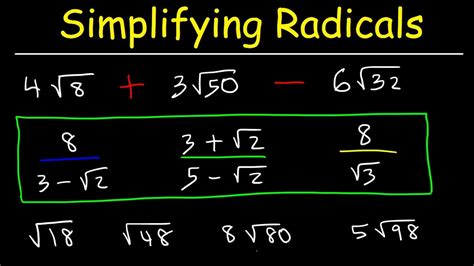
Radicals are an essential part of mathematics, particularly in algebra and geometry. They represent a way to express numbers that cannot be simplified into a whole number or a decimal. However, working with radicals can be daunting, especially when faced with complex math problems. Fortunately, there are several ways to simplify radicals, making it easier to solve equations and manipulate expressions.

What are Radicals and Why Simplify Them?

Radicals, also known as roots, are mathematical symbols used to represent the nth root of a number. The most common radical is the square root (√), which represents the number that, when multiplied by itself, gives the original value. For example, √16 = 4, because 4 multiplied by 4 equals 16. Simplifying radicals is essential in mathematics, as it helps to:

• Reduce complex expressions to more manageable forms
• Facilitate calculations and algebraic manipulations
• Enhance understanding and visualization of mathematical concepts

Why Simplify Radicals?

Simplifying radicals is crucial in various areas of mathematics, including algebra, geometry, trigonometry, and calculus. By simplifying radicals, students can:

• Develop problem-solving skills and strategies
• Improve their understanding of mathematical concepts and relationships
• Enhance their ability to communicate mathematical ideas effectively

6 Ways to Simplify Radicals in Math Problems

There are several techniques to simplify radicals in math problems. Here are six ways to simplify radicals:

1. Simplifying Square Roots

Simplifying square roots involves finding the largest perfect square that divides the radicand (the number inside the radical). For example:

√16 = √(4 × 4) = 4

In this example, 4 is the largest perfect square that divides 16, so the square root of 16 is simplified to 4.

2. Simplifying Cube Roots

Simplifying cube roots involves finding the largest perfect cube that divides the radicand. For example:

³√27 = ³√(3 × 3 × 3) = 3

In this example, 3 is the largest perfect cube that divides 27, so the cube root of 27 is simplified to 3.

3. Simplifying Radicals with Variables

Simplifying radicals with variables involves combining like terms and using algebraic properties to simplify the expression. For example:

√(x^2 + 4x + 4) = √((x + 2)^2) = x + 2

In this example, the expression inside the radical is factored, and the resulting expression is simplified using algebraic properties.

4. Simplifying Radicals with Rational Exponents

Simplifying radicals with rational exponents involves converting the radical to a rational exponent and then simplifying the resulting expression. For example:

³√(x^2) = x^(2/3)

In this example, the radical is converted to a rational exponent, and the resulting expression is simplified using algebraic properties.

5. Simplifying Radicals with Absolute Value

Simplifying radicals with absolute value involves removing the absolute value sign and simplifying the resulting expression. For example:

|√(x^2)| = x

In this example, the absolute value sign is removed, and the resulting expression is simplified using algebraic properties.

6. Simplifying Radicals with Conjugates

Simplifying radicals with conjugates involves multiplying the radical by its conjugate and then simplifying the resulting expression. For example:

√(x + 1) = (√(x + 1)) × (√(x - 1)) / (√(x - 1)) = (x + 1) / √(x - 1)

In this example, the radical is multiplied by its conjugate, and the resulting expression is simplified using algebraic properties.

Practical Examples of Simplifying Radicals

Here are some practical examples of simplifying radicals:

• Simplifying √(16x^2) = 4x
• Simplifying ³√(27x^3) = 3x
• Simplifying √(x^2 + 4x + 4) = x + 2
• Simplifying ³√(x^2) = x^(2/3)
• Simplifying |√(x^2)| = x
• Simplifying √(x + 1) = (√(x + 1)) × (√(x - 1)) / (√(x - 1)) = (x + 1) / √(x - 1)

By mastering these six techniques, students can simplify radicals with confidence and accuracy, making it easier to solve math problems and understand complex mathematical concepts.

Conclusion: Simplifying Radicals Made Easy

Simplifying radicals is an essential skill in mathematics, and with practice and patience, students can master the techniques outlined in this article. By understanding the different types of radicals and how to simplify them, students can develop problem-solving skills, improve their understanding of mathematical concepts, and enhance their ability to communicate mathematical ideas effectively.

We hope this article has been informative and helpful in your journey to simplify radicals. If you have any questions or comments, please feel free to share them below.