The world of mathematics is filled with fascinating concepts, and one of the most intriguing is the hyperbola. A hyperbola is a type of curve that is defined by a specific equation, and it has a wide range of applications in various fields, including physics, engineering, and computer science. In this article, we will delve into the world of hyperbolas and explore five essential equation forms that will help you master this complex concept.
Understanding Hyperbolas

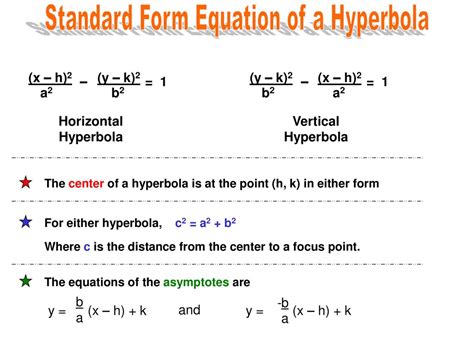
A hyperbola is a curve that is defined by a specific equation, which is typically written in the form of (x^2/a^2) - (y^2/b^2) = 1. This equation represents a curve that has two distinct branches, which are separated by a central axis. The hyperbola is symmetrical about this axis, and it has two vertices, which are the points where the curve intersects the axis.
The study of hyperbolas is essential in various fields, including physics, engineering, and computer science. In physics, hyperbolas are used to describe the motion of objects under the influence of gravity, while in engineering, they are used to design and optimize systems such as satellite dishes and radio antennas. In computer science, hyperbolas are used in computer graphics and game development to create realistic models of curves and surfaces.
Why Mastering Hyperbola Equation Forms is Important
Mastering hyperbola equation forms is essential for anyone who wants to excel in mathematics, physics, engineering, or computer science. By understanding the different equation forms of hyperbolas, you will be able to analyze and solve problems that involve these curves. Additionally, you will be able to apply your knowledge of hyperbolas to real-world problems, which will give you a competitive edge in your chosen field.
Equation Form 1: Standard Form

The standard form of a hyperbola is (x^2/a^2) - (y^2/b^2) = 1. This equation represents a hyperbola that is centered at the origin (0,0) and has its transverse axis along the x-axis. The values of a and b determine the shape and size of the hyperbola.
To graph a hyperbola in standard form, you can use the following steps:
- Determine the values of a and b
- Plot the vertices of the hyperbola at (±a,0)
- Plot the co-vertices of the hyperbola at (0,±b)
- Draw the asymptotes of the hyperbola, which are the lines that the curve approaches as x and y approach infinity
Example of Standard Form
Find the equation of a hyperbola that has a transverse axis of length 10 and a conjugate axis of length 6.
Solution: The equation of the hyperbola is (x^2/25) - (y^2/9) = 1.
Equation Form 2: Transverse Axis

The transverse axis form of a hyperbola is (x^2/a^2) - (y^2/b^2) = 1, where the transverse axis is along the x-axis. This equation represents a hyperbola that is centered at the origin (0,0) and has its transverse axis along the x-axis.
To graph a hyperbola in transverse axis form, you can use the following steps:
- Determine the values of a and b
- Plot the vertices of the hyperbola at (±a,0)
- Plot the co-vertices of the hyperbola at (0,±b)
- Draw the asymptotes of the hyperbola, which are the lines that the curve approaches as x and y approach infinity
Example of Transverse Axis
Find the equation of a hyperbola that has a transverse axis of length 8 and a conjugate axis of length 4.
Solution: The equation of the hyperbola is (x^2/16) - (y^2/4) = 1.
Equation Form 3: Conjugate Axis

The conjugate axis form of a hyperbola is (y^2/a^2) - (x^2/b^2) = 1, where the conjugate axis is along the y-axis. This equation represents a hyperbola that is centered at the origin (0,0) and has its conjugate axis along the y-axis.
To graph a hyperbola in conjugate axis form, you can use the following steps:
- Determine the values of a and b
- Plot the vertices of the hyperbola at (0,±a)
- Plot the co-vertices of the hyperbola at (±b,0)
- Draw the asymptotes of the hyperbola, which are the lines that the curve approaches as x and y approach infinity
Example of Conjugate Axis
Find the equation of a hyperbola that has a conjugate axis of length 12 and a transverse axis of length 8.
Solution: The equation of the hyperbola is (y^2/144) - (x^2/64) = 1.
Equation Form 4: Centered at (h,k)

The centered at (h,k) form of a hyperbola is ((x-h)^2/a^2) - ((y-k)^2/b^2) = 1. This equation represents a hyperbola that is centered at the point (h,k) and has its transverse axis along the x-axis.
To graph a hyperbola in centered at (h,k) form, you can use the following steps:
- Determine the values of a, b, h, and k
- Plot the center of the hyperbola at (h,k)
- Plot the vertices of the hyperbola at (h±a,k)
- Plot the co-vertices of the hyperbola at (h,k±b)
- Draw the asymptotes of the hyperbola, which are the lines that the curve approaches as x and y approach infinity
Example of Centered at (h,k)
Find the equation of a hyperbola that is centered at the point (2,3) and has a transverse axis of length 6 and a conjugate axis of length 4.
Solution: The equation of the hyperbola is ((x-2)^2/9) - ((y-3)^2/4) = 1.
Equation Form 5: Rotation

The rotation form of a hyperbola is ((x-h)^2/a^2) - ((y-k)^2/b^2) = 1, where the hyperbola is rotated by an angle θ. This equation represents a hyperbola that is centered at the point (h,k) and has its transverse axis along the x-axis.
To graph a hyperbola in rotation form, you can use the following steps:
- Determine the values of a, b, h, k, and θ
- Plot the center of the hyperbola at (h,k)
- Plot the vertices of the hyperbola at (h±a,k)
- Plot the co-vertices of the hyperbola at (h,k±b)
- Draw the asymptotes of the hyperbola, which are the lines that the curve approaches as x and y approach infinity
Example of Rotation
Find the equation of a hyperbola that is centered at the point (2,3) and has a transverse axis of length 6 and a conjugate axis of length 4. The hyperbola is rotated by an angle of 45°.
Solution: The equation of the hyperbola is ((x-2)^2/9) - ((y-3)^2/4) = 1, where the hyperbola is rotated by an angle of 45°.
We hope this article has helped you master the five essential equation forms of hyperbolas. By understanding these equation forms, you will be able to analyze and solve problems that involve hyperbolas, and apply your knowledge to real-world problems.
Do you have any questions or comments about this article? Share your thoughts with us in the comments section below!