Write A Parabola Equation In Standard Form Easily

Quick Links :

Understanding the concept of parabolas and their equations is a fundamental aspect of algebra and geometry. A parabola is a type of curve that is shaped like a U or a bowl, and it can be described using a quadratic equation. The standard form of a parabola equation is essential for analyzing and solving problems related to these curves.

What is a Parabola?

A parabola is a set of points that are equidistant from a fixed point called the focus and a fixed line called the directrix. The parabola can be oriented vertically or horizontally, and it can be described using a quadratic equation. The parabola is a fundamental concept in mathematics, physics, and engineering, and it has numerous applications in various fields.

Standard Form of a Parabola Equation

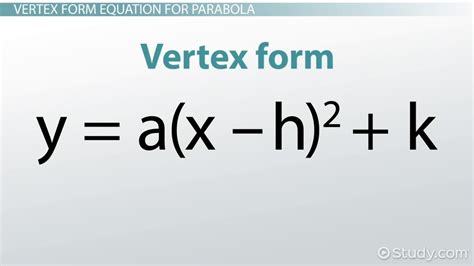
The standard form of a parabola equation is:

y = a(x - h)^2 + k

or

x = a(y - k)^2 + h

where (h, k) is the vertex of the parabola, and 'a' is a coefficient that determines the direction and width of the parabola.

Understanding the Variables

• (h, k) is the vertex of the parabola, which is the lowest or highest point of the curve.
• 'a' is a coefficient that determines the direction and width of the parabola. If 'a' is positive, the parabola opens upwards; if 'a' is negative, the parabola opens downwards.
• (x - h) or (y - k) is the horizontal or vertical distance from the vertex to a point on the parabola.

How to Write a Parabola Equation in Standard Form

To write a parabola equation in standard form, follow these steps:

1. Determine the vertex (h, k) of the parabola.
2. Determine the direction and width of the parabola, which is determined by the coefficient 'a'.
3. Write the equation in the standard form y = a(x - h)^2 + k or x = a(y - k)^2 + h.

Example 1: Write a Parabola Equation in Standard Form

Find the equation of a parabola with vertex (2, 3) and a coefficient 'a' of 2.

Solution:

y = 2(x - 2)^2 + 3

Example 2: Write a Parabola Equation in Standard Form

Find the equation of a parabola with vertex (-1, 4) and a coefficient 'a' of -3.

Solution:

y = -3(x + 1)^2 + 4

Real-World Applications of Parabola Equations

Parabola equations have numerous applications in various fields, including physics, engineering, and computer science. Some examples include:

• Projectile motion: The trajectory of a projectile can be described using a parabola equation.
• Mirrors and lenses: Parabola equations can be used to describe the shape of mirrors and lenses.
• Electronic circuits: Parabola equations can be used to analyze electronic circuits.

Conclusion

Writing a parabola equation in standard form is an essential skill in algebra and geometry. By understanding the concept of parabolas and the standard form of their equations, you can analyze and solve problems related to these curves. With numerous applications in various fields, parabola equations are an important tool in mathematics and science.

We hope this article has been informative and helpful. Do you have any questions or comments about parabola equations? Please share them with us.