Quadratic Equations In Standard Form Made Easy

Quick Links :

Quadratic equations are a fundamental concept in algebra, and understanding how to solve them is crucial for success in mathematics and science. However, for many students, quadratic equations can seem daunting, especially when they are presented in standard form. In this article, we will break down the concept of quadratic equations in standard form, explore their importance, and provide a step-by-step guide on how to solve them.

Quadratic equations are polynomial equations of degree two, which means the highest power of the variable (usually x) is two. They have the general form of ax^2 + bx + c = 0, where a, b, and c are constants. Quadratic equations can be solved using various methods, including factoring, the quadratic formula, and graphing.

What is Standard Form?

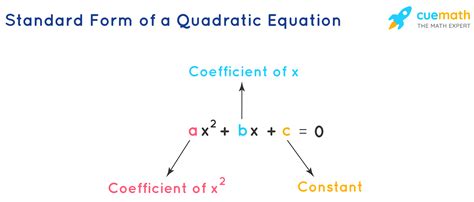
Standard form is a way of writing quadratic equations in a specific format, which makes it easier to identify the coefficients and solve the equation. The standard form of a quadratic equation is ax^2 + bx + c = 0, where a, b, and c are constants, and a cannot be zero. This format is useful because it allows us to easily identify the coefficients and apply various solving techniques.

Why are Quadratic Equations Important?

Quadratic equations have numerous applications in mathematics, science, and engineering. They are used to model real-world phenomena, such as the trajectory of a projectile, the shape of a mirror, and the growth of a population. Quadratic equations are also used in various fields, including physics, chemistry, and computer science.

Quadratic equations are also essential in problem-solving and critical thinking. They require students to think creatively and apply mathematical concepts to solve problems. By mastering quadratic equations, students can develop their problem-solving skills and prepare themselves for more advanced math concepts.

How to Solve Quadratic Equations in Standard Form

Solving quadratic equations in standard form involves several steps, which we will outline below:

Step 1: Factor the Equation (If Possible)

Factoring is a method of solving quadratic equations by expressing them as a product of two binomials. If the equation can be factored, it can be written in the form (x + m)(x + n) = 0, where m and n are constants.

For example, the equation x^2 + 5x + 6 = 0 can be factored as (x + 2)(x + 3) = 0.

Step 2: Use the Quadratic Formula

If the equation cannot be factored, the quadratic formula can be used. The quadratic formula is x = (-b ± √(b^2 - 4ac)) / 2a, where a, b, and c are the coefficients of the equation.

For example, the equation x^2 + 4x + 5 = 0 can be solved using the quadratic formula:

x = (-4 ± √(4^2 - 4(1)(5))) / 2(1) x = (-4 ± √(16 - 20)) / 2 x = (-4 ± √(-4)) / 2 x = (-4 ± 2i) / 2 x = -2 ± i

Step 3: Graph the Equation

Graphing is a method of solving quadratic equations by plotting the graph of the related function. The x-intercepts of the graph represent the solutions to the equation.

For example, the equation x^2 + 2x - 6 = 0 can be graphed as follows:

The x-intercepts of the graph are -3 and 2, which represent the solutions to the equation.

Practical Applications of Quadratic Equations

Quadratic equations have numerous practical applications in mathematics, science, and engineering. Here are a few examples:

• Projectile Motion: Quadratic equations are used to model the trajectory of projectiles, such as the path of a thrown ball or the trajectory of a rocket.
• Mirrors and Lenses: Quadratic equations are used to model the shape of mirrors and lenses, which are used in optics and photonics.
• Population Growth: Quadratic equations are used to model the growth of populations, which is essential in biology and ecology.

Conclusion

Quadratic equations in standard form are a fundamental concept in algebra, and understanding how to solve them is crucial for success in mathematics and science. By mastering quadratic equations, students can develop their problem-solving skills and prepare themselves for more advanced math concepts. With practice and patience, anyone can become proficient in solving quadratic equations in standard form.