Convert Factored Form To Standard Form Easily Today

Quick Links :

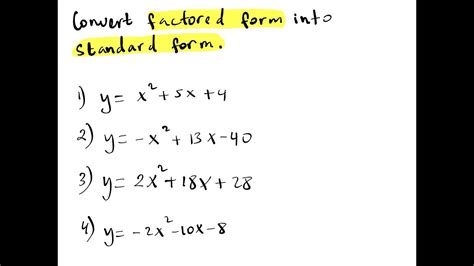
Converting factored form to standard form is an essential skill in algebra, and it's easier than you think. In this article, we'll explore the step-by-step process of converting factored form to standard form, providing you with a comprehensive understanding of the concept.

Understanding Factored Form and Standard Form

Before we dive into the conversion process, let's define what factored form and standard form mean in algebra.

Factored form refers to an expression that is written as a product of its factors, whereas standard form represents an expression in its simplest form, without any factors.

For example, the expression (x + 3)(x - 2) is in factored form, while the expression x^2 + x - 6 is in standard form.

Converting Factored Form to Standard Form: A Step-by-Step Guide

Converting factored form to standard form involves multiplying the factors together using the distributive property. Here's a step-by-step guide to help you convert factored form to standard form:

1. Identify the factors: Start by identifying the factors in the factored form expression. For example, in the expression (x + 3)(x - 2), the factors are (x + 3) and (x - 2).
2. Multiply the factors: Multiply the factors together using the distributive property. This means multiplying each term in the first factor by each term in the second factor.
3. Combine like terms: Combine any like terms that result from the multiplication process.
4. Simplify the expression: Simplify the resulting expression by combining any constants and rearranging the terms.

Let's use the example (x + 3)(x - 2) to illustrate this process:

(x + 3)(x - 2) = x(x) + x(-2) + 3(x) + 3(-2) = x^2 - 2x + 3x - 6 = x^2 + x - 6

As you can see, the resulting expression x^2 + x - 6 is in standard form.

Common Mistakes to Avoid When Converting Factored Form to Standard Form

When converting factored form to standard form, there are a few common mistakes to avoid:

• Forgetting to multiply the factors together using the distributive property
• Failing to combine like terms
• Not simplifying the resulting expression

To avoid these mistakes, make sure to follow the step-by-step guide outlined above and double-check your work.

Examples of Converting Factored Form to Standard Form

Let's take a look at some examples of converting factored form to standard form:

Example 1: (x + 2)(x - 5)

(x + 2)(x - 5) = x(x) + x(-5) + 2(x) + 2(-5) = x^2 - 5x + 2x - 10 = x^2 - 3x - 10

Example 2: (x - 3)(x + 2)

(x - 3)(x + 2) = x(x) + x(2) - 3(x) - 3(2) = x^2 + 2x - 3x - 6 = x^2 - x - 6

Example 3: (x + 1)(x - 4)

(x + 1)(x - 4) = x(x) + x(-4) + 1(x) + 1(-4) = x^2 - 4x + x - 4 = x^2 - 3x - 4

As you can see, converting factored form to standard form is a straightforward process that involves multiplying the factors together using the distributive property and combining like terms.

Conclusion

In this article, we've explored the step-by-step process of converting factored form to standard form. By following the guide outlined above and avoiding common mistakes, you can easily convert factored form to standard form.

Remember to practice converting factored form to standard form with different examples to reinforce your understanding of the concept.

Final Thoughts

Converting factored form to standard form is an essential skill in algebra that requires practice and patience. By mastering this skill, you'll be able to solve equations and inequalities with ease.

We hope this article has provided you with a comprehensive understanding of converting factored form to standard form. If you have any questions or comments, please feel free to share them below.