Gausss Law In Differential Form Explained

Quick Links :

Gauss's Law is a fundamental principle in physics, specifically in the field of electromagnetism, that describes the behavior of electric fields and their relationship to charges. While Gauss's Law is often expressed in its integral form, it can also be formulated in a differential form, which is a more compact and powerful way of expressing the same idea. In this article, we will delve into the world of Gauss's Law in differential form, exploring its significance, derivation, and applications.

Why Gauss's Law Matters

Gauss's Law is a cornerstone of electromagnetism, a branch of physics that deals with the interactions between electrically charged particles and the electromagnetic force, one of the four fundamental forces of nature. The law is named after Carl Friedrich Gauss, a German mathematician and physicist who first formulated it in the 19th century. Gauss's Law has far-reaching implications in various fields, including electrical engineering, materials science, and particle physics. It provides a fundamental understanding of how electric fields behave and interact with charged particles, which is essential for designing and optimizing electrical systems, from simple circuits to complex devices like particle accelerators.

Differential Form of Gauss's Law

The differential form of Gauss's Law is a partial differential equation (PDE) that relates the electric field (E) to the charge density (ρ) at a given point in space. Mathematically, it is expressed as:

∇⋅E = ρ/ε₀

where ∇ is the gradient operator, E is the electric field, ρ is the charge density, and ε₀ is the electric constant (also known as the permittivity of free space).

This equation states that the divergence of the electric field (the rate at which the field "spreads out" or "diverges" from a given point) is proportional to the charge density at that point. In other words, the electric field tends to "diverge" from regions of high charge density and "converge" into regions of low charge density.

Derivation of the Differential Form

To derive the differential form of Gauss's Law, we can start with the integral form of the law, which is:

∮E⋅dA = Q/ε₀

where ∮ is the surface integral, E is the electric field, dA is the differential area element, and Q is the total charge enclosed within the surface.

Using the divergence theorem, we can convert the surface integral into a volume integral:

∫∫∫(∇⋅E)dV = Q/ε₀

Assuming that the charge density is uniform within the volume, we can rewrite the equation as:

∫∫∫(∇⋅E)dV = ∫∫∫(ρ/ε₀)dV

Since the volume element dV is arbitrary, we can equate the integrands:

∇⋅E = ρ/ε₀

This is the differential form of Gauss's Law.

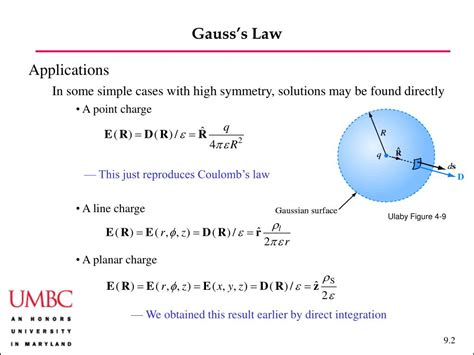
Applications of the Differential Form

The differential form of Gauss's Law has numerous applications in physics and engineering. Some of the key areas where it is used include:

• Electrostatics: The differential form is used to calculate the electric field and potential in various electrostatic problems, such as finding the field inside a conductor or the potential around a point charge.
• Electromagnetic theory: The differential form is used to derive the wave equation for electromagnetic waves and to study the behavior of electromagnetic fields in different media.
• Plasma physics: The differential form is used to study the behavior of plasmas, which are ionized gases, and to understand the dynamics of charged particles in these systems.
• Materials science: The differential form is used to study the behavior of electric fields in materials and to understand the properties of dielectrics and conductors.

Numerical Methods for Solving the Differential Form

The differential form of Gauss's Law is a PDE that requires numerical methods to solve. Some of the common numerical methods used to solve this equation include:

• Finite difference methods: These methods discretize the differential equation into a set of linear equations, which can be solved using matrix algebra.
• Finite element methods: These methods discretize the differential equation into a set of linear equations, which can be solved using matrix algebra, and also take into account the geometry of the problem.
• Method of moments: This method uses a set of basis functions to approximate the solution and solves the resulting linear system.

These numerical methods are widely used in various fields, including electrical engineering, materials science, and particle physics, to solve problems involving electric fields and charged particles.

Conclusion and Future Directions

In this article, we have explored the differential form of Gauss's Law, a fundamental principle in electromagnetism that describes the behavior of electric fields and their relationship to charges. We have derived the differential form from the integral form and discussed its applications in various fields. We have also touched upon numerical methods for solving the differential form, which are essential for solving problems involving electric fields and charged particles.

The differential form of Gauss's Law is a powerful tool for understanding and analyzing electric fields and charged particles. Its applications continue to grow, and it remains a fundamental principle in electromagnetism and related fields.

We hope this article has provided a comprehensive overview of the differential form of Gauss's Law. If you have any questions or comments, please feel free to share them with us.