Simplify Radical Form In Easy Steps

Quick Links :

Radical expressions can be intimidating, but simplifying them doesn't have to be. In fact, with a few easy steps, you can master the art of simplifying radicals and take your math skills to the next level.

Radicals are an essential part of mathematics, particularly in algebra and geometry. They help us solve equations and find the roots of numbers. However, working with radicals can be challenging, especially when they are complex or contain variables. But fear not! With a step-by-step approach, you can simplify even the most complicated radicals.

In this article, we will explore the concept of radicals, their importance in mathematics, and provide a straightforward guide on how to simplify radical expressions.

What are Radicals?

Radicals, also known as roots, are mathematical expressions that represent a number raised to a fractional exponent. They are used to simplify equations, find the roots of numbers, and solve algebraic expressions. The most common types of radicals are square roots, cube roots, and nth roots.

Types of Radicals

• Square Roots: The square root of a number is a value that, when multiplied by itself, gives the original number. For example, √16 = 4, because 4 × 4 = 16.
• Cube Roots: The cube root of a number is a value that, when multiplied by itself twice, gives the original number. For example, ∛27 = 3, because 3 × 3 × 3 = 27.
• Nth Roots: The nth root of a number is a value that, when multiplied by itself n times, gives the original number. For example, ∜16 = 2, because 2 × 2 × 2 × 2 = 16.

Why Simplify Radicals?

Simplifying radicals is essential in mathematics because it helps us to:

• Solve equations and inequalities
• Find the roots of numbers
• Simplify algebraic expressions
• Make calculations easier and more efficient

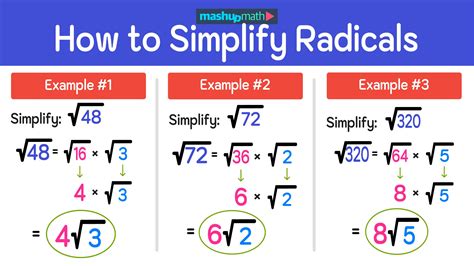
Step-by-Step Guide to Simplifying Radicals

Simplifying radicals involves a few easy steps. Here's a step-by-step guide to help you simplify radical expressions:

Step 1: Factor the Radicand

The radicand is the number inside the radical symbol. To simplify a radical, start by factoring the radicand into its prime factors.

Step 2: Identify Perfect Squares or Cubes

Look for perfect squares or cubes among the factors. A perfect square is a number that can be expressed as the product of an integer with itself, such as 16 = 4 × 4. A perfect cube is a number that can be expressed as the product of an integer with itself twice, such as 27 = 3 × 3 × 3.

Step 3: Simplify the Radical

If you find a perfect square or cube, you can simplify the radical by taking the square root or cube root of the perfect square or cube. For example, √16 = √(4 × 4) = 4.

Step 4: Rationalize the Denominator (Optional)

If the radical is in the denominator of a fraction, you may need to rationalize the denominator by multiplying the numerator and denominator by the conjugate of the denominator.

Examples of Simplifying Radicals

Here are some examples of simplifying radicals:

• √16 = √(4 × 4) = 4
• ∛27 = ∛(3 × 3 × 3) = 3
• ∜16 = ∜(2 × 2 × 2 × 2) = 2
• √(x^2 + 4) = √(x^2) + √4 = x + 2

Tips and Tricks for Simplifying Radicals

Here are some tips and tricks to help you simplify radicals:

• Always factor the radicand into its prime factors.
• Look for perfect squares or cubes among the factors.
• Simplify the radical by taking the square root or cube root of the perfect square or cube.
• Rationalize the denominator if necessary.
• Use online tools or calculators to check your answers.

Common Mistakes to Avoid

Here are some common mistakes to avoid when simplifying radicals:

• Not factoring the radicand into its prime factors.
• Not identifying perfect squares or cubes among the factors.
• Not simplifying the radical correctly.
• Not rationalizing the denominator when necessary.

Conclusion

Simplifying radicals is an essential skill in mathematics that can help you solve equations, find the roots of numbers, and simplify algebraic expressions. By following the step-by-step guide outlined in this article, you can master the art of simplifying radicals and take your math skills to the next level. Remember to always factor the radicand, identify perfect squares or cubes, simplify the radical, and rationalize the denominator if necessary.

We hope this article has been helpful in simplifying radical form in easy steps. Do you have any questions or comments about simplifying radicals? Share them with us in the comments section below!