Simplify Expressions Into Radical Form Made Easy

Quick Links :

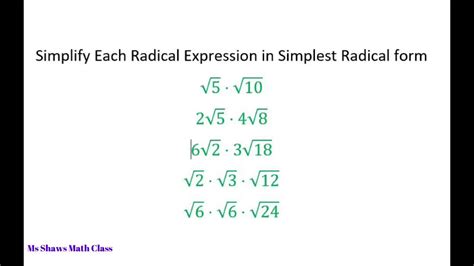
Simplifying expressions into radical form can seem daunting, but with a solid understanding of the concepts and step-by-step instructions, it can become a manageable task. Radicals, also known as roots, are used to express numbers that cannot be expressed as simple fractions. In this article, we will delve into the world of radicals and explore how to simplify expressions into radical form.

Understanding Radicals

Before we dive into simplifying expressions, it's essential to understand the basics of radicals. A radical is a symbol used to represent the root of a number. The most common type of radical is the square root, denoted by the symbol √. Other types of radicals include cube roots, fourth roots, and so on.

Types of Radicals

There are two main types of radicals: square roots and nth roots. Square roots are the most common type of radical and are used to find the number that, when multiplied by itself, gives the original value. Nth roots, on the other hand, are used to find the number that, when raised to the power of n, gives the original value.

Simplifying Expressions into Radical Form

Simplifying expressions into radical form involves rewriting the expression in a way that eliminates any radicals in the denominator. This is done to make the expression easier to work with and to avoid any potential errors.

Step-by-Step Instructions

Here are the step-by-step instructions for simplifying expressions into radical form:

1. Identify the radical: Identify the radical in the expression and determine the type of radical it is.
2. Check for any common factors: Check if there are any common factors between the numerator and the denominator that can be canceled out.
3. Rationalize the denominator: If the denominator contains a radical, multiply the numerator and denominator by the radical conjugate to rationalize the denominator.
4. Simplify the expression: Simplify the expression by combining like terms and canceling out any common factors.

Examples of Simplifying Expressions into Radical Form

Here are some examples of simplifying expressions into radical form:

Example 1: Simplifying a Square Root Expression

Simplify the expression: √(16/9)

To simplify this expression, we can start by identifying the square root and checking for any common factors. In this case, the numerator and denominator have a common factor of 4, which can be canceled out.

√(16/9) = √(4 × 4 / 9) = √(4) × √(4/9) = 2 × √(1/9) = 2/3

Example 2: Simplifying an Nth Root Expression

Simplify the expression: ∛(27/8)

To simplify this expression, we can start by identifying the nth root and checking for any common factors. In this case, the numerator and denominator have a common factor of 1, which cannot be canceled out.

∛(27/8) = ∛(3 × 3 × 3 / 8) = ∛(3) × ∛(3) × ∛(3/8) = 3 × ∛(1/8) = 3/2

Benefits of Simplifying Expressions into Radical Form

Simplifying expressions into radical form has several benefits, including:

Improved Readability

Simplifying expressions into radical form can make the expression easier to read and understand. By eliminating any radicals in the denominator, the expression becomes more manageable and less prone to errors.

Increased Accuracy

Simplifying expressions into radical form can also improve the accuracy of calculations. By avoiding any potential errors caused by radicals in the denominator, the expression becomes more reliable and trustworthy.

Common Mistakes to Avoid

When simplifying expressions into radical form, there are several common mistakes to avoid, including:

Forgetting to Rationalize the Denominator

One of the most common mistakes when simplifying expressions into radical form is forgetting to rationalize the denominator. This can lead to incorrect calculations and errors.

Not Checking for Common Factors

Another common mistake is not checking for common factors between the numerator and denominator. This can lead to oversimplification of the expression and errors.

Conclusion

Simplifying expressions into radical form is a crucial skill in mathematics and can seem daunting at first. However, with practice and a solid understanding of the concepts, it can become a manageable task. By following the step-by-step instructions and avoiding common mistakes, you can simplify expressions into radical form with ease.