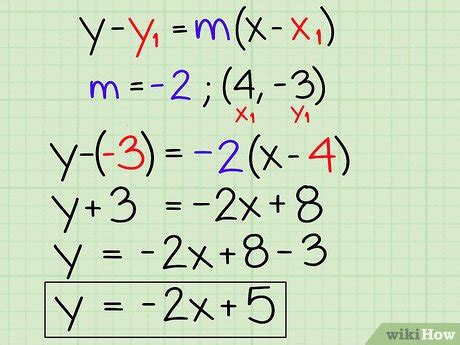Slope Intercept Form Made Easy

Quick Links :

Slope intercept form is one of the most commonly used forms of linear equations in mathematics. It is a way of expressing a linear equation in the form y = mx + b, where m is the slope of the line and b is the y-intercept. Understanding slope intercept form is essential for solving problems in algebra, geometry, and other branches of mathematics.

The concept of slope intercept form is fundamental to mathematics, and it has numerous applications in real-life scenarios. For instance, it is used in physics to describe the motion of objects, in engineering to design systems, and in economics to model economic systems. Despite its importance, many students struggle to grasp the concept of slope intercept form, which can lead to difficulties in solving problems and understanding mathematical concepts.

However, with the right approach and resources, learning slope intercept form can be made easy and enjoyable. In this article, we will delve into the world of slope intercept form, explore its definition, benefits, and applications, and provide practical examples and tips to help you master this essential mathematical concept.

What is Slope Intercept Form?

Slope intercept form is a way of expressing a linear equation in the form y = mx + b, where:

• y is the dependent variable (the variable being measured or observed)
• m is the slope of the line (a measure of how steep the line is)
• x is the independent variable (the variable being manipulated or changed)
• b is the y-intercept (the point where the line crosses the y-axis)

The slope intercept form is also known as the "slope-y-intercept form" or "point-slope form". It is a powerful tool for solving linear equations and graphing lines.

Benefits of Slope Intercept Form

Slope intercept form has several benefits that make it a popular choice among mathematicians and scientists. Some of the benefits include:

• Easy to understand and use: Slope intercept form is a simple and intuitive way of expressing linear equations.
• Versatile: Slope intercept form can be used to solve a wide range of problems, from simple algebra to complex calculus.
• Powerful: Slope intercept form can be used to model real-world phenomena, such as population growth, financial transactions, and physical systems.

How to Convert to Slope Intercept Form

Converting to slope intercept form is a straightforward process that involves rearranging the equation to isolate y. Here are the steps:

1. Write the equation in standard form (ax + by = c).
2. Subtract b from both sides of the equation to isolate y.
3. Divide both sides of the equation by a to solve for y.
4. Write the equation in slope intercept form (y = mx + b).

Examples of Converting to Slope Intercept Form

Here are a few examples of converting to slope intercept form:

• Example 1: Convert the equation 2x + 3y = 5 to slope intercept form. Solution: Subtract 2x from both sides to get 3y = -2x + 5. Then, divide both sides by 3 to get y = (-2/3)x + 5/3.
• Example 2: Convert the equation x - 2y = 3 to slope intercept form. Solution: Add 2y to both sides to get x = 2y + 3. Then, subtract 2y from both sides to get x - 2y = 3. Finally, divide both sides by -2 to get y = (-1/2)x + 3/2.

Applications of Slope Intercept Form

Slope intercept form has numerous applications in real-life scenarios. Here are a few examples:

• Physics: Slope intercept form is used to describe the motion of objects, including the trajectory of projectiles and the motion of particles.
• Engineering: Slope intercept form is used to design systems, including bridges, buildings, and electronic circuits.
• Economics: Slope intercept form is used to model economic systems, including supply and demand curves.

Real-World Examples of Slope Intercept Form

Here are a few real-world examples of slope intercept form:

• Example 1: A company produces a product that sells for $10 per unit. The cost of producing x units is given by the equation C(x) = 2x + 500. Find the revenue and profit functions using slope intercept form. Solution: The revenue function is given by R(x) = 10x, which can be written in slope intercept form as R(x) = 10x + 0. The profit function is given by P(x) = R(x) - C(x) = 10x - (2x + 500) = 8x - 500.
• Example 2: A car rental company charges a base fee of $20 plus an additional $0.25 per mile driven. Find the cost function using slope intercept form. Solution: The cost function is given by C(x) = 20 + 0.25x, which can be written in slope intercept form as C(x) = 0.25x + 20.

Common Mistakes to Avoid

When working with slope intercept form, there are several common mistakes to avoid. Here are a few:

• Forgetting to isolate y: Make sure to isolate y by subtracting b from both sides of the equation.
• Forgetting to divide by a: Make sure to divide both sides of the equation by a to solve for y.
• Writing the equation in the wrong form: Make sure to write the equation in slope intercept form (y = mx + b).

Tips for Mastering Slope Intercept Form

Here are a few tips for mastering slope intercept form:

• Practice, practice, practice: The more you practice, the more comfortable you will become with slope intercept form.
• Use online resources: There are many online resources available to help you learn slope intercept form, including video tutorials and practice exercises.
• Watch video tutorials: Video tutorials can help you understand the concept of slope intercept form and how to apply it to real-world problems.

We hope this article has helped you understand the concept of slope intercept form and how to apply it to real-world problems. Remember to practice regularly and use online resources to help you master this essential mathematical concept.

If you have any questions or comments, please feel free to ask. We would love to hear from you!