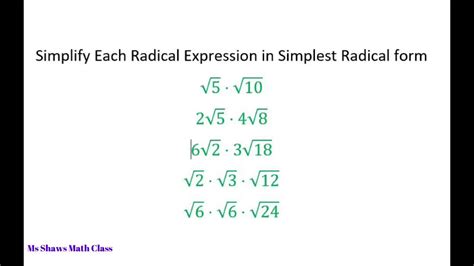
Simplifying 18 To Its Radical Form Made Easy

Quick Links :

Simplifying radicals is a fundamental concept in mathematics, particularly in algebra and geometry. Radicals, also known as roots, are mathematical operations that involve finding a value that, when raised to a specific power, gives a specified number. Simplifying radicals to their radical form is an essential skill that can be a bit challenging, but with the right approach, it can be made easy.

In this article, we will delve into the world of radicals, focusing on simplifying the radical form of 18. We will explore the concept of radicals, their importance, and provide a step-by-step guide on how to simplify the radical form of 18. By the end of this article, you will have a thorough understanding of radicals and be able to simplify them with ease.

What are Radicals?

Radicals are mathematical operations that involve finding a value that, when raised to a specific power, gives a specified number. The most common type of radical is the square root, which is denoted by the symbol √. The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 16 is 4, because 4 multiplied by 4 equals 16.

Radicals are used in various mathematical operations, such as algebra, geometry, and calculus. They are essential in solving equations, finding the area and perimeter of shapes, and calculating distances.

Why Simplify Radicals?

Simplifying radicals is crucial in mathematics because it helps to:

• Reduce complex expressions to simpler forms
• Make calculations easier and more manageable
• Identify equivalent expressions
• Solve equations and inequalities
• Graph functions and relations

Simplifying radicals can also help to avoid errors and misunderstandings in mathematical calculations.

Simplifying the Radical Form of 18

Now, let's focus on simplifying the radical form of 18. To simplify the radical form of 18, we need to find the prime factorization of 18.

The prime factorization of 18 is:

18 = 2 × 3 × 3

Using the prime factorization, we can rewrite the radical form of 18 as:

√18 = √(2 × 3 × 3)

We can simplify the radical form further by separating the prime factors into two groups:

√18 = √(2 × 3²)

Now, we can simplify the radical form even further by taking the square root of the perfect square:

√18 = √(2) × √(3²)

Since the square root of 3² is 3, we can simplify the radical form to:

√18 = √2 × 3

Therefore, the simplified radical form of 18 is √2 × 3.

Step-by-Step Guide to Simplifying Radicals

Here's a step-by-step guide to simplifying radicals:

1. Find the prime factorization of the number inside the radical.
2. Separate the prime factors into two groups: one group with perfect squares and another group with non-perfect squares.
3. Take the square root of the perfect squares.
4. Multiply the results from step 3 with the non-perfect squares.
5. Simplify the result further, if possible.

By following these steps, you can simplify radicals to their radical form with ease.

Conclusion

Simplifying radicals to their radical form is an essential skill in mathematics. By understanding the concept of radicals and following the step-by-step guide, you can simplify radicals with ease. Remember to find the prime factorization of the number inside the radical, separate the prime factors into two groups, take the square root of the perfect squares, and multiply the results with the non-perfect squares.

We hope this article has helped you to simplify the radical form of 18 and has given you a better understanding of radicals. If you have any questions or comments, please feel free to share them below.