3 Ways To Find X Intercepts In Standard Form

Quick Links :

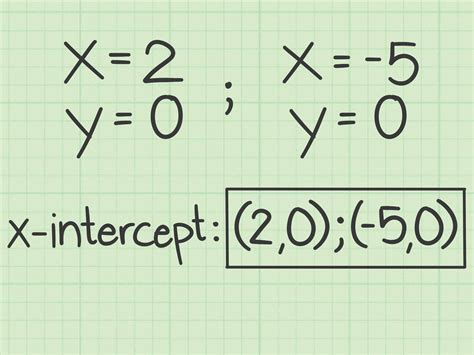
Finding the x-intercepts of a line or curve is a fundamental concept in algebra and geometry. The x-intercept is the point where the graph of an equation crosses the x-axis, and it plays a crucial role in understanding the behavior of functions. In this article, we will explore three ways to find x-intercepts in standard form, providing you with a comprehensive understanding of this essential math concept.

What are X Intercepts?

Before we dive into the methods for finding x-intercepts, it's essential to understand what they are. The x-intercept is the point where the graph of an equation crosses the x-axis. It's the value of x when y is equal to zero. In other words, the x-intercept is the solution to the equation when y = 0.

Why are X Intercepts Important?

X-intercepts are crucial in various mathematical and real-world applications. They help us understand the behavior of functions, including the maximum and minimum values, and the intervals where the function is increasing or decreasing. In addition, x-intercepts are used to solve equations, graph functions, and model real-world problems.

Method 1: Factoring

Factoring is a popular method for finding x-intercepts, especially when dealing with quadratic equations. The idea is to factor the equation into its simplest form, making it easier to identify the x-intercepts.

For example, consider the quadratic equation:

x^2 + 5x + 6 = 0

We can factor this equation as:

(x + 3)(x + 2) = 0

This tells us that the x-intercepts are x = -3 and x = -2.

Step-by-Step Factoring

To factor an equation, follow these steps:

1. Write the equation in standard form (ax^2 + bx + c = 0).
2. Look for two numbers whose product is ac and whose sum is b.
3. Write the equation as the product of two binomials.
4. Set each binomial equal to zero and solve for x.

Method 2: Graphing

Graphing is a visual method for finding x-intercepts. By plotting the graph of the equation, we can identify the points where the graph crosses the x-axis.

For example, consider the equation:

y = x^2 - 4x - 3

We can graph this equation using a graphing calculator or software. The graph shows that the x-intercepts are x = -1 and x = 3.

Step-by-Step Graphing

To graph an equation, follow these steps:

1. Write the equation in standard form (ax^2 + bx + c = 0).
2. Plot the graph using a graphing calculator or software.
3. Identify the points where the graph crosses the x-axis.
4. Read the x-coordinates of these points to find the x-intercepts.

Method 3: Quadratic Formula

The quadratic formula is a powerful method for finding x-intercepts, especially when dealing with quadratic equations that cannot be factored.

The quadratic formula is:

x = (-b ± √(b^2 - 4ac)) / 2a

For example, consider the quadratic equation:

x^2 + 2x + 1 = 0

We can use the quadratic formula to find the x-intercepts:

x = (-2 ± √(2^2 - 4(1)(1))) / 2(1) x = (-2 ± √(4 - 4)) / 2 x = (-2 ± √0) / 2 x = -1

This tells us that the x-intercept is x = -1.

Step-by-Step Quadratic Formula

To use the quadratic formula, follow these steps:

1. Write the equation in standard form (ax^2 + bx + c = 0).
2. Identify the values of a, b, and c.
3. Plug these values into the quadratic formula.
4. Simplify the expression to find the x-intercepts.

We hope this article has provided you with a comprehensive understanding of finding x-intercepts in standard form. Whether you use factoring, graphing, or the quadratic formula, you now have the tools to tackle this essential math concept. So, go ahead and practice finding x-intercepts – your math skills will thank you!