Simplify Radicals In Simplest Form Made Easy

Quick Links :

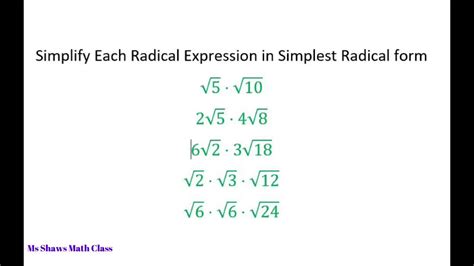
Radicals, also known as roots, are a fundamental concept in mathematics, particularly in algebra and geometry. However, working with radicals can be intimidating, especially when it comes to simplifying them. In this article, we will explore the concept of simplifying radicals in their simplest form, making it easier for you to understand and work with.

Radicals are mathematical expressions that contain a root symbol (√), indicating the extraction of a root from a number. For example, √4 represents the square root of 4, which equals 2. However, not all radicals can be simplified to a whole number. Some may result in decimal or irrational numbers. Simplifying radicals involves expressing them in their most basic form, without any unnecessary complexity.

Simplifying radicals requires a solid understanding of the properties of radicals and the rules that govern their behavior. In this article, we will delve into the world of radicals, exploring the key concepts and techniques necessary to simplify them. We will also provide numerous examples, practical tips, and strategies to help you master the art of simplifying radicals.

Understanding Radicals and Their Properties

Before we dive into simplifying radicals, it's essential to understand the basics of radicals and their properties. A radical expression consists of a radicand (the number inside the radical symbol) and an index (the number outside the radical symbol). The index indicates the type of root being extracted, such as square root (√), cube root (∛), or nth root (∜).

Some key properties of radicals include:

• The product rule: √(ab) = √a × √b
• The quotient rule: √(a/b) = √a / √b
• The power rule: (√a)^n = a^(n/2)

Understanding these properties is crucial for simplifying radicals.

Types of Radicals

Radicals can be classified into two main categories: simple radicals and complex radicals. Simple radicals involve a single root, whereas complex radicals involve multiple roots or a combination of roots.

Simple radicals can be further divided into:

• Square roots (√)
• Cube roots (∛)
• nth roots (∜)

Complex radicals, on the other hand, involve:

• Multiple roots: √(a × b) or √(a + b)
• Nested roots: √(√a) or ∛(∛a)

Simplifying Simple Radicals

Simplifying simple radicals involves expressing the radicand in a form that allows us to easily extract the root. Here are some steps to simplify simple radicals:

1. Factor the radicand: Break down the radicand into its prime factors.
2. Extract perfect squares or cubes: Identify perfect squares or cubes within the radicand and extract them.
3. Simplify the radical: Combine the extracted roots and simplify the expression.

Examples:

• √16 = √(4 × 4) = 4
• ∛27 = ∛(3 × 3 × 3) = 3

Simplifying Complex Radicals

Simplifying complex radicals involves breaking down the expression into simpler components and then simplifying each part.

1. Simplify the inner radical: Simplify the innermost radical expression, if possible.
2. Simplify the outer radical: Simplify the outer radical expression using the simplified inner radical.
3. Combine the results: Combine the simplified expressions to obtain the final result.

Examples:

• √(2 + 3) = √2 + √3
• ∛(2 × 3) = ∛2 × ∛3

Practical Tips and Strategies

Here are some practical tips and strategies to help you simplify radicals:

• Factor, factor, factor: Factorizing the radicand is key to simplifying radicals.
• Use the properties of radicals: Apply the product, quotient, and power rules to simplify radicals.
• Simplify complex radicals step-by-step: Break down complex radicals into simpler components and simplify each part.
• Practice, practice, practice: The more you practice simplifying radicals, the more comfortable you'll become.

Common Mistakes to Avoid

When simplifying radicals, it's essential to avoid common mistakes that can lead to incorrect results. Here are some common mistakes to watch out for:

• Incorrectly simplifying complex radicals: Failing to simplify the inner radical or incorrectly combining the simplified expressions.
• Ignoring the index: Forgetting to include the index or incorrectly applying it can lead to incorrect results.
• Not factorizing the radicand: Failing to factorize the radicand can make it difficult to simplify the radical.

Conclusion

Simplifying radicals may seem intimidating at first, but with practice and patience, you can master the techniques and strategies necessary to simplify even the most complex radicals. Remember to factor the radicand, apply the properties of radicals, and simplify complex radicals step-by-step. By following these tips and strategies, you'll be able to simplify radicals with ease.

We hope this article has provided you with a comprehensive understanding of simplifying radicals in their simplest form. If you have any questions or need further clarification, please don't hesitate to ask. Share your thoughts and comments below, and don't forget to share this article with your friends and colleagues who may benefit from it.

FAQ Section: