Point Slope Form Calculator For Perpendicular Lines Made Easy

Quick Links :

The world of mathematics, where lines and slopes reign supreme. Among the various concepts that govern the behavior of lines, perpendicular lines hold a special place. But, have you ever found yourself struggling to calculate the equation of a line perpendicular to another? Fear not, dear reader, for we're about to make point slope form calculator for perpendicular lines a breeze.

In this article, we'll delve into the world of perpendicular lines, explore the concept of point slope form, and provide a step-by-step guide on how to use a point slope form calculator to find the equation of a line perpendicular to another. Buckle up, and let's get started!

What are Perpendicular Lines?

Perpendicular lines are two lines that intersect at a right angle (90 degrees). They are a fundamental concept in geometry and are used extensively in various fields, including architecture, engineering, and design. Perpendicular lines have several key properties, including:

• They intersect at a single point (the point of intersection)
• The angle between them is 90 degrees
• The slopes of perpendicular lines are negative reciprocals of each other

What is Point Slope Form?

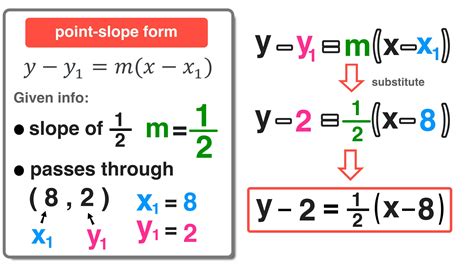
Point slope form is a way of expressing the equation of a line in a two-dimensional plane. It's a powerful tool for finding the equation of a line, especially when you're given the slope and a point on the line. The point slope form of a line is given by:

y - y1 = m(x - x1)

where:

• (x1, y1) is a point on the line
• m is the slope of the line

How to Use a Point Slope Form Calculator for Perpendicular Lines

Now that we've covered the basics, let's dive into how to use a point slope form calculator to find the equation of a line perpendicular to another. Here's a step-by-step guide:

1. Find the slope of the original line: Use the point slope form to find the slope of the original line. If you're given two points on the line, you can use the slope formula to find the slope.
2. Find the negative reciprocal of the slope: To find the slope of the perpendicular line, take the negative reciprocal of the original slope. For example, if the original slope is 2, the slope of the perpendicular line is -1/2.
3. Choose a point on the perpendicular line: Select a point on the perpendicular line. This can be any point that lies on the line.
4. Use the point slope form to find the equation: Plug the slope and point into the point slope form equation to find the equation of the perpendicular line.

Example 1

Find the equation of a line perpendicular to the line 2x + 3y = 7, passing through the point (4, 5).

First, find the slope of the original line using the point slope form:

y - 5 = (-2/3)(x - 4)

The slope of the original line is -2/3. To find the slope of the perpendicular line, take the negative reciprocal:

m = 3/2

Now, use the point slope form to find the equation of the perpendicular line:

y - 5 = (3/2)(x - 4)

Simplifying, we get:

y = (3/2)x - 1

Example 2

Find the equation of a line perpendicular to the line x - 2y = 3, passing through the point (-1, 2).

First, find the slope of the original line using the point slope form:

y - 2 = (1/2)(x + 1)

The slope of the original line is 1/2. To find the slope of the perpendicular line, take the negative reciprocal:

m = -2

Now, use the point slope form to find the equation of the perpendicular line:

y - 2 = (-2)(x + 1)

Simplifying, we get:

y = -2x - 4

Benefits of Using a Point Slope Form Calculator

Using a point slope form calculator to find the equation of a line perpendicular to another offers several benefits, including:

• Saves time: With a point slope form calculator, you can quickly find the equation of a perpendicular line, saving you time and effort.
• Reduces errors: The calculator eliminates the risk of human error, ensuring that your calculations are accurate and reliable.
• Improves understanding: By using a point slope form calculator, you can gain a deeper understanding of the concept of perpendicular lines and how to apply it to real-world problems.

Real-World Applications of Perpendicular Lines

Perpendicular lines have numerous real-world applications, including:

• Architecture: Perpendicular lines are used extensively in building design, ensuring that walls, floors, and ceilings are perfectly aligned.
• Engineering: Perpendicular lines are used in the design of bridges, roads, and other infrastructure, ensuring that structures are stable and secure.
• Design: Perpendicular lines are used in graphic design, ensuring that text and images are perfectly aligned and visually appealing.

Conclusion

In conclusion, finding the equation of a line perpendicular to another is a crucial skill in mathematics, with numerous real-world applications. By using a point slope form calculator, you can quickly and accurately find the equation of a perpendicular line, saving you time and effort. Remember to follow the steps outlined in this article, and practice using the calculator to become proficient in finding the equation of perpendicular lines.

We hope this article has helped you understand how to use a point slope form calculator to find the equation of a line perpendicular to another. If you have any further questions or need clarification on any of the concepts, please don't hesitate to ask. Happy calculating!