Understanding Standard Form with Two Points

Are you struggling to find the standard form of a line when given two points? You're not alone. Many students and professionals in mathematics, physics, and engineering often face difficulties when trying to find the standard form of a line, especially when dealing with two points. However, with the right approach and understanding of the concept, finding the standard form with two points can be a breeze.
In this article, we will delve into the world of standard form, exploring its definition, importance, and steps to find it using two points. We will also provide practical examples and illustrations to make the concept more accessible and easier to grasp.
What is Standard Form?

Standard form, also known as the slope-intercept form, is a way of expressing a linear equation in the form of y = mx + b, where m is the slope of the line and b is the y-intercept. The standard form is useful for finding the equation of a line, determining the slope and y-intercept, and solving linear equations.
Why is Standard Form Important?
Standard form is crucial in mathematics and science, as it provides a simple and concise way to express linear relationships between variables. It is widely used in various fields, including physics, engineering, economics, and computer science.
Some of the key benefits of standard form include:
- Easy to graph and visualize
- Simple to solve linear equations
- Convenient for finding slope and y-intercept
- Useful for modeling real-world phenomena
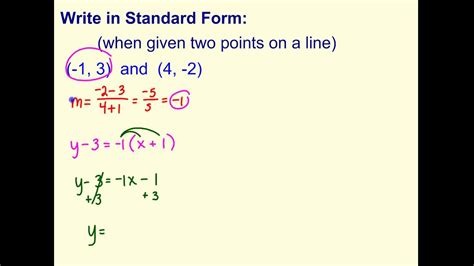
How to Find Standard Form with Two Points

Now that we have understood the concept of standard form, let's move on to finding the standard form with two points. Here are the steps:
- Identify the two points: Let's say we have two points (x1, y1) and (x2, y2).
- Calculate the slope: Use the formula m = (y2 - y1) / (x2 - x1) to find the slope.
- Find the y-intercept: Use the formula b = y1 - m * x1 to find the y-intercept.
- Write the equation: Combine the slope and y-intercept to write the equation in standard form (y = mx + b).
Example 1: Finding Standard Form with Two Points
Suppose we have two points (2, 3) and (4, 5). Let's find the standard form of the line passing through these points.
- Calculate the slope: m = (5 - 3) / (4 - 2) = 2 / 2 = 1
- Find the y-intercept: b = 3 - 1 * 2 = 1
- Write the equation: y = x + 1
Practical Applications of Standard Form

Standard form has numerous practical applications in various fields, including:
- Physics: Modeling the motion of objects, force, and energy
- Engineering: Designing electronic circuits, mechanical systems, and structures
- Economics: Analyzing supply and demand curves, cost-benefit analysis
- Computer Science: Developing algorithms, modeling data, and creating simulations
Common Mistakes to Avoid

When finding the standard form with two points, it's essential to avoid common mistakes, including:
- Incorrectly calculating the slope or y-intercept
- Forgetting to write the equation in standard form
- Misinterpreting the results or applying the wrong formula
Conclusion and Final Thoughts
Finding the standard form with two points is a fundamental concept in mathematics and science. By understanding the steps and practical applications, you can master this skill and solve a wide range of problems. Remember to avoid common mistakes and practice regularly to become proficient.
We hope this article has helped you understand the concept of standard form and its importance in various fields. If you have any questions or comments, please share them below.
What is the difference between standard form and slope-intercept form?
+Standard form and slope-intercept form are essentially the same thing. Both refer to the equation of a line in the form of y = mx + b, where m is the slope and b is the y-intercept.
Can I use standard form to find the equation of a line with three or more points?
+Yes, you can use standard form to find the equation of a line with three or more points. However, you will need to use a different method, such as finding the slope and y-intercept using two points, and then using the third point to verify the equation.
What are some real-world applications of standard form?
+Standard form has numerous real-world applications, including physics, engineering, economics, and computer science. It is used to model real-world phenomena, such as the motion of objects, force, and energy, and to solve problems in fields like electronics, mechanics, and finance.