Radicals can be a challenging topic in mathematics, especially when it comes to simplifying them. However, with the right techniques and strategies, simplifying radicals can become a breeze. In this article, we will explore five ways to simplify radicals, making it easier for you to tackle even the most complex radical expressions.
Understanding Radicals

Before we dive into the ways to simplify radicals, let's first understand what radicals are. A radical is a mathematical expression that represents the root of a number. The most common radical is the square root, which is denoted by the symbol √. Other types of radicals include cube roots, fourth roots, and so on.
Method 1: Simplifying Radicals Using Perfect Squares

One of the easiest ways to simplify radicals is by using perfect squares. A perfect square is a number that can be expressed as the square of an integer. For example, 4, 9, 16, and 25 are all perfect squares because they can be expressed as 2^2, 3^2, 4^2, and 5^2, respectively.
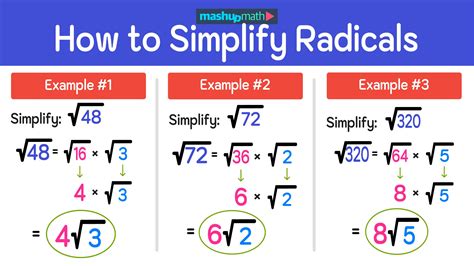
To simplify a radical using perfect squares, look for the largest perfect square that divides the number inside the radical. For example, to simplify √48, we can look for the largest perfect square that divides 48. In this case, it is 16, which is a perfect square because 16 = 4^2.
√48 = √(16 × 3) = √16 × √3 = 4√3
Example Problems
- Simplify √24
- Simplify √75
Method 2: Simplifying Radicals Using Prime Factorization

Another way to simplify radicals is by using prime factorization. Prime factorization involves breaking down a number into its prime factors. For example, the prime factorization of 48 is 2^4 × 3.
To simplify a radical using prime factorization, break down the number inside the radical into its prime factors. Then, look for pairs of the same prime factor. For each pair, you can take one of the factors outside the radical.
For example, to simplify √48, we can break down 48 into its prime factors:
48 = 2^4 × 3
Then, we can look for pairs of the same prime factor:
√48 = √(2^4 × 3) = √(2^2 × 2^2 × 3) = 2^2√3 = 4√3
Example Problems
- Simplify √80
- Simplify √125
Method 3: Simplifying Radicals Using the Quotient Property

The quotient property of radicals states that √(a/b) = √a / √b. This property can be used to simplify radicals by dividing the number inside the radical by the largest perfect square that divides it.
For example, to simplify √(24/9), we can use the quotient property:
√(24/9) = √24 / √9 = √(16 × 1.5) / 3 = 4√1.5 / 3 = 4√(3/2) / 3 = 4/3√3/2 = 2√2/3
Example Problems
- Simplify √(16/4)
- Simplify √(25/9)
Method 4: Simplifying Radicals Using the Product Property

The product property of radicals states that √(ab) = √a × √b. This property can be used to simplify radicals by multiplying the number inside the radical by the largest perfect square that divides it.
For example, to simplify √(12 × 2), we can use the product property:
√(12 × 2) = √12 × √2 = √(4 × 3) × √2 = 2√3 × √2 = 2√6
Example Problems
- Simplify √(8 × 5)
- Simplify √(20 × 3)
Method 5: Simplifying Radicals Using Rational Exponents

Rational exponents are exponents that are fractions. For example, 1/2, 1/3, and 2/3 are all rational exponents. Rational exponents can be used to simplify radicals by expressing the radical as a rational exponent.
For example, to simplify √(8^1/3), we can express the radical as a rational exponent:
√(8^1/3) = 8^1/6 = (2^3)^1/6 = 2^1/2 = √2
Example Problems
- Simplify √(27^1/3)
- Simplify √(16^1/4)
What is the difference between a perfect square and a perfect cube?
+A perfect square is a number that can be expressed as the square of an integer, while a perfect cube is a number that can be expressed as the cube of an integer.
How do I simplify a radical using prime factorization?
+To simplify a radical using prime factorization, break down the number inside the radical into its prime factors. Then, look for pairs of the same prime factor. For each pair, you can take one of the factors outside the radical.
What is the quotient property of radicals?
+The quotient property of radicals states that √(a/b) = √a / √b.
We hope this article has helped you understand the different ways to simplify radicals. Whether you're using perfect squares, prime factorization, the quotient property, the product property, or rational exponents, simplifying radicals can be a breeze. Remember to practice regularly to become more proficient in simplifying radicals.
If you have any questions or need further clarification, please don't hesitate to ask. Share your thoughts and experiences with simplifying radicals in the comments section below.