4 Steps To Convert Vertex Form To Factored Form

Quick Links :

Hide

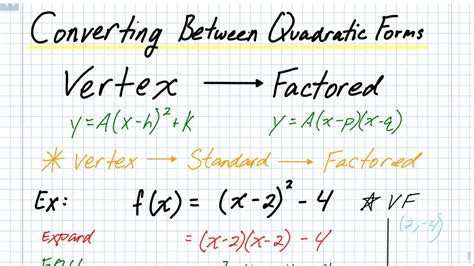
Converting a quadratic equation from vertex form to factored form is an essential skill in algebra. The vertex form of a quadratic equation is in the form y = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. On the other hand, the factored form is in the form y = a(x - r)(x - s), where r and s are the roots of the equation. In this article, we will discuss the steps to convert a quadratic equation from vertex form to factored form.

Understanding the Vertex Form

Before we dive into the conversion process, let's first understand the vertex form of a quadratic equation. The vertex form is useful for graphing and identifying the vertex of a parabola. However, it's not always easy to identify the roots of the equation from the vertex form.

Identifying the Vertex

The vertex of a parabola in the form `y = a(x - h)^2 + k` is `(h, k)`. The value of `h` represents the x-coordinate of the vertex, while `k` represents the y-coordinate. To convert the vertex form to factored form, we need to identify the roots of the equation, which are the x-intercepts of the parabola.

Step 1: Identify the Roots of the Equation

The roots of a quadratic equation in the form `y = a(x - h)^2 + k` can be found by setting `y` to 0 and solving for `x`. This will give us the x-intercepts of the parabola, which are the roots of the equation. For example, if we have the equation `y = (x - 2)^2 - 3`, we can set `y` to 0 and solve for `x` to find the roots.

Solving for Roots

To solve for the roots, we can use the quadratic formula: `x = (-b ± √(b^2 - 4ac)) / 2a`. In this case, `a` is the coefficient of the squared term, `b` is the coefficient of the linear term, and `c` is the constant term. Since our equation is in the form `y = a(x - h)^2 + k`, we can rewrite it as `y = a(x^2 - 2hx + h^2) + k`. By comparing this with the standard form `ax^2 + bx + c`, we can identify `a`, `b`, and `c`.

Step 2: Rewrite the Equation in Standard Form

Once we have identified the roots of the equation, we can rewrite the equation in standard form `ax^2 + bx + c`. This will allow us to factor the equation and find the factored form. For example, if we have the equation `y = (x - 2)^2 - 3`, we can rewrite it as `y = x^2 - 4x + 4 - 3` or `y = x^2 - 4x + 1`.

Identifying a, b, and c

By comparing the rewritten equation with the standard form `ax^2 + bx + c`, we can identify `a`, `b`, and `c`. In this case, `a` is 1, `b` is -4, and `c` is 1.

Step 3: Factor the Equation

Now that we have rewritten the equation in standard form, we can factor the equation to find the factored form. The factored form of a quadratic equation is in the form `y = a(x - r)(x - s)`, where `r` and `s` are the roots of the equation. We can use the roots we found in Step 1 to factor the equation.

Factoring the Equation

To factor the equation, we can use the fact that `x^2 - 4x + 1` can be factored as `(x - 2 + √3)(x - 2 - √3)`. Therefore, the factored form of the equation is `y = (x - 2 + √3)(x - 2 - √3) - 3`.

Step 4: Simplify the Factored Form

Finally, we can simplify the factored form by combining like terms. In this case, we can simplify the factored form to `y = (x - 2 + √3)(x - 2 - √3) - 3`.

Simplified Factored Form

The simplified factored form of the equation is `y = (x - 2 + √3)(x - 2 - √3) - 3`. This is the final answer.

We hope this article has helped you understand the steps to convert a quadratic equation from vertex form to factored form. With practice and patience, you can master this skill and become proficient in algebra.