Graphing Quadratic Functions In Standard Form Made Easy

Quick Links :

Quadratic functions are a fundamental concept in algebra, and graphing them can be a challenging task for many students. However, with a clear understanding of the standard form of quadratic functions, graphing them can become a much easier process. In this article, we will explore the world of quadratic functions in standard form, and provide a step-by-step guide on how to graph them with ease.

Understanding Quadratic Functions in Standard Form

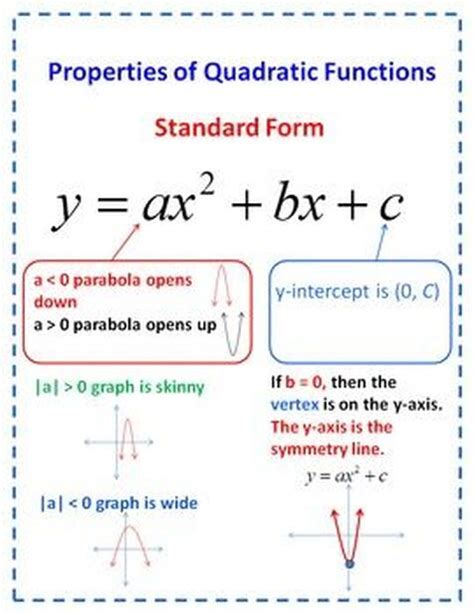
A quadratic function in standard form is written in the form of f(x) = ax^2 + bx + c, where a, b, and c are constants, and x is the variable. The standard form of a quadratic function provides valuable information about the graph of the function, including its shape, vertex, and axis of symmetry.

To graph a quadratic function in standard form, we need to identify the values of a, b, and c, and use them to determine the vertex, axis of symmetry, and x-intercepts of the graph.

Identifying the Vertex, Axis of Symmetry, and X-Intercepts

The vertex of a quadratic function in standard form is given by the formula (h, k), where h = -b/2a and k = f(h). The axis of symmetry is a vertical line that passes through the vertex, and is given by the equation x = h.

To find the x-intercepts of the graph, we need to solve the equation f(x) = 0. This can be done by factoring the quadratic expression, or by using the quadratic formula.

Example: Graphing a Quadratic Function in Standard Form

Let's consider the quadratic function f(x) = x^2 + 4x + 4. To graph this function, we need to identify the values of a, b, and c, and use them to determine the vertex, axis of symmetry, and x-intercepts.

Comparing the given function with the standard form, we can see that a = 1, b = 4, and c = 4.

Using the formula for the vertex, we get h = -b/2a = -4/2(1) = -2, and k = f(h) = f(-2) = (-2)^2 + 4(-2) + 4 = 4 - 8 + 4 = 0.

Therefore, the vertex of the graph is (-2, 0).

The axis of symmetry is a vertical line that passes through the vertex, and is given by the equation x = -2.

To find the x-intercepts, we need to solve the equation f(x) = 0. This can be done by factoring the quadratic expression, which gives us (x + 2)(x + 2) = 0. Therefore, the x-intercepts are x = -2.

Graphing the Quadratic Function

Now that we have identified the vertex, axis of symmetry, and x-intercepts, we can graph the quadratic function.

The graph of the quadratic function f(x) = x^2 + 4x + 4 is a parabola that opens upwards, with a vertex at (-2, 0) and an axis of symmetry at x = -2. The x-intercept is at x = -2.

Benefits of Graphing Quadratic Functions in Standard Form

Graphing quadratic functions in standard form provides several benefits, including:

• Easy identification of the vertex, axis of symmetry, and x-intercepts
• Clear understanding of the shape and direction of the graph
• Simplified process of graphing the function
• Improved accuracy in graphing the function

Common Mistakes to Avoid When Graphing Quadratic Functions

When graphing quadratic functions in standard form, there are several common mistakes to avoid, including:

• Failure to identify the values of a, b, and c correctly
• Incorrect calculation of the vertex, axis of symmetry, and x-intercepts
• Failure to graph the function accurately
• Not checking the graph for accuracy

By avoiding these common mistakes, you can ensure that you graph quadratic functions in standard form accurately and with ease.

Conclusion: Mastering the Art of Graphing Quadratic Functions

Graphing quadratic functions in standard form is a fundamental concept in algebra that requires a clear understanding of the standard form of quadratic functions. By identifying the values of a, b, and c, and using them to determine the vertex, axis of symmetry, and x-intercepts, you can graph quadratic functions with ease.

Remember to avoid common mistakes, and to check your graph for accuracy. With practice and patience, you can master the art of graphing quadratic functions in standard form.

We hope this article has helped you to understand the concept of graphing quadratic functions in standard form. If you have any questions or need further clarification, please don't hesitate to ask.