Graphing Lines In Slope Intercept Form Made Easy

Quick Links :

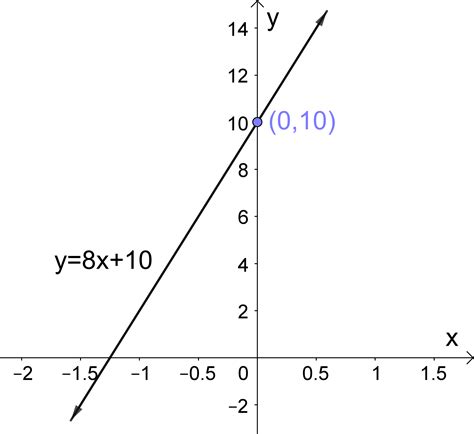
Learning to graph lines in slope-intercept form is an essential skill for any student of mathematics, particularly those studying algebra. The slope-intercept form of a linear equation, y = mx + b, where m represents the slope and b represents the y-intercept, is a powerful tool for graphing lines on the coordinate plane. In this article, we will explore the concept of slope-intercept form, provide examples and explanations, and offer practical tips for mastering this fundamental skill.

Understanding Slope-Intercept Form

The slope-intercept form of a linear equation, y = mx + b, is one of the most common and useful forms of linear equations. In this equation, m represents the slope of the line, which measures how steep the line is. The slope can be positive, negative, or zero. A positive slope indicates that the line rises from left to right, a negative slope indicates that the line falls from left to right, and a slope of zero indicates that the line is horizontal.

On the other hand, b represents the y-intercept, which is the point where the line intersects the y-axis. The y-intercept can be positive, negative, or zero, and it provides a starting point for graphing the line.

Benefits of Slope-Intercept Form

The slope-intercept form has several benefits that make it a popular choice for graphing lines:

• Easy to understand and use
• Provides a clear visual representation of the line
• Allows for quick identification of the slope and y-intercept
• Useful for graphing lines with positive, negative, or zero slopes

Graphing Lines in Slope-Intercept Form

Now that we have a solid understanding of the slope-intercept form, let's explore how to graph lines using this method. Here are the steps:

1. Identify the slope and y-intercept of the equation.
2. Plot the y-intercept on the y-axis.
3. Use the slope to determine the direction of the line.
4. Plot a second point on the line using the slope.
5. Draw a straight line through the two points.

Example: Graphing a Line with a Positive Slope

Consider the equation y = 2x + 3. In this example, the slope is 2, and the y-intercept is 3. To graph the line, we can follow the steps outlined above:

• Plot the y-intercept (0, 3) on the y-axis.
• Use the slope (2) to determine the direction of the line. Since the slope is positive, the line will rise from left to right.
• Plot a second point on the line using the slope. For example, we can use the point (1, 5).
• Draw a straight line through the two points.

Common Mistakes to Avoid

When graphing lines in slope-intercept form, there are several common mistakes to avoid:

• Incorrectly identifying the slope and y-intercept
• Plotting the y-intercept incorrectly
• Using the wrong slope to determine the direction of the line
• Failing to plot a second point on the line

Tips for Mastering Slope-Intercept Form

Here are some practical tips for mastering the slope-intercept form:

• Practice, practice, practice: The more you practice graphing lines in slope-intercept form, the more comfortable you will become with the process.
• Use online resources: There are many online resources available that can provide additional support and practice exercises.
• Watch video tutorials: Video tutorials can provide a visual explanation of the process and help to clarify any concepts that you may be struggling with.

Real-World Applications of Slope-Intercept Form

The slope-intercept form has many real-world applications, including:

• Physics and engineering: The slope-intercept form is used to model the motion of objects and to design systems.
• Economics: The slope-intercept form is used to model economic systems and to make predictions about future trends.
• Computer science: The slope-intercept form is used in computer programming to model complex systems and to make predictions about future trends.

Example: Using Slope-Intercept Form in Physics

Consider a ball that is thrown upwards from the ground. The height of the ball can be modeled using the equation y = -4.9x^2 + 20x + 1, where y is the height of the ball and x is the time. By using the slope-intercept form, we can identify the slope and y-intercept of the equation and graph the line.

In conclusion, the slope-intercept form is a powerful tool for graphing lines on the coordinate plane. By understanding the concept of slope-intercept form and following the steps outlined in this article, you can become proficient in graphing lines and unlock a wide range of real-world applications.