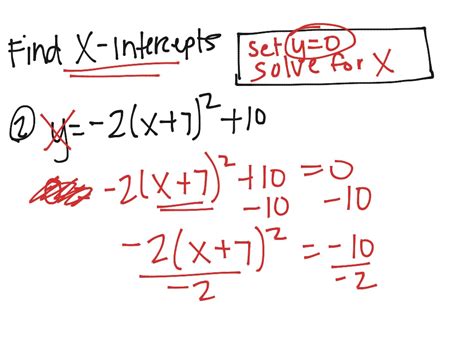3 Ways To Find X Intercepts From Vertex Form

Quick Links :

Finding the x-intercepts of a quadratic equation can be a challenging task, especially when the equation is in vertex form. However, with the right strategies and techniques, you can easily find the x-intercepts of any quadratic equation, regardless of its form. In this article, we will explore three ways to find x-intercepts from vertex form.

Understanding Vertex Form

Before we dive into the methods for finding x-intercepts, it's essential to understand what vertex form is. Vertex form is a way of expressing a quadratic equation in the form y = a(x - h)^2 + k, where (h, k) is the vertex of the parabola. This form is particularly useful for finding the vertex and axis of symmetry of a parabola.

Method 1: Using the Vertex Form Formula

One way to find the x-intercepts of a quadratic equation in vertex form is to use the formula x = h ± √(-k/a). This formula is derived from the fact that the x-intercepts of a parabola are symmetric about the axis of symmetry, which passes through the vertex.

To use this formula, simply plug in the values of a, h, and k from the vertex form equation. For example, if the equation is y = 2(x - 3)^2 + 4, the values of a, h, and k are 2, 3, and 4, respectively. Plugging these values into the formula, we get x = 3 ± √(-4/2) = 3 ± √(-2).

Step-by-Step Example

• Write down the vertex form equation: y = 2(x - 3)^2 + 4
• Identify the values of a, h, and k: a = 2, h = 3, k = 4
• Plug these values into the formula: x = 3 ± √(-4/2)
• Simplify the expression: x = 3 ± √(-2)
• Solve for x: x = 3 ± i√2

As you can see, the x-intercepts of this quadratic equation are complex numbers.

Method 2: Factoring the Quadratic Expression

Another way to find the x-intercepts of a quadratic equation in vertex form is to factor the quadratic expression. This method is particularly useful when the equation is not easily solvable using the formula.

To factor the quadratic expression, start by expanding the squared term. For example, if the equation is y = 2(x - 3)^2 + 4, expand the squared term to get y = 2(x^2 - 6x + 9) + 4.

Next, factor out the greatest common factor (GCF) of the quadratic expression. In this case, the GCF is 2. Factoring out the GCF, we get y = 2(x^2 - 6x + 9 + 2).

Now, factor the quadratic expression inside the parentheses. In this case, the expression factors to (x - 3)^2. Therefore, the factored form of the equation is y = 2(x - 3)^2 + 4.

Step-by-Step Example

• Write down the vertex form equation: y = 2(x - 3)^2 + 4
• Expand the squared term: y = 2(x^2 - 6x + 9) + 4
• Factor out the GCF: y = 2(x^2 - 6x + 9 + 2)
• Factor the quadratic expression: y = 2(x - 3)^2 + 4
• Set the expression equal to zero: 2(x - 3)^2 + 4 = 0
• Solve for x: x = 3 ± √(-2)

As you can see, the x-intercepts of this quadratic equation are the same as those found using the formula.

Method 3: Using the Quadratic Formula

A third way to find the x-intercepts of a quadratic equation in vertex form is to use the quadratic formula. This method is particularly useful when the equation is not easily solvable using the formula or factoring.

To use the quadratic formula, start by rewriting the equation in the form ax^2 + bx + c = 0. In this case, the equation is y = 2(x - 3)^2 + 4, which can be rewritten as 2x^2 - 12x + 18 = 0.

Next, plug the values of a, b, and c into the quadratic formula: x = (-b ± √(b^2 - 4ac)) / 2a. In this case, the values of a, b, and c are 2, -12, and 18, respectively.

Step-by-Step Example

• Write down the vertex form equation: y = 2(x - 3)^2 + 4
• Rewrite the equation in the form ax^2 + bx + c = 0: 2x^2 - 12x + 18 = 0
• Plug the values of a, b, and c into the quadratic formula: x = (12 ± √((-12)^2 - 4(2)(18))) / 2(2)
• Simplify the expression: x = (12 ± √(144 - 144)) / 4
• Solve for x: x = 3 ± √(-2)

As you can see, the x-intercepts of this quadratic equation are the same as those found using the formula and factoring.

Conclusion

Finding the x-intercepts of a quadratic equation in vertex form can be a challenging task, but with the right strategies and techniques, you can easily find the x-intercepts of any quadratic equation. In this article, we explored three ways to find x-intercepts from vertex form: using the vertex form formula, factoring the quadratic expression, and using the quadratic formula. Each method has its own strengths and weaknesses, and the choice of method will depend on the specific equation and your personal preference.

We hope this article has been informative and helpful. If you have any questions or comments, please don't hesitate to ask. Share this article with your friends and colleagues, and don't forget to like and subscribe for more math tutorials and tips.