5 Ways To Simplify √32

Quick Links :

Simplifying radicals can seem intimidating, but with a few simple steps, you can break down even the most complex radicals into manageable parts. In this article, we'll explore five ways to simplify √32, a radical that may seem daunting at first but can be simplified with ease.

Method 1: Prime Factorization

One of the most effective ways to simplify radicals is by using prime factorization. This method involves breaking down the number inside the radical into its prime factors. To simplify √32 using prime factorization, we'll start by identifying the prime factors of 32.

32 = 2 × 2 × 2 × 2 × 2

Now, we can rewrite √32 as:

√32 = √(2 × 2 × 2 × 2 × 2)

Since we have an even number of 2's, we can simplify the radical by taking out the perfect square:

√32 = √(2^5)

√32 = 2√(2^3)

√32 = 2√8

Method 1 Example

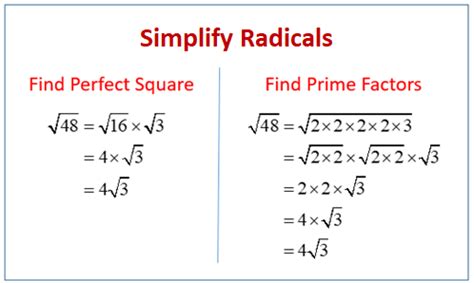
For example, let's say we want to simplify √48 using prime factorization. We'll start by identifying the prime factors of 48:

48 = 2 × 2 × 2 × 2 × 3

Now, we can rewrite √48 as:

√48 = √(2 × 2 × 2 × 2 × 3)

Since we have an even number of 2's, we can simplify the radical by taking out the perfect square:

√48 = √(2^4 × 3)

√48 = 2√(2^2 × 3)

√48 = 2√12

Method 2: Finding Perfect Squares

Another way to simplify radicals is by finding perfect squares within the number inside the radical. To simplify √32 using this method, we'll look for perfect squares that divide evenly into 32.

32 = 16 × 2

Since 16 is a perfect square, we can rewrite √32 as:

√32 = √(16 × 2)

Now, we can simplify the radical by taking out the perfect square:

√32 = √16 × √2

√32 = 4√2

Method 2 Example

For example, let's say we want to simplify √80 using the perfect squares method. We'll start by looking for perfect squares that divide evenly into 80:

80 = 16 × 5

Since 16 is a perfect square, we can rewrite √80 as:

√80 = √(16 × 5)

Now, we can simplify the radical by taking out the perfect square:

√80 = √16 × √5

√80 = 4√5

Method 3: Using the Distributive Property

The distributive property can also be used to simplify radicals. This method involves breaking down the number inside the radical into smaller parts and then simplifying each part separately. To simplify √32 using the distributive property, we'll start by breaking down 32 into smaller parts:

32 = 16 + 16

Now, we can rewrite √32 as:

√32 = √(16 + 16)

Using the distributive property, we can simplify the radical as:

√32 = √16 + √16

√32 = 4 + 4

√32 = 8

However, this method is not always accurate, and the result may not be the simplest form of the radical.

Method 3 Example

For example, let's say we want to simplify √20 using the distributive property. We'll start by breaking down 20 into smaller parts:

20 = 4 + 16

Now, we can rewrite √20 as:

√20 = √(4 + 16)

Using the distributive property, we can simplify the radical as:

√20 = √4 + √16

√20 = 2 + 4

However, this result is not the simplest form of the radical.

Method 4: Using a Calculator

In today's digital age, calculators have become an essential tool for simplifying radicals. Most calculators have a built-in radical simplification function that can simplify even the most complex radicals with ease. To simplify √32 using a calculator, simply enter the number inside the radical and press the radical simplification button.

Method 4 Example

For example, let's say we want to simplify √48 using a calculator. Simply enter the number inside the radical and press the radical simplification button:

√48 ≈ 6.93

Method 5: Using a Radical Simplification Chart

A radical simplification chart is a handy tool that can help you simplify radicals quickly and easily. This chart lists the square roots of common numbers, making it easy to simplify radicals by simply looking up the value. To simplify √32 using a radical simplification chart, simply look up the value of √32 on the chart.

Method 5 Example

For example, let's say we want to simplify √20 using a radical simplification chart. Simply look up the value of √20 on the chart:

√20 = √(4 × 5)

√20 = 2√5

In conclusion, simplifying radicals can seem intimidating, but with the right methods and tools, it can be a breeze. Whether you use prime factorization, find perfect squares, use the distributive property, a calculator, or a radical simplification chart, simplifying radicals is an essential math skill that can help you tackle even the most complex math problems.

We'd love to hear from you! What's your favorite method for simplifying radicals? Share your thoughts and experiences in the comments below. Don't forget to share this article with your friends and family who may be struggling with radicals.