Polynomials Made Easy: Standard Form Converter

Quick Links :

The world of mathematics is full of fascinating concepts, and one of the most fundamental is polynomials. Polynomials are expressions consisting of variables and coefficients combined using only addition, subtraction, and multiplication. They are used to model real-world phenomena, solve problems, and analyze data. However, working with polynomials can be challenging, especially when it comes to converting them into standard form. In this article, we will explore the concept of polynomials, their importance, and how to make them easier to work with using a standard form converter.

Polynomials are essential in various fields, including physics, engineering, economics, and computer science. They are used to describe the relationship between variables, model complex systems, and make predictions. For instance, in physics, polynomials are used to describe the motion of objects, while in economics, they are used to model the behavior of markets. However, polynomials can be complex and difficult to work with, especially when they are not in standard form.

What is Standard Form?

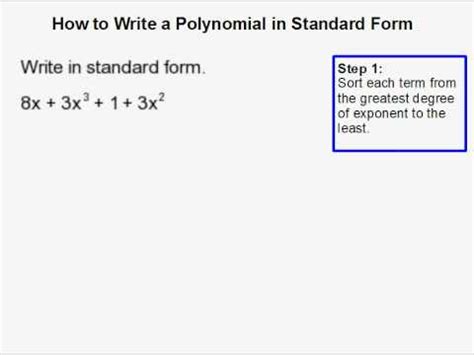
Standard form is a way of writing polynomials in a consistent and organized manner. It involves arranging the terms of the polynomial in descending order of their exponents. For example, the polynomial 3x^2 + 2x - 4 is in standard form because the terms are arranged in descending order of their exponents. Standard form makes it easier to work with polynomials, as it allows for easier comparison, addition, and subtraction.

Why is Standard Form Important?

Standard form is important because it provides a consistent and organized way of writing polynomials. This consistency makes it easier to work with polynomials, as it allows for easier comparison, addition, and subtraction. Standard form also makes it easier to identify the degree of the polynomial, which is the highest exponent of the variable. The degree of the polynomial is important because it determines the number of solutions the polynomial has.

How to Convert Polynomials to Standard Form

Converting polynomials to standard form involves arranging the terms in descending order of their exponents. Here are the steps to follow:

1. Identify the terms of the polynomial.
2. Arrange the terms in descending order of their exponents.
3. Combine like terms, if necessary.
4. Write the polynomial in standard form.

For example, consider the polynomial 2x - 3x^2 + 4. To convert this polynomial to standard form, we need to arrange the terms in descending order of their exponents. The exponent of the first term is 1, the exponent of the second term is 2, and the exponent of the third term is 0. Therefore, the polynomial in standard form is -3x^2 + 2x + 4.

Using a Standard Form Converter

Converting polynomials to standard form can be time-consuming and prone to errors. To make this process easier, we can use a standard form converter. A standard form converter is a tool that takes a polynomial as input and outputs the polynomial in standard form. This tool can save time and reduce errors, making it easier to work with polynomials.

Benefits of Using a Standard Form Converter

Using a standard form converter has several benefits. Here are some of the advantages of using this tool:

• Saves time: Converting polynomials to standard form can be time-consuming, especially for complex polynomials. A standard form converter can save time by automating this process.
• Reduces errors: Converting polynomials to standard form can be prone to errors, especially for complex polynomials. A standard form converter can reduce errors by ensuring that the polynomial is converted correctly.
• Improves accuracy: A standard form converter can improve accuracy by ensuring that the polynomial is converted correctly.
• Enhances productivity: A standard form converter can enhance productivity by allowing users to focus on other tasks while the tool converts the polynomial.

How to Choose a Standard Form Converter

Choosing a standard form converter depends on several factors. Here are some of the factors to consider:

• Ease of use: The tool should be easy to use, even for users who are not familiar with polynomials.
• Accuracy: The tool should be accurate and reliable.
• Speed: The tool should be fast and efficient.
• Compatibility: The tool should be compatible with different devices and operating systems.

Conclusion

In conclusion, polynomials are essential in various fields, and converting them to standard form is crucial for easier comparison, addition, and subtraction. A standard form converter can make this process easier, saving time and reducing errors. When choosing a standard form converter, consider factors such as ease of use, accuracy, speed, and compatibility.

We hope this article has been informative and helpful. If you have any questions or comments, please feel free to share them below.