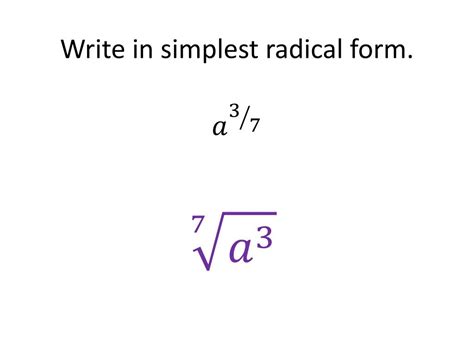
Writing radicals in their simplest form is an essential skill in mathematics, particularly in algebra and geometry. It involves expressing radicals in a way that eliminates any radicals in the denominator and simplifies the expression as much as possible. In this article, we will explore three ways to write radicals in their simplest form.
Understanding Radicals

Before we dive into the methods of simplifying radicals, it's crucial to understand what radicals are and how they work. A radical is a symbol that represents the nth root of a number. The most common type of radical is the square root, which is represented by the symbol √. For example, √16 represents the square root of 16, which equals 4.
Method 1: Factoring Out Perfect Squares

One way to simplify radicals is by factoring out perfect squares. A perfect square is a number that can be expressed as the product of an integer with itself. For example, 16 is a perfect square because it can be expressed as 4 × 4.
To simplify a radical using this method, follow these steps:
- Identify the perfect squares within the radical expression.
- Factor out the perfect squares from the radical expression.
- Simplify the radical expression by expressing the perfect squares as their square roots.
For example, let's simplify the radical expression √48.
- Identify the perfect squares within the radical expression: 48 = 16 × 3, and 16 is a perfect square.
- Factor out the perfect squares from the radical expression: √(16 × 3) = √16 × √3.
- Simplify the radical expression by expressing the perfect squares as their square roots: √16 = 4, so √(16 × 3) = 4√3.
Example Problems
- Simplify the radical expression √75.
- Simplify the radical expression √200.
Method 2: Using the Quotient Rule

Another way to simplify radicals is by using the quotient rule. The quotient rule states that the square root of a quotient is equal to the quotient of the square roots.
To simplify a radical using this method, follow these steps:
- Identify the quotient within the radical expression.
- Apply the quotient rule by expressing the square root of the quotient as the quotient of the square roots.
- Simplify the radical expression by expressing the square roots as their simplest forms.
For example, let's simplify the radical expression √(16/9).
- Identify the quotient within the radical expression: 16/9.
- Apply the quotient rule: √(16/9) = √16 / √9.
- Simplify the radical expression by expressing the square roots as their simplest forms: √16 = 4 and √9 = 3, so √(16/9) = 4/3.
Example Problems
- Simplify the radical expression √(25/4).
- Simplify the radical expression √(49/16).
Method 3: Using the Product Rule

The third way to simplify radicals is by using the product rule. The product rule states that the square root of a product is equal to the product of the square roots.
To simplify a radical using this method, follow these steps:
- Identify the product within the radical expression.
- Apply the product rule by expressing the square root of the product as the product of the square roots.
- Simplify the radical expression by expressing the square roots as their simplest forms.
For example, let's simplify the radical expression √(16 × 3).
- Identify the product within the radical expression: 16 × 3.
- Apply the product rule: √(16 × 3) = √16 × √3.
- Simplify the radical expression by expressing the square roots as their simplest forms: √16 = 4, so √(16 × 3) = 4√3.
Example Problems
- Simplify the radical expression √(25 × 2).
- Simplify the radical expression √(49 × 5).
As you can see, there are several ways to write radicals in their simplest form. By understanding the different methods and practicing them, you'll become proficient in simplifying radicals and be able to tackle more complex math problems with ease.
Conclusion: Simplifying Radicals Made Easy

Simplifying radicals is an essential skill in mathematics, and by mastering the three methods outlined in this article, you'll be able to simplify radicals with ease. Remember to always identify the perfect squares, quotients, or products within the radical expression, and apply the corresponding rule to simplify the expression.
If you have any questions or need further clarification on any of the methods, feel free to ask in the comments below. Don't forget to share this article with your friends and classmates who may be struggling with simplifying radicals.
What is the definition of a radical?
+A radical is a symbol that represents the nth root of a number. The most common type of radical is the square root, which is represented by the symbol √.
What is the quotient rule for radicals?
+The quotient rule states that the square root of a quotient is equal to the quotient of the square roots.
What is the product rule for radicals?
+The product rule states that the square root of a product is equal to the product of the square roots.