Expressing Quadratic Functions In Standard Form Made Easy

Quick Links :

Understanding the Importance of Standard Form in Quadratic Functions

Quadratic functions are a fundamental concept in algebra, and expressing them in standard form is crucial for solving equations, graphing parabolas, and modeling real-world problems. However, many students struggle to convert quadratic functions into standard form, which can lead to difficulties in understanding and working with these functions. In this article, we will explore the importance of standard form in quadratic functions, provide a step-by-step guide on how to express quadratic functions in standard form, and offer practical examples and tips to make this process easier.

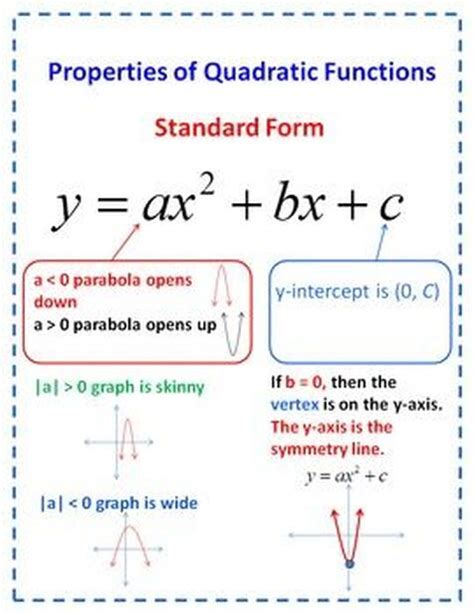
The standard form of a quadratic function is f(x) = ax^2 + bx + c, where a, b, and c are constants, and x is the variable. This form is essential because it allows us to easily identify the vertex, axis of symmetry, and x-intercepts of the parabola, which are critical components of graphing and analyzing quadratic functions.

The Benefits of Expressing Quadratic Functions in Standard Form

Expressing quadratic functions in standard form has numerous benefits, including:

• Easy identification of the vertex: The vertex of the parabola can be found using the formula x = -b/2a, which is only possible when the function is in standard form.
• Simplified graphing: With the function in standard form, we can easily identify the axis of symmetry, x-intercepts, and the direction of the parabola's opening.
• Easier solving of equations: Standard form makes it easier to solve quadratic equations, as we can use the factored form or the quadratic formula to find the solutions.
• Improved modeling of real-world problems: Quadratic functions are used to model various real-world phenomena, such as projectile motion, population growth, and electrical circuits. Expressing these functions in standard form allows us to better understand and analyze these phenomena.

Step-by-Step Guide to Expressing Quadratic Functions in Standard Form

Converting a quadratic function to standard form involves a series of steps:

1. Factor out the greatest common factor (GCF): If the function has a common factor, factor it out to simplify the expression.
2. Complete the square: If the function is not a perfect square trinomial, complete the square to rewrite it in standard form.
3. Use the quadratic formula: If the function cannot be factored or completed into a perfect square, use the quadratic formula to find the solutions and rewrite the function in standard form.

Practical Examples of Expressing Quadratic Functions in Standard Form

Let's consider a few examples:

• Example 1: Express the quadratic function f(x) = x^2 + 5x + 6 in standard form. Solution: Factor out the GCF, which is 1. Then, complete the square to get f(x) = (x + 2)^2 - 4 + 6 = (x + 2)^2 + 2.
• Example 2: Express the quadratic function f(x) = x^2 - 3x - 4 in standard form. Solution: Use the quadratic formula to find the solutions, which are x = -1 and x = 4. Then, rewrite the function in standard form as f(x) = (x + 1)(x - 4).

Tips and Tricks for Expressing Quadratic Functions in Standard Form

• Always factor out the GCF: Before attempting to complete the square or use the quadratic formula, factor out the GCF to simplify the expression.
• Use the quadratic formula wisely: Only use the quadratic formula when the function cannot be factored or completed into a perfect square.
• Check your work: Verify that the function is in standard form by checking that it matches the format f(x) = ax^2 + bx + c.

Common Mistakes to Avoid When Expressing Quadratic Functions in Standard Form

When expressing quadratic functions in standard form, it's essential to avoid common mistakes, such as:

• Forgetting to factor out the GCF: Failing to factor out the GCF can lead to a more complicated expression that is difficult to work with.
• Incorrectly completing the square: Completing the square incorrectly can result in an incorrect standard form.
• Using the quadratic formula unnecessarily: Using the quadratic formula when the function can be factored or completed into a perfect square can lead to unnecessary complexity.

Conclusion: Mastering the Art of Expressing Quadratic Functions in Standard Form

Expressing quadratic functions in standard form is a crucial skill in algebra, and with practice and patience, you can master this technique. By following the step-by-step guide, using practical examples, and avoiding common mistakes, you'll become proficient in converting quadratic functions into standard form. Remember to always factor out the GCF, use the quadratic formula wisely, and check your work to ensure accuracy. With this skill, you'll be able to solve equations, graph parabolas, and model real-world problems with confidence.

We hope this article has helped you understand the importance of expressing quadratic functions in standard form. If you have any questions or need further clarification, please don't hesitate to ask. Share your thoughts and experiences in the comments below, and don't forget to share this article with your friends and classmates who may benefit from it.