Simplify Radicals In Simplest Form Made Easy

Quick Links :

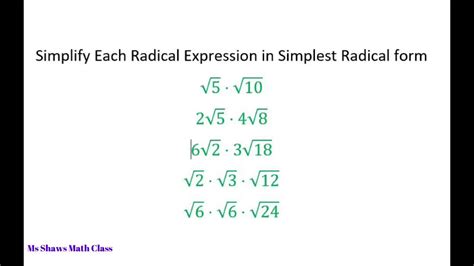
Simplifying radicals is a fundamental concept in mathematics, particularly in algebra and geometry. It's a crucial skill to master, as it enables you to work with radicals in a more efficient and effective way. In this article, we'll delve into the world of radicals, explore the concept of simplifying radicals, and provide you with practical tips and examples to make it easier for you.

Understanding Radicals

A radical, also known as a root, is a mathematical symbol that represents the nth root of a number. For example, the square root of 16 is represented by √16, which equals 4. Radicals are used to express numbers that cannot be expressed as simple fractions. They play a vital role in various mathematical operations, such as solving equations, graphing functions, and calculating distances.

Why Simplify Radicals?

Simplifying radicals is essential for several reasons:

1. Easier calculations: Simplifying radicals makes calculations more manageable and less prone to errors. When radicals are in their simplest form, you can perform arithmetic operations, such as addition, subtraction, multiplication, and division, more easily.
2. Clearer expressions: Simplified radicals make mathematical expressions clearer and more readable. This, in turn, helps you to better understand and analyze mathematical problems.
3. Improved accuracy: Simplifying radicals reduces the risk of errors, as you're working with more manageable numbers.

How to Simplify Radicals

Simplifying radicals involves factoring the radicand (the number inside the radical) into its prime factors and then simplifying the expression. Here are the general steps to follow:

1. Factor the radicand: Break down the radicand into its prime factors.
2. Identify perfect squares or cubes: Look for perfect squares or cubes among the prime factors.
3. Simplify the radical: Take the square root or cube root of the perfect square or cube, and simplify the expression.

Examples of Simplifying Radicals

1. Simplify √16: Factor 16 into its prime factors: 16 = 2 × 2 × 2 × 2. Identify the perfect square: 2 × 2 = 4. Simplify the radical: √16 = √(2 × 2 × 2 × 2) = 4.
2. Simplify √24: Factor 24 into its prime factors: 24 = 2 × 2 × 2 × 3. Identify the perfect square: 2 × 2 = 4. Simplify the radical: √24 = √(2 × 2 × 2 × 3) = 2√6.

Common Mistakes to Avoid

When simplifying radicals, be mindful of the following common mistakes:

1. Forgetting to factor the radicand: Always factor the radicand into its prime factors before simplifying the radical.
2. Not identifying perfect squares or cubes: Take the time to identify perfect squares or cubes among the prime factors.
3. Simplifying incorrectly: Double-check your simplification to ensure it's correct.

Tips and Tricks

To simplify radicals more efficiently, keep the following tips in mind:

1. Use a calculator: Use a calculator to check your simplifications and ensure accuracy.
2. Practice, practice, practice: The more you practice simplifying radicals, the more comfortable you'll become with the process.
3. Break down complex radicals: When dealing with complex radicals, break them down into smaller, more manageable parts.

Simplifying Radicals with Variables

Simplifying radicals with variables requires a slightly different approach. Here are the general steps to follow:

1. Factor the radicand: Break down the radicand into its prime factors, including variables.
2. Identify perfect squares or cubes: Look for perfect squares or cubes among the prime factors, including variables.
3. Simplify the radical: Take the square root or cube root of the perfect square or cube, and simplify the expression.

Examples of Simplifying Radicals with Variables

1. Simplify √(x^2 + 4): Factor the radicand: x^2 + 4 = (x + 2)(x - 2). Identify the perfect square: (x + 2)(x - 2) = (x + 2)^2 - 4. Simplify the radical: √(x^2 + 4) = √((x + 2)^2 - 4) = |x + 2|.
2. Simplify √(2x^3 + 3): Factor the radicand: 2x^3 + 3 = (2x + 1)(x^2 - 2x + 3). Identify the perfect cube: (2x + 1)(x^2 - 2x + 3) = (2x + 1)^3 - 3. Simplify the radical: √(2x^3 + 3) = √((2x + 1)^3 - 3) = |2x + 1|.

Conclusion

Simplifying radicals is a vital skill in mathematics, and with practice, you can master it. By following the steps outlined in this article, you'll be able to simplify radicals with ease and confidence. Remember to factor the radicand, identify perfect squares or cubes, and simplify the expression. Don't be afraid to use a calculator to check your work, and practice regularly to improve your skills.

What's Next?

Now that you've learned how to simplify radicals, it's time to put your skills to the test. Try simplifying radicals with variables and more complex expressions. With time and practice, you'll become proficient in simplifying radicals and be able to tackle more challenging mathematical problems.

FAQs