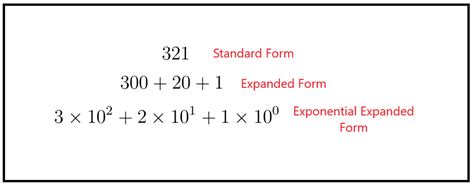
Mastering exponents in expanded form is a crucial math skill that can benefit students in various ways. Not only does it help them understand the concept of exponents, but it also enables them to solve problems more efficiently. In this article, we will explore five ways to master exponents in expanded form, making math more manageable and enjoyable for students.
Understanding the Basics of Exponents
Before diving into the expanded form of exponents, it's essential to understand the basics. An exponent is a shorthand way of writing repeated multiplication of the same number. For instance, 2^3 represents 2 multiplied by itself three times, i.e., 2 × 2 × 2 = 8.

Method 1: Repeated Multiplication
One way to master exponents in expanded form is by using repeated multiplication. This method involves multiplying the base number by itself as many times as the exponent indicates. For example, to expand 2^4, you would multiply 2 by itself four times: 2 × 2 × 2 × 2 = 16.
Using Repeated Multiplication for Expanded Form
| Exponent | Expanded Form |
|---|---|
| 2^2 | 2 × 2 = 4 |
| 2^3 | 2 × 2 × 2 = 8 |
| 2^4 | 2 × 2 × 2 × 2 = 16 |
| 2^5 | 2 × 2 × 2 × 2 × 2 = 32 |
Method 2: Patterns and Relationships
Another way to master exponents in expanded form is by recognizing patterns and relationships. This method involves identifying the pattern between the exponent and the expanded form. For example, when the exponent increases by 1, the expanded form is multiplied by the base number.
Patterns in Exponents
| Exponent | Expanded Form |
|---|---|
| 2^2 | 2 × 2 = 4 |
| 2^3 | 2 × 2 × 2 = 8 ( multiply 4 by 2) |
| 2^4 | 2 × 2 × 2 × 2 = 16 ( multiply 8 by 2) |
| 2^5 | 2 × 2 × 2 × 2 × 2 = 32 ( multiply 16 by 2) |
Method 3: Visualizing Exponents
Visualizing exponents is another effective way to master them in expanded form. This method involves using diagrams or arrays to represent the repeated multiplication. For example, to visualize 2^3, you can create a 2 × 3 array of dots, where each dot represents a single unit.

Using Arrays for Visualization
| Exponent | Array Representation |
|---|---|
| 2^2 | 2 × 2 array |
| 2^3 | 2 × 3 array |
| 2^4 | 2 × 4 array |
| 2^5 | 2 × 5 array |
Method 4: Real-World Applications
Applying exponents to real-world scenarios is an excellent way to master them in expanded form. This method involves using everyday examples to illustrate the concept of exponents. For instance, if a bacteria population doubles every 24 hours, and you start with 100 bacteria, you can use exponents to calculate the population after 3 days: 100 × 2^3 = 800.
Real-World Examples of Exponents
| Scenario | Exponent Representation |
|---|---|
| Bacteria population | 100 × 2^3 = 800 |
| Compound interest | $100 × (1 + 0.05)^5 = $127.67 |
| Population growth | 1000 × 2^4 = 16000 |
Method 5: Practice and Review
Finally, practicing and reviewing exponents regularly is essential to mastering them in expanded form. This method involves using worksheets, online resources, or math games to reinforce understanding and build fluency.
Practice Exercises for Exponents
| Exercise | Answer |
|---|---|
| 2^2 × 2^3 | 2^5 = 32 |
| (3^2)^3 | 3^6 = 729 |
| 4^2 + 4^3 | 16 + 64 = 80 |
In conclusion, mastering exponents in expanded form requires practice, patience, and persistence. By using these five methods – repeated multiplication, patterns and relationships, visualization, real-world applications, and practice and review – students can develop a deep understanding of exponents and become proficient in using them to solve problems.
We hope you found this article informative and helpful in your math journey. If you have any questions or comments, please feel free to share them below.
What is the expanded form of 2^4?
+The expanded form of 2^4 is 2 × 2 × 2 × 2 = 16.
How can I visualize exponents?
+You can visualize exponents using diagrams or arrays, where each dot or unit represents a single multiplication.
What is the real-world application of exponents?
+Exponents have numerous real-world applications, such as calculating population growth, compound interest, and bacteria population.