Exponential Form Of Complex Numbers Simplified

Quick Links :

Unlocking the Power of Complex Numbers: An Introduction

Complex numbers are a fundamental concept in mathematics, playing a crucial role in various fields, including algebra, geometry, trigonometry, and calculus. They are used to represent quantities that have both magnitude and direction, making them essential for solving problems in physics, engineering, and other disciplines. In this article, we will delve into the exponential form of complex numbers, exploring its significance, benefits, and applications.

Understanding Complex Numbers

A complex number is a mathematical expression that combines a real and an imaginary part. It can be represented in the form z = a + bi, where a is the real part, b is the imaginary part, and i is the imaginary unit, which satisfies the equation i^2 = -1. Complex numbers can be added, subtracted, multiplied, and divided, just like real numbers, but with some additional rules.

The Importance of Complex Numbers

Complex numbers have numerous applications in various fields, including:

• Signal processing: Complex numbers are used to represent signals and filter them.
• Control systems: Complex numbers are used to analyze and design control systems.
• Electromagnetism: Complex numbers are used to describe the behavior of electromagnetic waves.
• Quantum mechanics: Complex numbers are used to describe the behavior of subatomic particles.

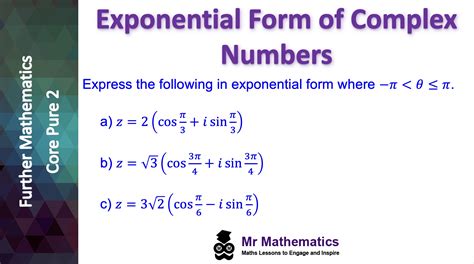
Exponential Form of Complex Numbers

The exponential form of a complex number is a way of expressing it using the exponential function. It is given by:

z = re^(iθ)

where r is the magnitude (or length) of the complex number, e is the base of the natural logarithm, i is the imaginary unit, and θ is the argument (or angle) of the complex number.

Benefits of Exponential Form

The exponential form of complex numbers has several benefits, including:

• Simplified calculations: The exponential form makes it easier to perform calculations with complex numbers.
• Geometric interpretation: The exponential form provides a geometric interpretation of complex numbers, making it easier to visualize and understand their behavior.
• Applications in physics and engineering: The exponential form is used extensively in physics and engineering to describe the behavior of complex systems.

Working with Exponential Form

To work with the exponential form of complex numbers, you need to understand the following:

• Euler's formula: e^(iθ) = cos(θ) + i sin(θ)
• De Moivre's theorem: (e^(iθ))^n = e^(inθ)

These formulas allow you to perform calculations with complex numbers in exponential form.

Examples of Exponential Form

Here are some examples of complex numbers in exponential form:

• z = 2e^(iπ/2) represents a complex number with magnitude 2 and argument π/2.
• z = 3e^(iπ/4) represents a complex number with magnitude 3 and argument π/4.

Applications of Exponential Form

The exponential form of complex numbers has numerous applications in physics, engineering, and other fields, including:

• Signal processing: The exponential form is used to represent and analyze signals.
• Control systems: The exponential form is used to design and analyze control systems.
• Electromagnetism: The exponential form is used to describe the behavior of electromagnetic waves.

Real-World Examples

Here are some real-world examples of the exponential form of complex numbers:

• Electrical engineering: The exponential form is used to analyze and design electrical circuits.
• Mechanical engineering: The exponential form is used to analyze and design mechanical systems.
• Computer science: The exponential form is used in computer graphics and game development.

Conclusion and Future Directions

In conclusion, the exponential form of complex numbers is a powerful tool for representing and analyzing complex systems. Its applications in physics, engineering, and other fields make it an essential concept for students and professionals alike.

As technology continues to advance, the importance of complex numbers and their exponential form will only continue to grow. Future directions for research and development include:

• Quantum computing: The exponential form of complex numbers is used in quantum computing to represent and manipulate qubits.
• Artificial intelligence: The exponential form of complex numbers is used in artificial intelligence to represent and analyze complex data.

We hope this article has provided a comprehensive introduction to the exponential form of complex numbers. Whether you are a student, researcher, or professional, we encourage you to explore the many applications and benefits of this powerful mathematical concept.