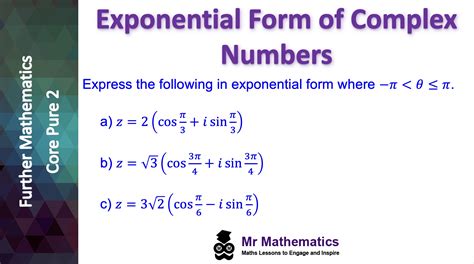
In the realm of mathematics, complex numbers are a fundamental concept that extends the real number system to include imaginary numbers. These numbers are crucial in various fields, such as algebra, geometry, trigonometry, and calculus, as well as in scientific and engineering applications. One of the most powerful and versatile ways to represent complex numbers is in exponential form. In this article, we will delve into the world of exponential form of complex numbers, exploring its definition, benefits, and applications, making it easy for readers to grasp this fascinating topic.
Introduction to Complex Numbers

Complex numbers are numbers that can be expressed in the form a + bi, where a and b are real numbers and i is the imaginary unit, satisfying i^2 = -1. The real part of the complex number is a, and the imaginary part is b. Complex numbers can be represented graphically in the complex plane, where the x-axis represents the real part and the y-axis represents the imaginary part.
What is Exponential Form of Complex Numbers?

The exponential form of complex numbers is a way to represent complex numbers using the exponential function. It is also known as the polar form or trigonometric form. In this form, a complex number is represented as re^(iθ), where r is the magnitude (or length) of the complex number, and θ is the argument (or angle) of the complex number.
Magnitude and Argument of Complex Numbers
The magnitude of a complex number, denoted by |z|, is the distance from the origin to the point representing the complex number in the complex plane. It is calculated using the formula |z| = √(a^2 + b^2), where a and b are the real and imaginary parts of the complex number, respectively.
The argument of a complex number, denoted by Arg(z), is the angle between the positive x-axis and the line segment connecting the origin to the point representing the complex number in the complex plane. It is calculated using the formula Arg(z) = arctan(b/a), where a and b are the real and imaginary parts of the complex number, respectively.
Benefits of Exponential Form of Complex Numbers

The exponential form of complex numbers has several benefits that make it a powerful tool in mathematics and science. Some of the benefits include:
- Simplifies complex calculations: The exponential form makes it easier to perform calculations involving complex numbers, such as multiplication and division.
- Provides a more intuitive understanding: The exponential form provides a more intuitive understanding of complex numbers, as it represents them in terms of magnitude and direction.
- Facilitates visualization: The exponential form makes it easier to visualize complex numbers, as it represents them in the complex plane.
Applications of Exponential Form of Complex Numbers

The exponential form of complex numbers has numerous applications in various fields, including:
- Algebra: The exponential form is used to solve equations involving complex numbers, such as quadratic equations.
- Geometry: The exponential form is used to describe the properties of geometric shapes, such as circles and spirals.
- Trigonometry: The exponential form is used to simplify trigonometric expressions and solve trigonometric equations.
- Calculus: The exponential form is used to solve differential equations and integrate complex functions.
Practical Examples of Exponential Form of Complex Numbers

Here are some practical examples of the exponential form of complex numbers:
- Example 1: Find the exponential form of the complex number 3 + 4i.
Solution: The magnitude of the complex number is |z| = √(3^2 + 4^2) = 5. The argument of the complex number is Arg(z) = arctan(4/3) = 53.13°. Therefore, the exponential form of the complex number is 5e^(i53.13°).
- Example 2: Simplify the expression (2 + 3i) × (4 + 5i) using the exponential form.
Solution: The exponential form of the first complex number is 2e^(i71.57°), and the exponential form of the second complex number is 4e^(i51.34°). Using the properties of exponents, we can simplify the expression as (2 × 4)e^(i(71.57° + 51.34°)) = 8e^(i122.91°).
Conclusion
The exponential form of complex numbers is a powerful tool that simplifies complex calculations, provides a more intuitive understanding, and facilitates visualization. Its applications are numerous, ranging from algebra to calculus. By understanding the exponential form of complex numbers, readers can unlock the secrets of complex number arithmetic and apply them to real-world problems.What is the exponential form of complex numbers?
+The exponential form of complex numbers is a way to represent complex numbers using the exponential function, where a complex number is represented as re^(iθ), with r being the magnitude and θ being the argument of the complex number.
What are the benefits of using the exponential form of complex numbers?
+The exponential form of complex numbers simplifies complex calculations, provides a more intuitive understanding, and facilitates visualization.
What are some practical examples of the exponential form of complex numbers?
+Some practical examples include finding the exponential form of a complex number, simplifying expressions involving complex numbers, and applying the exponential form to solve equations and integrate complex functions.