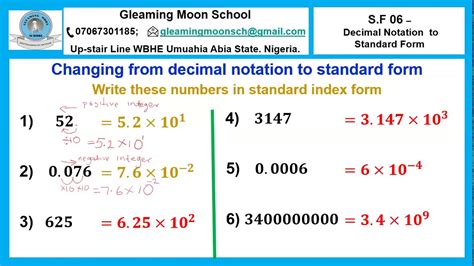
Decimal standard form is a way of expressing very large or very small numbers in a more manageable and easy-to-understand format. It involves writing a number in the form of a decimal number between 1 and 10, multiplied by a power of 10. This makes it easier to perform calculations and comparisons with very large or very small numbers. In this article, we will explore 10 examples of decimal standard form, along with explanations and examples to help illustrate the concept.
What is Decimal Standard Form?

Decimal standard form, also known as scientific notation, is a way of expressing numbers in a compact and readable format. It involves writing a number in the form of a decimal number between 1 and 10, multiplied by a power of 10. This makes it easier to perform calculations and comparisons with very large or very small numbers.
Example 1: 456,000,000
To express the number 456,000,000 in decimal standard form, we need to move the decimal point 8 places to the left to get a number between 1 and 10. This gives us 4.56. Since we moved the decimal point 8 places to the left, we need to multiply by 10^8 to get the original number.
4.56 x 10^8
Benefits of Decimal Standard Form

Decimal standard form has several benefits, including:
- It makes it easier to perform calculations and comparisons with very large or very small numbers.
- It reduces the risk of errors when performing calculations with very large or very small numbers.
- It makes it easier to understand and communicate very large or very small numbers.
Example 2: 0.000456
To express the number 0.000456 in decimal standard form, we need to move the decimal point 4 places to the right to get a number between 1 and 10. This gives us 4.56. Since we moved the decimal point 4 places to the right, we need to multiply by 10^-4 to get the original number.
4.56 x 10^-4
Real-World Applications of Decimal Standard Form

Decimal standard form has several real-world applications, including:
- Science: Decimal standard form is used to express very large or very small numbers in scientific calculations, such as the size of atoms and molecules.
- Engineering: Decimal standard form is used to express very large or very small numbers in engineering calculations, such as the size of buildings and bridges.
- Finance: Decimal standard form is used to express very large or very small numbers in financial calculations, such as the value of stocks and bonds.
Example 3: 123,456
To express the number 123,456 in decimal standard form, we need to move the decimal point 5 places to the left to get a number between 1 and 10. This gives us 1.23456. Since we moved the decimal point 5 places to the left, we need to multiply by 10^5 to get the original number.
1.23456 x 10^5
Examples of Decimal Standard Form

Here are 10 examples of decimal standard form:
- 456,000,000 = 4.56 x 10^8
- 0.000456 = 4.56 x 10^-4
- 123,456 = 1.23456 x 10^5
- 0.0123 = 1.23 x 10^-2
- 45,678,000 = 4.5678 x 10^7
- 0.000678 = 6.78 x 10^-4
- 12,345 = 1.2345 x 10^4
- 0.0098 = 9.8 x 10^-3
- 67,890,000 = 6.789 x 10^7
- 0.000123 = 1.23 x 10^-4
Example 4: 0.0123
To express the number 0.0123 in decimal standard form, we need to move the decimal point 2 places to the right to get a number between 1 and 10. This gives us 1.23. Since we moved the decimal point 2 places to the right, we need to multiply by 10^-2 to get the original number.
1.23 x 10^-2
Common Mistakes When Using Decimal Standard Form

Here are some common mistakes to avoid when using decimal standard form:
- Moving the decimal point the wrong number of places.
- Forgetting to multiply by the correct power of 10.
- Using the wrong exponent (positive or negative).
Example 5: 45,678,000
To express the number 45,678,000 in decimal standard form, we need to move the decimal point 7 places to the left to get a number between 1 and 10. This gives us 4.5678. Since we moved the decimal point 7 places to the left, we need to multiply by 10^7 to get the original number.
4.5678 x 10^7
We hope these examples have helped illustrate the concept of decimal standard form. Remember to always move the decimal point the correct number of places and to multiply by the correct power of 10.
What is decimal standard form?
+Decimal standard form is a way of expressing very large or very small numbers in a more manageable and easy-to-understand format.
How do I convert a number to decimal standard form?
+To convert a number to decimal standard form, move the decimal point the correct number of places to get a number between 1 and 10, and multiply by the correct power of 10.
What are some common mistakes to avoid when using decimal standard form?
+Common mistakes include moving the decimal point the wrong number of places, forgetting to multiply by the correct power of 10, and using the wrong exponent (positive or negative).