3 Ways To Convert Vertex To Standard Form

Quick Links :

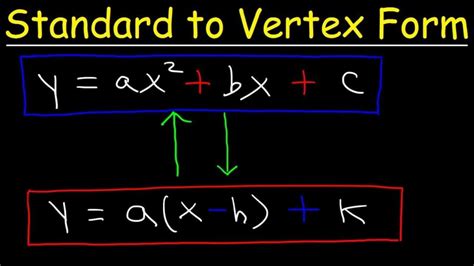
Converting a vertex form equation to standard form is an essential skill in algebra, allowing you to manipulate and solve equations with ease. In this article, we'll explore three ways to convert vertex form to standard form, providing you with a comprehensive understanding of the process.

The importance of converting vertex form to standard form lies in its ability to simplify complex equations, making them easier to work with. Standard form, also known as the general form, is a widely accepted format for writing quadratic equations. By converting vertex form to standard form, you can compare and analyze equations more effectively.

Method 1: Using the Vertex Form Formula

The vertex form formula is a straightforward way to convert vertex form to standard form. The formula is:

y = a(x - h)^2 + k

where (h, k) is the vertex of the parabola.

To convert vertex form to standard form using this formula, follow these steps:

• Expand the squared term: (x - h)^2 = x^2 - 2hx + h^2
• Multiply the coefficient "a" by the expanded term: ax^2 - 2ahx + ah^2
• Add the constant term "k" to the result: ax^2 - 2ahx + ah^2 + k

This will give you the standard form of the equation.

Example 1: Converting Vertex Form to Standard Form Using the Formula

Suppose we have the vertex form equation:

y = 2(x - 3)^2 + 1

Using the formula, we can expand the squared term:

(x - 3)^2 = x^2 - 6x + 9

Multiplying the coefficient "2" by the expanded term:

2x^2 - 12x + 18

Adding the constant term "1":

2x^2 - 12x + 19

This is the standard form of the equation.

Method 2: Using the Completing the Square Method

Completing the square is another method for converting vertex form to standard form. This method involves manipulating the equation to create a perfect square trinomial.

To complete the square, follow these steps:

• Move the constant term to the right-hand side of the equation
• Take half of the coefficient of the x-term and square it
• Add the result to both sides of the equation
• Factor the left-hand side of the equation into a perfect square trinomial

Example 2: Converting Vertex Form to Standard Form Using Completing the Square

Suppose we have the vertex form equation:

y = x^2 + 4x + 3

Moving the constant term to the right-hand side:

y - 3 = x^2 + 4x

Taking half of the coefficient of the x-term and squaring it:

(4/2)^2 = 4

Adding the result to both sides:

y - 3 + 4 = x^2 + 4x + 4

Factoring the left-hand side:

y + 1 = (x + 2)^2

This is the standard form of the equation.

Method 3: Using a Graphing Calculator

A graphing calculator can also be used to convert vertex form to standard form. This method is particularly useful when working with complex equations.

To use a graphing calculator, follow these steps:

• Enter the vertex form equation into the calculator
• Graph the equation to find the vertex
• Use the calculator's built-in functions to convert the equation to standard form

Example 3: Converting Vertex Form to Standard Form Using a Graphing Calculator

Suppose we have the vertex form equation:

y = 3(x - 2)^2 + 1

Entering the equation into a graphing calculator and graphing it, we can find the vertex at (2, 1). Using the calculator's built-in functions, we can convert the equation to standard form:

y = 3x^2 - 12x + 11

This is the standard form of the equation.

In conclusion, converting vertex form to standard form is an essential skill in algebra. By using the vertex form formula, completing the square method, or a graphing calculator, you can simplify complex equations and make them easier to work with. Whether you're a student or a professional, mastering these methods will help you to better understand and manipulate quadratic equations.