Convert Standard Form To Slope Intercept Form Easily

Quick Links :

Converting standard form to slope-intercept form is a crucial skill in algebra and is used extensively in various mathematical and real-world applications. The slope-intercept form, denoted as y = mx + b, provides an intuitive way to understand the relationship between the dependent and independent variables in a linear equation. In this article, we will delve into the world of linear equations, explore the benefits of converting standard form to slope-intercept form, and provide a step-by-step guide on how to do it easily.

Why Convert Standard Form to Slope-Intercept Form?

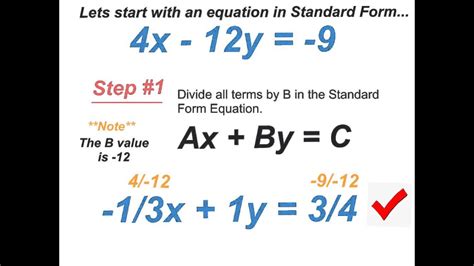
The standard form of a linear equation is represented as Ax + By = C, where A, B, and C are constants. While this form is useful for various algebraic manipulations, it does not provide an immediate understanding of the line's slope and y-intercept. In contrast, the slope-intercept form (y = mx + b) explicitly shows the slope (m) and the y-intercept (b), making it easier to visualize and analyze the line's behavior.

Benefits of Slope-Intercept Form

1. Easy Visualization: With the slope-intercept form, you can quickly determine the line's slope and y-intercept, allowing for effortless visualization of the line's orientation and position.
2. Simplified Analysis: The slope-intercept form facilitates the analysis of the line's behavior, enabling you to identify key features such as the x-intercept, maximum/minimum points, and the line's direction.
3. Efficient Calculations: Converting to slope-intercept form often simplifies calculations, especially when dealing with systems of linear equations or linear inequalities.

Step-by-Step Guide to Converting Standard Form to Slope-Intercept Form

Converting a linear equation from standard form (Ax + By = C) to slope-intercept form (y = mx + b) involves a straightforward process:

Step 1: Rearrange the Equation

Move the x-term to the right-hand side of the equation, and the constant term to the left-hand side:

Ax + By = C → By = -Ax + C

Step 2: Isolate y

Divide both sides of the equation by B to isolate y:

y = (-A/B)x + C/B

Step 3: Simplify the Equation

Combine the fractions and simplify the equation to obtain the slope-intercept form:

y = (-(A/B))x + (C/B)

Example 1: Converting a Simple Linear Equation

Standard form: 2x + 3y = 5

Rearrange the equation: 3y = -2x + 5

Isolate y: y = (-2/3)x + 5/3

Simplify: y = (-2/3)x + 5/3

Example 2: Converting a Linear Equation with Negative Coefficients

Standard form: -4x - 2y = -8

Rearrange the equation: -2y = 4x - 8

Isolate y: y = (-4/2)x + 8/2

Simplify: y = (-2)x + 4

Common Mistakes to Avoid

1. Incorrect Sign: When rearranging the equation, ensure that you maintain the correct signs for the coefficients.
2. Insufficient Simplification: Always simplify the fractions and combine like terms to obtain the slope-intercept form.

Real-World Applications

Converting standard form to slope-intercept form has numerous real-world applications, including:

1. Physics: Analyzing the motion of objects, where the slope represents the velocity and the y-intercept represents the initial position.
2. Economics: Modeling the relationship between price and demand, where the slope represents the elasticity of demand and the y-intercept represents the intercept.
3. Computer Science: Representing linear relationships in programming, where the slope-intercept form facilitates calculations and visualizations.

Takeaways and Call to Action

Converting standard form to slope-intercept form is a fundamental skill in algebra that provides numerous benefits, including easy visualization, simplified analysis, and efficient calculations. By following the step-by-step guide outlined in this article, you can easily convert linear equations to slope-intercept form and apply this skill in various real-world applications.

We encourage you to practice converting standard form to slope-intercept form using the examples provided and to explore the numerous real-world applications of this skill. Share your experiences, ask questions, and provide feedback in the comments section below.