5 Steps To Convert Complex Numbers To Trigonometric Form

Quick Links :

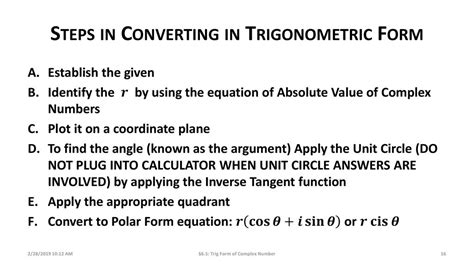
Understanding the Importance of Converting Complex Numbers to Trigonometric Form

In mathematics, complex numbers are used to extend the real number system to include imaginary numbers. These numbers are essential in various fields, including algebra, calculus, and engineering. However, working with complex numbers in their standard form can be challenging. Converting complex numbers to trigonometric form simplifies many mathematical operations and provides a more intuitive understanding of these numbers. In this article, we will explore the five steps to convert complex numbers to trigonometric form.

The Need for Trigonometric Form

The trigonometric form of complex numbers is also known as polar form. It represents a complex number as a sum of its magnitude (length) and angle (argument). This form is more convenient for certain mathematical operations, such as multiplication and division. Additionally, trigonometric form provides a clear visual representation of complex numbers, making it easier to understand their properties and relationships.

Visual Representation of Complex Numbers

Imagine a complex number as a point on the complex plane. The x-axis represents the real part, while the y-axis represents the imaginary part. The distance from the origin to the point is the magnitude (length) of the complex number. The angle between the positive x-axis and the line connecting the origin to the point is the argument (angle) of the complex number.

Step 1: Write the Complex Number in Standard Form

To convert a complex number to trigonometric form, we first need to write it in standard form. The standard form of a complex number is a + bi, where a is the real part and b is the imaginary part.

For example, consider the complex number 3 + 4i. Here, a = 3 and b = 4.

Why Standard Form is Essential

Writing a complex number in standard form is crucial for conversion to trigonometric form. It allows us to identify the real and imaginary parts, which are necessary for calculating the magnitude and angle.

Step 2: Calculate the Magnitude of the Complex Number

The magnitude (length) of a complex number is calculated using the formula:

Magnitude = √(a^2 + b^2)

where a is the real part and b is the imaginary part.

Using the example from Step 1, the magnitude of 3 + 4i is:

Magnitude = √(3^2 + 4^2) = √(9 + 16) = √25 = 5

Importance of Magnitude in Trigonometric Form

The magnitude is a critical component of the trigonometric form of complex numbers. It represents the distance from the origin to the point on the complex plane.

Step 3: Calculate the Angle of the Complex Number

The angle (argument) of a complex number is calculated using the formula:

Angle = arctan(b/a)

where a is the real part and b is the imaginary part.

Using the example from Step 1, the angle of 3 + 4i is:

Angle = arctan(4/3) ≈ 53.13°

Understanding the Angle in Trigonometric Form

The angle is another essential component of the trigonometric form of complex numbers. It represents the angle between the positive x-axis and the line connecting the origin to the point on the complex plane.

Step 4: Write the Complex Number in Trigonometric Form

Using the magnitude and angle calculated in Steps 2 and 3, we can write the complex number in trigonometric form:

Trigonometric Form = Magnitude × cis(Angle)

where cis(Angle) = cos(Angle) + i sin(Angle)

Using the example from Step 1, the trigonometric form of 3 + 4i is:

Trigonometric Form = 5 × cis(53.13°) = 5(cos(53.13°) + i sin(53.13°))

Benefits of Trigonometric Form

The trigonometric form of complex numbers simplifies many mathematical operations and provides a more intuitive understanding of these numbers.

Step 5: Verify the Conversion

Finally, we need to verify that the conversion is correct. We can do this by converting the trigonometric form back to standard form.

Using the example from Step 1, we can convert the trigonometric form back to standard form:

5(cos(53.13°) + i sin(53.13°)) = 3 + 4i

This verifies that the conversion is correct.

Conclusion and Future Directions

In conclusion, converting complex numbers to trigonometric form is a powerful technique for simplifying mathematical operations and gaining a deeper understanding of these numbers. By following the five steps outlined in this article, you can master this technique and apply it to a wide range of mathematical problems. Remember to always verify your conversions to ensure accuracy.

Practical Applications of Trigonometric Form

The trigonometric form of complex numbers has numerous practical applications in fields such as electrical engineering, signal processing, and physics.

Encourage readers to explore these applications and experiment with converting complex numbers to trigonometric form using various mathematical software tools.