3 Steps To Write Complex Numbers In Standard Form

Quick Links :

Hide

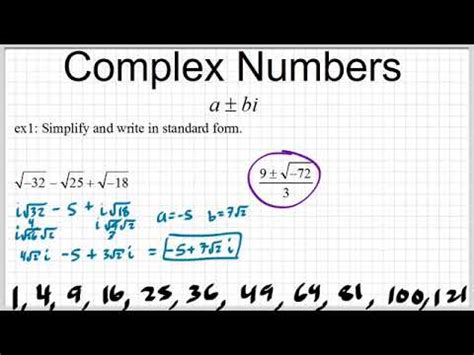
Understanding complex numbers is crucial in various mathematical and scientific applications, including algebra, calculus, and physics. Complex numbers are expressions that have both real and imaginary components, which can be represented in the form a + bi, where 'a' is the real part and 'bi' is the imaginary part. Writing complex numbers in standard form, which is a way of expressing these numbers in a consistent and clear manner, is essential for solving equations and performing operations with complex numbers. In this article, we will explore the steps to write complex numbers in standard form, explaining the process in detail and providing examples to illustrate each step.

Step 1: Simplify the Expression

The first step in writing complex numbers in standard form is to simplify the given expression. This involves combining like terms and ensuring that the real and imaginary parts are clearly separated. When simplifying, remember that the real part is the part without the imaginary unit 'i', while the imaginary part is the coefficient of 'i'. For example, if we have the expression 2 + 3i + 4 - 2i, we can simplify it by combining the real parts (2 + 4) and the imaginary parts (3i - 2i), resulting in 6 + i.

Example:

Simplify the expression 3 + 2i - 1 - 4i.

To simplify this expression, we combine the real parts (3 - 1) and the imaginary parts (2i - 4i), resulting in 2 - 2i.

Step 2: Write the Expression in a + bi Form

Once the expression is simplified, the next step is to write it in the standard form a + bi. This involves ensuring that the real part 'a' is written first, followed by the imaginary part 'bi'. If the imaginary part is negative, the minus sign should be written before the imaginary unit 'i'. For example, if we have the simplified expression 2 - 3i, it is already in standard form. However, if we have an expression like 3i + 2, we need to rearrange it to 2 + 3i to write it in standard form.

Example:

Write the expression 4i - 3 in standard form.

To write this expression in standard form, we need to rearrange it to have the real part first, followed by the imaginary part. The correct standard form is -3 + 4i.

Step 3: Check for Any Remaining Simplifications

The final step is to check the expression for any remaining simplifications. This involves ensuring that the real and imaginary parts are fully simplified and that there are no remaining like terms that can be combined. Additionally, check if the expression can be written in a more simplified form, such as combining complex fractions or eliminating any redundant terms.

Example:

Check the expression 2 + (3 + 2i)i for any remaining simplifications.

To check for remaining simplifications, we first need to simplify the expression inside the parentheses. The expression (3 + 2i)i can be simplified by distributing the imaginary unit 'i' to the terms inside the parentheses, resulting in 3i + 2i^2. Since i^2 = -1, we can further simplify the expression to 3i - 2. Now, the original expression 2 + (3 + 2i)i can be rewritten as 2 + 3i - 2, which simplifies to 3i.

By following these three steps, we can write complex numbers in standard form, making it easier to perform operations and solve equations involving complex numbers.

Practical Applications of Complex Numbers

Complex numbers have numerous practical applications in various fields, including physics, engineering, and computer science. In physics, complex numbers are used to describe the behavior of electrical circuits and the motion of objects in two-dimensional space. In engineering, complex numbers are used in signal processing and control systems. In computer science, complex numbers are used in algorithms for solving systems of linear equations and in computer graphics.

Example:

Use complex numbers to describe the impedance of an electrical circuit.

The impedance of an electrical circuit can be represented as a complex number Z = R + iX, where R is the resistance and X is the reactance. By using complex numbers, we can easily calculate the magnitude and phase angle of the impedance, which is essential for designing and analyzing electrical circuits.

Conclusion - Mastering Complex Numbers

Mastering complex numbers is crucial for success in various mathematical and scientific applications. By understanding how to write complex numbers in standard form, we can perform operations and solve equations with ease. Whether you are a student or a professional, complex numbers are an essential tool in your toolkit. With practice and patience, you can become proficient in working with complex numbers and unlock the doors to new mathematical and scientific discoveries.

We hope this article has been helpful in explaining the steps to write complex numbers in standard form. If you have any questions or comments, please share them with us below.