Complex Numbers In Standard Form Made Easy

Quick Links :

Understanding complex numbers in standard form is a crucial concept in mathematics, particularly in algebra and calculus. Complex numbers are used to extend the real number system to include numbers that can be expressed in the form a + bi, where a and b are real numbers and i is the imaginary unit, which satisfies i^2 = -1. In this article, we will delve into the world of complex numbers, exploring their definition, importance, and how to express them in standard form.

What are Complex Numbers?

Complex numbers are numbers that have both real and imaginary parts. The real part is the part that is not multiplied by i, while the imaginary part is the part that is multiplied by i. For example, in the complex number 3 + 4i, 3 is the real part and 4i is the imaginary part.

Importance of Complex Numbers

Complex numbers have numerous applications in mathematics, physics, engineering, and other fields. They are used to solve equations that cannot be solved using only real numbers, such as quadratic equations with negative discriminants. Complex numbers are also used in electrical engineering, signal processing, and control systems.

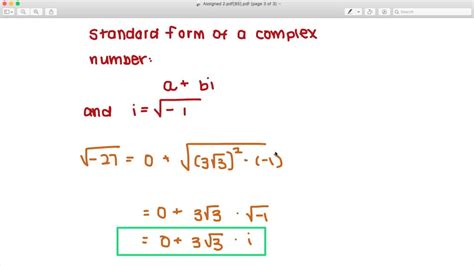
Standard Form of Complex Numbers

The standard form of a complex number is a + bi, where a and b are real numbers and i is the imaginary unit. To express a complex number in standard form, we need to combine the real and imaginary parts.

For example, to express the complex number 2 + 3i in standard form, we can write it as 2 + 3i. However, to express the complex number 2 - 3i in standard form, we need to rewrite it as 2 + (-3)i.

Rules for Expressing Complex Numbers in Standard Form

Here are some rules to follow when expressing complex numbers in standard form:

• The real part a should be written first.
• The imaginary part bi should be written second.
• If the imaginary part is negative, write it as -bi.
• If the imaginary part is zero, omit it.
• If the real part is zero, omit it.

Examples of Complex Numbers in Standard Form

Here are some examples of complex numbers in standard form:

• 2 + 3i
• 4 - 2i
• 0 + 5i
• 3 + 0i

Operations with Complex Numbers

We can perform various operations with complex numbers, such as addition, subtraction, multiplication, and division.

• Addition: (a + bi) + (c + di) = (a + c) + (b + d)i
• Subtraction: (a + bi) - (c + di) = (a - c) + (b - d)i
• Multiplication: (a + bi)(c + di) = (ac - bd) + (ad + bc)i
• Division: (a + bi)/(c + di) = ((ac + bd)/(c^2 + d^2)) + ((bc - ad)/(c^2 + d^2))i

Applications of Complex Numbers

Complex numbers have numerous applications in mathematics, physics, engineering, and other fields. Some examples include:

• Electrical engineering: Complex numbers are used to analyze AC circuits and filters.
• Signal processing: Complex numbers are used to represent signals and filter them.
• Control systems: Complex numbers are used to analyze and design control systems.

Real-World Examples

Here are some real-world examples of complex numbers in action:

• Medical imaging: Complex numbers are used in medical imaging techniques such as MRI and CT scans.
• Navigation: Complex numbers are used in GPS systems to determine location and direction.
• Finance: Complex numbers are used in finance to model and analyze complex systems.

Conclusion

In conclusion, complex numbers in standard form are an essential concept in mathematics, with numerous applications in physics, engineering, and other fields. By understanding the rules for expressing complex numbers in standard form, we can perform various operations with them and apply them to real-world problems.

We hope this article has helped you understand complex numbers in standard form and their importance in mathematics and real-world applications. Share your thoughts and feedback in the comments section below!