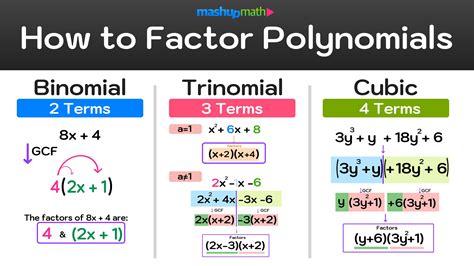
Identifying standard form polynomials is a crucial skill in algebra, and it can seem daunting at first, but with practice and the right strategies, it becomes second nature. In this article, we will explore three ways to identify standard form polynomials, along with practical examples and explanations to help solidify your understanding.
What are Standard Form Polynomials?

Standard form polynomials, also known as canonical form, are polynomials written in a specific format, making it easier to perform operations like addition, subtraction, and multiplication. A standard form polynomial is written in descending order of exponents, with the term having the highest exponent first, followed by the term with the next highest exponent, and so on.
Example of a Standard Form Polynomial
For instance, the polynomial 3x^2 + 2x - 4 is in standard form because the term with the highest exponent (3x^2) comes first, followed by the term with the next highest exponent (2x), and finally the constant term (-4).
Method 1: Identify the Exponents

One way to identify standard form polynomials is to look at the exponents of the variables. In a standard form polynomial, the exponents should be in descending order. Start by identifying the term with the highest exponent and check if it comes first in the polynomial. Then, move on to the next term and check if its exponent is lower than the previous one. Continue this process until you reach the constant term.
Example
Consider the polynomial x^3 + 2x^2 - 5x + 1. To determine if it's in standard form, start by identifying the exponents: 3, 2, 1, and 0 (the constant term). Since the exponents are in descending order, this polynomial is indeed in standard form.
Method 2: Check the Coefficients

Another way to identify standard form polynomials is to look at the coefficients of the terms. In a standard form polynomial, the coefficients should be written in a specific order. Start by looking at the coefficient of the term with the highest exponent. Then, move on to the coefficient of the term with the next highest exponent, and so on.
Example
Consider the polynomial 2x^2 + 3x - 4. To determine if it's in standard form, start by looking at the coefficients: 2, 3, and -4. Since the coefficients are written in the correct order (i.e., the coefficient of the term with the highest exponent comes first), this polynomial is indeed in standard form.
Method 3: Use the "Descending Order" Rule

A third way to identify standard form polynomials is to use the "descending order" rule. This rule states that in a standard form polynomial, the terms should be written in descending order of exponents. Start by looking at the first term and check if its exponent is higher than the exponent of the second term. Then, move on to the second term and check if its exponent is higher than the exponent of the third term, and so on.
Example
Consider the polynomial x^4 - 2x^3 + 3x^2 - 4x + 1. To determine if it's in standard form, start by applying the descending order rule: x^4 comes before x^3, which comes before x^2, and so on. Since the terms are written in descending order of exponents, this polynomial is indeed in standard form.
What is the definition of a standard form polynomial?
+A standard form polynomial is a polynomial written in a specific format, with the term having the highest exponent first, followed by the term with the next highest exponent, and so on.
How can I identify standard form polynomials?
+You can identify standard form polynomials by looking at the exponents, coefficients, or using the "descending order" rule.
What are some common mistakes to avoid when identifying standard form polynomials?
+Some common mistakes to avoid include not checking the order of the exponents, not looking at the coefficients, and not applying the descending order rule correctly.
In conclusion, identifying standard form polynomials is a crucial skill in algebra, and it can be achieved by using one of the three methods discussed in this article. By practicing these methods and applying them to different polynomials, you will become proficient in identifying standard form polynomials in no time.