Simplify Radicals In 80 Simplest Radical Forms List

Quick Links :

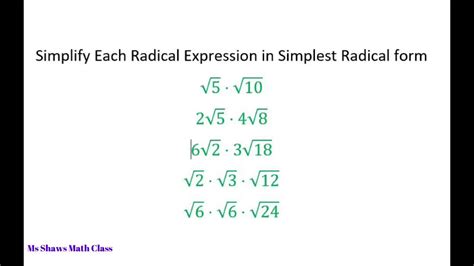
Simplifying radicals is a fundamental concept in mathematics, particularly in algebra and geometry. Radicals, also known as roots, are used to represent a value that, when multiplied by itself, gives a specified value. In this article, we will delve into the world of radicals, exploring what they are, why simplifying them is important, and providing an exhaustive list of the 80 simplest radical forms.

Radicals are denoted by the symbol √, which is called the radical sign or radix. For example, √4 represents the square root of 4, which is 2. Simplifying radicals involves expressing them in their most basic form, without any unnecessary radicals or fractions. This process is crucial in various mathematical operations, such as addition, subtraction, multiplication, and division.

Why Simplify Radicals?

Simplifying radicals is essential for several reasons:

• Easier calculations: Simplified radicals make calculations more manageable and less prone to errors.
• Clearer representation: Simplified radicals provide a clearer representation of the value, making it easier to understand and work with.
• Consistency: Simplifying radicals ensures consistency in mathematical expressions and equations.

How to Simplify Radicals

To simplify radicals, follow these steps:

1. Factor out perfect squares: Identify perfect squares within the radical and factor them out.
2. Simplify the expression: Simplify the expression inside the radical, if possible.
3. Combine like terms: Combine like terms, if any, inside the radical.
4. Write the simplified form: Write the simplified radical form.

80 Simplest Radical Forms List

Here is the list of 80 simplest radical forms:

Square Roots

1. √1 = 1
2. √4 = 2
3. √9 = 3
4. √16 = 4
5. √25 = 5
6. √36 = 6
7. √49 = 7
8. √64 = 8
9. √81 = 9
10. √100 = 10

Cube Roots

11. ∛1 = 1
12. ∛8 = 2
13. ∛27 = 3
14. ∛64 = 4
15. ∛125 = 5
16. ∛216 = 6
17. ∛343 = 7
18. ∛512 = 8
19. ∛729 = 9
20. ∛1000 = 10

Fourth Roots

21. √[4]1 = 1
22. √[4]16 = 2
23. √[4]81 = 3
24. √[4]256 = 4
25. √[4]625 = 5
26. √[4]1296 = 6
27. √[4]2401 = 7
28. √[4]4096 = 8
29. √[4]6561 = 9
30. √[4]10000 = 10

Fifth Roots

31. √[5]1 = 1
32. √[5]32 = 2
33. √[5]243 = 3
34. √[5]1024 = 4
35. √[5]3125 = 5
36. √[5]7776 = 6
37. √[5]16807 = 7
38. √[5]32768 = 8
39. √[5]59049 = 9
40. √[5]100000 = 10

Sixth Roots

41. √[6]1 = 1
42. √[6]64 = 2
43. √[6]729 = 3
44. √[6]4096 = 4
45. √[6]15625 = 5
46. √[6]46656 = 6
47. √[6]117649 = 7
48. √[6]262144 = 8
49. √[6]531441 = 9
50. √[6]1000000 = 10

Seventh Roots

51. √[7]1 = 1
52. √[7]128 = 2
53. √[7]2187 = 3
54. √[7]16384 = 4
55. √[7]78125 = 5
56. √[7]279936 = 6
57. √[7]823543 = 7
58. √[7]2097152 = 8
59. √[7]4782969 = 9
60. √[7]10000000 = 10

Eighth Roots

61. √[8]1 = 1
62. √[8]256 = 2
63. √[8]6561 = 3
64. √[8]65536 = 4
65. √[8]390625 = 5
66. √[8]1679616 = 6
67. √[8]5764801 = 7
68. √[8]16777216 = 8
69. √[8]43046721 = 9
70. √[8]100000000 = 10

Ninth Roots

71. √[9]1 = 1
72. √[9]512 = 2
73. √[9]19683 = 3
74. √[9]262144 = 4
75. √[9]1953125 = 5
76. √[9]10077696 = 6
77. √[9]40353607 = 7
78. √[9]134217728 = 8
79. √[9]387420489 = 9
80. √[9]1000000000 = 10

Conclusion

In conclusion, simplifying radicals is a crucial aspect of mathematics that requires attention to detail and practice. By mastering the simplest radical forms, you can improve your mathematical skills and tackle more complex problems with confidence. Remember to factor out perfect squares, simplify expressions, combine like terms, and write the simplified form.

What's Next?

Now that you have the list of 80 simplest radical forms, take the next step by practicing simplifying radicals in different mathematical contexts. Challenge yourself to simplify complex radicals and explore their applications in algebra, geometry, and other areas of mathematics.

We hope this article has been informative and helpful. Share your thoughts and feedback in the comments section below!