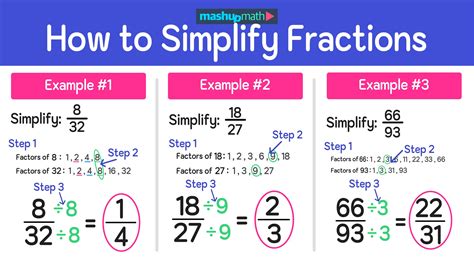
As a math enthusiast, I'm excited to share with you the process of simplifying fractions. Simplifying a fraction means reducing it to its lowest terms while maintaining its original value. In this article, we'll explore the steps to simplify 47 as a fraction, although it's a whole number and doesn't have a fractional part, we will explore some related concepts.
Understanding Fractions
Before we dive into simplifying fractions, let's quickly review what fractions are. A fraction is a way to express a part of a whole as a ratio of two numbers. It consists of a numerator (the top number) and a denominator (the bottom number). For example, 3/4 is a fraction where 3 is the numerator and 4 is the denominator.

Why Simplify Fractions?
Simplifying fractions is essential in mathematics because it helps to:
- Reduce complexity: Simplifying fractions makes them easier to work with and understand.
- Avoid confusion: Simplified fractions help to prevent errors and confusion when performing mathematical operations.
- Improve communication: Simplified fractions facilitate clearer communication and comparison of mathematical values.
Simplifying 47 as a Fraction
As mentioned earlier, 47 is a whole number and doesn't have a fractional part. However, if we were to express it as a fraction, it would be 47/1. This fraction is already in its simplest form, as the numerator and denominator have no common factors other than 1.
However, let's explore a related concept. Suppose we have a fraction like 94/2. To simplify this fraction, we would need to find the greatest common divisor (GCD) of the numerator and denominator.
Finding the Greatest Common Divisor (GCD)
To simplify a fraction, we need to find the GCD of the numerator and denominator. The GCD is the largest number that divides both numbers without leaving a remainder.
For example, let's find the GCD of 94 and 2:
- Factors of 94: 1, 2, 47, 94
- Factors of 2: 1, 2
The GCD of 94 and 2 is 2.
Simplifying the Fraction
Now that we have the GCD, we can simplify the fraction:
94 ÷ 2 = 47 2 ÷ 2 = 1
So, the simplified fraction is 47/1.

Steps to Simplify Fractions
Here are the general steps to simplify fractions:
- Check if the fraction is already simplified: If the numerator and denominator have no common factors other than 1, the fraction is already simplified.
- Find the greatest common divisor (GCD): Identify the largest number that divides both the numerator and denominator without leaving a remainder.
- Divide the numerator and denominator by the GCD: This will give you the simplified fraction.
Real-World Applications
Simplifying fractions has numerous real-world applications, including:
- Cooking and recipes: Simplifying fractions helps with scaling recipes and converting between different units of measurement.
- Finance and business: Simplifying fractions is essential for calculating interest rates, investment returns, and other financial metrics.
- Science and engineering: Simplifying fractions is crucial for calculating quantities and rates in various scientific and engineering applications.

Conclusion: Simplify with Confidence
In conclusion, simplifying fractions is an essential math skill that can help you tackle complex problems with confidence. By following the steps outlined in this article, you'll be able to simplify fractions with ease. Remember, practice makes perfect, so be sure to try simplifying different fractions to reinforce your understanding.
What's your favorite way to simplify fractions? Share your thoughts in the comments below!
FAQ Section:
What is the purpose of simplifying fractions?
+Simplifying fractions helps to reduce complexity, avoid confusion, and improve communication in mathematical expressions.
How do I find the greatest common divisor (GCD) of two numbers?
+The GCD is the largest number that divides both numbers without leaving a remainder. You can find the GCD by listing the factors of each number and identifying the largest common factor.
What are some real-world applications of simplifying fractions?
+Simplifying fractions has numerous real-world applications, including cooking, finance, business, science, and engineering.