Simplifying 45 To Its Simplest Form

Quick Links :

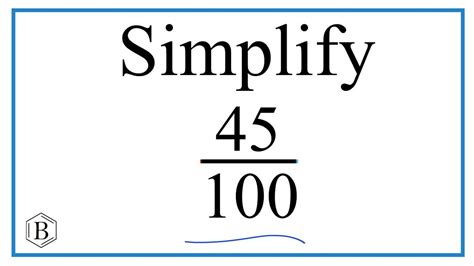
Reducing fractions to their simplest form is an essential math skill that can be applied to various real-life situations. Simplifying 45 to its simplest form is a straightforward process that requires understanding the concept of equivalent fractions and the greatest common divisor (GCD).

Understanding Equivalent Fractions

Equivalent fractions are fractions that represent the same value but have different numerators and denominators. For example, 1/2 is equivalent to 2/4 and 3/6. To simplify a fraction, we need to find an equivalent fraction with the smallest possible numerator and denominator.

What is Simplifying 45 to Its Simplest Form?

Simplifying 45 to its simplest form means reducing the fraction to its lowest terms while preserving its value. Since 45 is not a fraction, we assume you meant 45/100, which is a common fraction.

How to Simplify 45 to Its Simplest Form

To simplify 45/100, we need to find the greatest common divisor (GCD) of 45 and 100.

The GCD of 45 and 100 is 5. To simplify the fraction, we divide both the numerator and the denominator by the GCD.

45 ÷ 5 = 9 100 ÷ 5 = 20

So, the simplified form of 45/100 is 9/20.

Benefits of Simplifying Fractions

Simplifying fractions has numerous benefits in various aspects of life:

• Easier calculations: Simplifying fractions makes it easier to perform calculations, such as adding, subtracting, multiplying, and dividing fractions.
• Improved understanding: Simplifying fractions helps to develop a deeper understanding of mathematical concepts, such as equivalent ratios and proportions.
• Real-life applications: Simplifying fractions is essential in real-life situations, such as cooking, measurement, and finance.

Common Mistakes to Avoid

When simplifying fractions, there are common mistakes to avoid:

• Dividing by zero: Never divide a fraction by zero, as this will result in an undefined value.
• Dividing by a non-common factor: Make sure to divide both the numerator and the denominator by the greatest common divisor (GCD) to avoid incorrect simplification.
• Rounding errors: Avoid rounding errors by ensuring that the simplified fraction is in its lowest terms.

Tips and Tricks

Here are some tips and tricks to help you simplify fractions:

• Use visual aids: Visual aids, such as diagrams and charts, can help you understand the concept of equivalent fractions and simplify fractions more efficiently.
• Practice, practice, practice: The more you practice simplifying fractions, the more comfortable you will become with the process.
• Use online tools: Online tools, such as fraction simplifiers, can help you simplify fractions quickly and accurately.

Real-World Applications

Simplifying fractions has numerous real-world applications:

• Cooking: Simplifying fractions is essential in cooking, as it helps to measure ingredients accurately and adjust recipes accordingly.
• Measurement: Simplifying fractions is crucial in measurement, as it helps to convert between different units of measurement.
• Finance: Simplifying fractions is important in finance, as it helps to calculate interest rates, investment returns, and other financial metrics.

Conclusion

In conclusion, simplifying 45 to its simplest form is a straightforward process that requires understanding the concept of equivalent fractions and the greatest common divisor (GCD). By following the steps outlined in this article, you can simplify fractions with ease and accuracy. Remember to avoid common mistakes, use visual aids, and practice regularly to become proficient in simplifying fractions.

Frequently Asked Questions