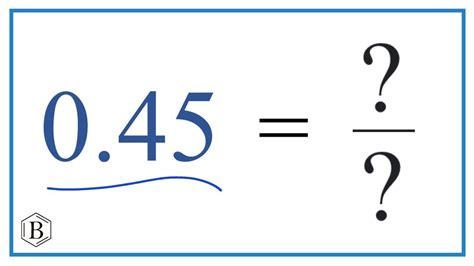45 As A Fraction: Simplified

Quick Links :

When it comes to fractions, simplicity is key. Fractions are a way to express a part of a whole, and simplifying them makes it easier to work with and understand the underlying math. In this article, we'll explore what 45 as a fraction looks like and how to simplify it.

45 is an integer, a whole number, and as such, it can be expressed as a fraction by placing it over 1. So, 45 as a fraction is 45/1.

Understanding Equivalent Fractions

Before we dive into simplifying 45/1, it's essential to understand the concept of equivalent fractions. Equivalent fractions are fractions that represent the same value but have different numerators and denominators. For example, 1/2 is equivalent to 2/4, 3/6, and 4/8. These fractions all represent the same proportion of a whole.

Why Simplify Fractions?

Simplifying fractions is crucial in mathematics because it helps in:

• Reducing complexity: Simplified fractions are easier to work with, especially when performing arithmetic operations like addition, subtraction, multiplication, and division.
• Avoiding confusion: Equivalent fractions can lead to confusion if not simplified. For instance, 2/4 and 1/2 are equivalent, but 1/2 is the simpler form.
• Enhancing understanding: Simplifying fractions helps in understanding the underlying math and relationships between numbers.

Simplifying 45/1

Now that we understand the importance of simplifying fractions, let's simplify 45/1. As mentioned earlier, 45/1 is already in its simplest form because the denominator is 1. When the denominator is 1, the fraction is essentially an integer, and no further simplification is needed.

However, if we were to simplify a fraction with a denominator other than 1, we would follow these steps:

1. Find the greatest common divisor (GCD) of the numerator and the denominator.
2. Divide both the numerator and the denominator by the GCD.

For example, if we wanted to simplify the fraction 45/15, we would:

1. Find the GCD of 45 and 15, which is 15.
2. Divide both the numerator and the denominator by 15, resulting in 3/1.

Practical Applications of Simplifying Fractions

Simplifying fractions has numerous practical applications in various fields, including:

• Cooking and recipe scaling
• Measuring ingredients and quantities
• Financial calculations and accounting
• Science and engineering applications

In conclusion, simplifying fractions is an essential skill in mathematics that helps in reducing complexity, avoiding confusion, and enhancing understanding. By mastering the art of simplifying fractions, you'll become more proficient in mathematical operations and problem-solving.

Real-World Examples of Simplifying Fractions

Let's explore some real-world examples of simplifying fractions:

• Recipe scaling: A recipe calls for 3/4 cup of flour, but you want to make half the recipe. You can simplify the fraction by dividing both the numerator and the denominator by 2, resulting in 3/8 cup.
• Measuring ingredients: A recipe requires 2 1/4 cups of sugar, but you only have a 1/4 cup measuring cup. You can simplify the fraction by converting it to an equivalent fraction with a denominator of 4, resulting in 9/4 cups.

Common Mistakes to Avoid When Simplifying Fractions

When simplifying fractions, it's essential to avoid common mistakes, such as:

• Dividing the numerator and denominator by different numbers
• Failing to find the greatest common divisor (GCD)
• Not checking for equivalent fractions

By avoiding these mistakes, you'll ensure that your simplified fractions are accurate and reliable.

Conclusion

In conclusion, simplifying fractions is a crucial skill in mathematics that helps in reducing complexity, avoiding confusion, and enhancing understanding. By mastering the art of simplifying fractions, you'll become more proficient in mathematical operations and problem-solving.

What's Next?

Now that you've learned about simplifying fractions, it's time to practice and apply your knowledge. Try simplifying different fractions and explore real-world examples of fraction simplification.