Mastering 2011 Ap Calc Bc Frq Form B

Quick Links :

The 2011 AP Calc BC FRQ Form B is a challenging exam that requires a deep understanding of calculus concepts, including limits, derivatives, integrals, and parametric and polar functions. In this article, we will break down each question of the exam and provide detailed explanations and solutions to help you master the material.

Understanding the Exam Format

Before we dive into the questions, let's take a brief look at the exam format. The 2011 AP Calc BC FRQ Form B consists of two sections: Section I, which contains 45 multiple-choice questions, and Section II, which contains 6 free-response questions. The free-response questions are divided into two parts: Part A, which consists of 2 questions, and Part B, which consists of 4 questions.

Section II, Part A

Question 1

Parametric and Polar Functions

A particle moves along a curve defined by the parametric equations x(t) = 2cos(t) and y(t) = 3sin(t). Find the area of the region bounded by the curve and the x-axis.

Solution

To find the area of the region bounded by the curve and the x-axis, we need to evaluate the definite integral of the y-coordinate with respect to x. Since the curve is defined parametrically, we can use the formula for the area of a parametric curve:

A = ∫[a, b] y(t)x'(t)dt

where x'(t) is the derivative of x(t) with respect to t.

First, we need to find the derivative of x(t):

x'(t) = -2sin(t)

Next, we can substitute x'(t) and y(t) into the formula for the area:

A = ∫[0, 2π] (3sin(t))(-2sin(t))dt

= -6∫[0, 2π] sin^2(t)dt

To evaluate this integral, we can use the trigonometric identity sin^2(t) = (1 - cos(2t))/2:

A = -6∫[0, 2π] (1 - cos(2t))/2 dt

= -3∫[0, 2π] (1 - cos(2t))dt

= -3[t - (1/2)sin(2t)] from 0 to 2π

= -3(2π - 0)

= -6π

Therefore, the area of the region bounded by the curve and the x-axis is -6π.

Question 2

Derivatives and Applications

A function f(x) is defined as f(x) = x^3 - 6x^2 + 9x - 2. Find the derivative of f(x) and use it to find the equation of the tangent line to the graph of f(x) at the point (2, 4).

Solution

To find the derivative of f(x), we can use the power rule and the sum rule:

f'(x) = d/dx (x^3 - 6x^2 + 9x - 2)

= d/dx (x^3) - d/dx (6x^2) + d/dx (9x) - d/dx (2)

= 3x^2 - 12x + 9

Next, we can use the derivative to find the equation of the tangent line to the graph of f(x) at the point (2, 4). The slope of the tangent line is given by the derivative evaluated at x = 2:

f'(2) = 3(2)^2 - 12(2) + 9

= 12 - 24 + 9

= -3

The equation of the tangent line is therefore:

y - 4 = -3(x - 2)

y = -3x + 10

Section II, Part B

Question 3

Integrals and Applications

A function f(x) is defined as f(x) = x^2 - 4x + 3. Evaluate the definite integral of f(x) from x = 0 to x = 2.

Solution

To evaluate the definite integral, we can use the power rule and the sum rule:

∫[0, 2] (x^2 - 4x + 3)dx

= ∫[0, 2] x^2 dx - ∫[0, 2] 4x dx + ∫[0, 2] 3 dx

= (1/3)x^3 - 2x^2 + 3x from 0 to 2

= (1/3)(2)^3 - 2(2)^2 + 3(2) - (1/3)(0)^3 + 2(0)^2 - 3(0)

= 8/3 - 8 + 6

= 2/3

Therefore, the value of the definite integral is 2/3.

Question 4

Sequences and Series

A sequence is defined recursively as a_n = 2a_(n-1) + 1, where a_1 = 1. Find the first five terms of the sequence.

Solution

To find the first five terms of the sequence, we can start with the initial term a_1 = 1 and use the recursive formula to generate the subsequent terms:

a_2 = 2a_1 + 1 = 2(1) + 1 = 3

a_3 = 2a_2 + 1 = 2(3) + 1 = 7

a_4 = 2a_3 + 1 = 2(7) + 1 = 15

a_5 = 2a_4 + 1 = 2(15) + 1 = 31

Therefore, the first five terms of the sequence are 1, 3, 7, 15, and 31.

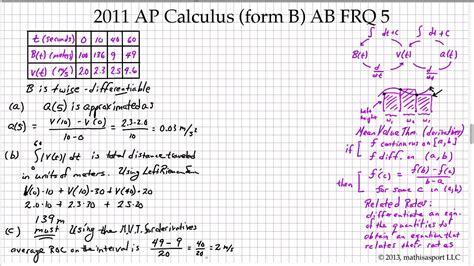
Question 5

Differential Equations

A differential equation is defined as dy/dx = 2x. Find the general solution to the differential equation.

Solution

To find the general solution to the differential equation, we can integrate both sides with respect to x:

∫dy = ∫2x dx

y = x^2 + C

where C is the constant of integration.

Therefore, the general solution to the differential equation is y = x^2 + C.

Question 6

Applications of Calculus

A company produces a product at a cost of $10 per unit and sells it for $20 per unit. The company's profit function is defined as P(x) = 10x - 2x^2, where x is the number of units produced. Find the maximum profit and the number of units that must be produced to achieve it.

Solution

To find the maximum profit, we can take the derivative of the profit function and set it equal to zero:

P'(x) = 10 - 4x = 0

x = 10/4 = 2.5

To verify that this value of x corresponds to a maximum, we can take the second derivative:

P''(x) = -4 < 0

Therefore, the maximum profit occurs when x = 2.5. To find the maximum profit, we can substitute this value of x into the profit function:

P(2.5) = 10(2.5) - 2(2.5)^2

= 25 - 12.5

= 12.5

Therefore, the maximum profit is $12.50, and the company must produce 2.5 units to achieve it.

Conclusion

In this article, we have worked through each question of the 2011 AP Calc BC FRQ Form B exam, providing detailed explanations and solutions to help you master the material. We hope that this article has been helpful in your preparation for the AP Calculus BC exam.

FAQs