Simplifying Radicals Made Easy: 180 Degrees In Simplest Form

Quick Links :

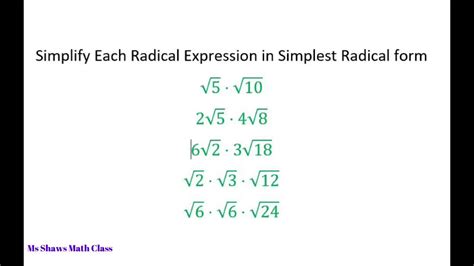
Unlocking the Secrets of Simplifying Radicals

Radicals, also known as roots, are a fundamental concept in mathematics, particularly in algebra and geometry. However, simplifying radicals can be a daunting task, especially for students who are new to the subject. In this article, we will delve into the world of radicals and provide a comprehensive guide on how to simplify them with ease.

Understanding Radicals

Before we dive into the simplification process, it's essential to understand what radicals are and how they work. A radical is a mathematical expression that represents a number that, when multiplied by itself, gives a specified value. For example, the square root of 16 is 4, because 4 multiplied by 4 equals 16.

Radicals are denoted by the radical symbol, which looks like a checkmark (√). The number inside the radical symbol is called the radicand. In the example above, 16 is the radicand.

Why Simplify Radicals?

Simplifying radicals is crucial in mathematics because it helps to:

• Reduce complex expressions to simpler forms
• Facilitate calculations and problem-solving
• Enhance understanding of mathematical concepts
• Improve communication and collaboration among mathematicians and scientists

The Basics of Simplifying Radicals

Simplifying radicals involves finding the simplest form of a radical expression. This can be achieved by factoring the radicand, using properties of radicals, and applying simplification rules.

Here are some basic steps to simplify radicals:

1. Factor the radicand: Break down the radicand into its prime factors.
2. Use properties of radicals: Apply the properties of radicals, such as the product rule and the quotient rule.
3. Simplify the expression: Combine the factors and simplify the expression.

Properties of Radicals

Understanding the properties of radicals is essential for simplifying radical expressions. Here are some key properties:

• Product rule: √(ab) = √a × √b
• Quotient rule: √(a/b) = √a ÷ √b
• Power rule: √(a^n) = a^(n/2)

These properties can be used to simplify radical expressions by factoring the radicand and applying the rules.

Simplifying Radical Expressions

Now that we have covered the basics and properties of radicals, let's move on to simplifying radical expressions.

Here are some examples:

• √(16) = √(4 × 4) = √4 × √4 = 2 × 2 = 4
• √(24) = √(4 × 6) = √4 × √6 = 2 × √6
• √(48) = √(16 × 3) = √16 × √3 = 4 × √3

Common Mistakes to Avoid

When simplifying radicals, there are some common mistakes to avoid:

• Not factoring the radicand: Failing to factor the radicand can lead to incorrect simplification.
• Not applying properties of radicals: Failing to apply the properties of radicals can lead to incorrect simplification.
• Not simplifying the expression: Failing to simplify the expression can lead to a complex and unnecessary radical expression.

Real-World Applications of Simplifying Radicals

Simplifying radicals has numerous real-world applications, including:

• Physics and engineering: Simplifying radicals is crucial in physics and engineering, where complex calculations are involved.
• Computer science: Simplifying radicals is used in computer science, particularly in algorithms and data structures.
• Mathematics: Simplifying radicals is a fundamental concept in mathematics, particularly in algebra and geometry.

Conclusion: Simplifying Radicals Made Easy

Simplifying radicals may seem daunting at first, but with practice and patience, it can become second nature. By understanding the basics, properties, and applications of radicals, you can simplify radical expressions with ease.

We hope this article has provided you with a comprehensive guide on simplifying radicals. Remember to practice regularly and avoid common mistakes.

What's your experience with simplifying radicals? Share your thoughts and tips in the comments below!

Want to learn more about mathematics and science? Check out our other articles and resources!