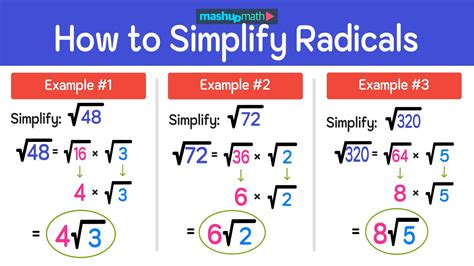
Simplifying radicals can seem like a daunting task, but with the right approach, it can be made easy. Whether you're a student or a professional, understanding how to simplify radicals is essential for problem-solving in mathematics. In this article, we'll break down the process into 18 easy steps, covering the basics, rules, and examples to help you master radical simplification.
Radicals are an essential part of mathematics, and simplifying them is a crucial skill. Radicals represent the nth root of a number, and they can be simplified by reducing the number under the radical sign to its most basic form. This process involves breaking down the number into its prime factors and simplifying the radical expression.

Understanding Radicals
Before we dive into the steps, it's essential to understand the basics of radicals. A radical is a symbol that represents the nth root of a number. The nth root of a number x is a value that, when raised to the power of n, gives x. For example, the square root of 16 is 4 because 4^2 = 16.
Radicals can be expressed in two forms: square roots and nth roots. Square roots are the most common type of radical and represent the second root of a number. Nth roots represent any root other than the square root.
Step 1-3: Identify the Type of Radical
To simplify a radical, you first need to identify the type of radical. Is it a square root or an nth root? This will determine the steps you take to simplify it.
- If the radical is a square root, proceed to step 4.
- If the radical is an nth root, proceed to step 5.
Step 4: Simplify Square Roots
To simplify a square root, look for perfect squares within the radical expression. A perfect square is a number that can be expressed as the product of an integer with itself. For example, 16 is a perfect square because it can be expressed as 4^2.
- Identify any perfect squares within the radical expression.
- Factor out the perfect squares.
- Simplify the radical expression.
Step 5-6: Simplify Nth Roots
To simplify an nth root, look for perfect nth powers within the radical expression. A perfect nth power is a number that can be expressed as the product of an integer with itself n times. For example, 27 is a perfect cube because it can be expressed as 3^3.
- Identify any perfect nth powers within the radical expression.
- Factor out the perfect nth powers.
- Simplify the radical expression.
Step 7-9: Break Down the Number into Prime Factors
Breaking down the number into prime factors is essential for simplifying radicals. Prime factors are the building blocks of numbers, and they can help you identify perfect squares and nth powers.
- Factor the number into its prime factors.
- Look for pairs of identical prime factors.
- Simplify the radical expression.
Step 10-12: Simplify Radical Expressions with Variables
Radical expressions with variables require special attention. To simplify them, you need to identify any perfect squares or nth powers within the expression.
- Identify any perfect squares or nth powers within the expression.
- Factor out the perfect squares or nth powers.
- Simplify the radical expression.
Step 13-15: Simplify Radical Expressions with Coefficients
Radical expressions with coefficients can be simplified by factoring out the coefficient.
- Factor out the coefficient.
- Simplify the radical expression.
Step 16-18: Simplify Complex Radical Expressions
Complex radical expressions involve multiple radicals or variables. To simplify them, you need to identify any perfect squares or nth powers within the expression.
- Identify any perfect squares or nth powers within the expression.
- Factor out the perfect squares or nth powers.
- Simplify the radical expression.

Rules for Simplifying Radicals
- The product rule: a√b × c√d = ac√(bd)
- The quotient rule: a√b ÷ c√d = (a/c)√(b/d)
- The power rule: (a√b)^n = a^n√(b^n)

Examples of Simplifying Radicals
- Simplify: √16 = √(4^2) = 4
- Simplify: ³√27 = ³√(3^3) = 3
- Simplify: √(x^2 + 4) = √((x + 2)(x - 2)) = x + 2

Conclusion
Simplifying radicals may seem like a daunting task, but with the right approach, it can be made easy. By following the 18 easy steps outlined in this article, you can master radical simplification and improve your problem-solving skills in mathematics. Remember to identify the type of radical, break down the number into prime factors, and simplify the radical expression. With practice and patience, you'll become proficient in simplifying radicals and tackle even the most complex mathematical problems with confidence.

Now it's your turn! Practice simplifying radicals using the steps outlined in this article. Share your experiences, ask questions, or provide feedback in the comments section below.